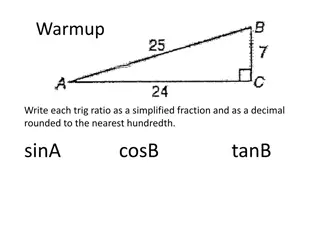
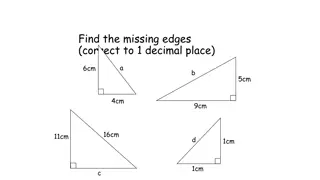
Understanding the Ambiguous Case in Trigonometry
Trigonometry's ambiguous case occurs when a triangle can have two possible solutions due to given side lengths and angles. This concept is pivotal in solving triangles accurately, avoiding confusion with multiple solutions. Explore scenarios, calculations, and identification methods for the ambiguous case in trigonometry.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
CHAPTER 2 - TRIGONOMETRY Section 2.3B The Ambiguous Case of the Sine LAW
WHAT IS THE AMBIGUOUS CASE? C C C C 16 16 10 10 30 30 A B B A A A B B A 2nd triangle that meet these specs can be made by making an isosceles triangle inside the 1st one An ambiguous case will occur when we have two different triangles that can meet the given requirements
So when solving a triangle with an ambiguous case, we need to consider both triangles case: EX: IN ABC, A = 10, B = 16, AND SOLVE FOR ANGLE B. C = 30 A C C C 16 16 10 10 10 30 30 B A B A A A B B sin30 10 16 sin30 10 sin 16 B = 53.13 53.13 = 126.17 B The triangle inside must be isosceles = sin B = 1 16 sin30 10 sin B = = 180 53.13 126.87 B B = 53.13 B
EX: SOLVE ABC, GIVEN THE FOLLOWING: 48 , 9, and B b = = A Solving a triangle means finding the length and degree of all the lines (BC) and angles ( A and C ) = 11 c 11 9 Note: There are two possible cases!! 180 A = 66.73 A = Use the sine law to find the length of BC sin66.73 BC ( ) 480 + 48 65.27 B C = sin48 9 sin 11 C 11sin48 9 1 11sin48 sin 65.27 sin65.27 11 11sin66.73 sin65.27 11.12 = sin C = = BC = C 9 C = BC =
Triangle #2 of the Ambiguous case: From the previous triangle, angle C was equal to 65.27 , and now we need to find C2 180 C = 114.73 C = A 65.27 11 2 9 C 65.27 65.27 480 2 2 B C 180 17.27 Find A = A = A ( ) Use the sine law to find the length of BC sin17.27 BC + 48 114.73 sin65.27 11 11 sin17.27 sin65.27 3.60 = = BC Note: Side BC from this triangle needs to be shorter than the other one BC =
E Suppose you are given a crappy diagram where the missing side is not on the botom Note: Rotate the shape so the missing side will be on the bottom! sin50 sin 15 17 17 sin50 sin 15 0.86818 sinE = 2 F F sin50 15 15 sin10.25 sin50 15 50 F D 17 = E Ambiguous case! 180 60.25 E = 119.75 E = F 2 = 17 E 15 2 = = 180 50 119.75 10.25 sin F f 50 E D 1 E 2 = sin 0.86818 60.25 69.75 E E F = sin50 15 sin F = = = f = = f 3.484 15 sin69.75 sin50 = = 18.37 f
HOW TO TELL IF A TRIANGLE IS AMBIGUOUS First thing you do is place the two given sides on top and the missing side on the bottom If the side opposite from the given angle is smaller, then the triangle will be ambiguous C C C C C C 14 15 12 13 12 15 34 34 B A A A B B B A A A B B The side opposite the angle is smaller, so it can be ambiguous, b/c an isos triangle can be made The side opposite the angle is bigger, so it can NOT be ambiguous, b/c an isos triangle can NOT be made
PRACTICE: ARE THESE TRIANGLES AMBIGUOUS? 15 24 21 24 12 50 18 24 27 33 No, b/c 2 angles are given Yes b/c side opposite of angle is smaller No b/c side opp. Of angle is bigger If you can t tell, rotate the triangle so the missing side is on the bottom 8 20 3 27 16.7 12 57 12 Technically, this one should be yes, HOWEVER, the opposite is too small The height is 16.7, can t have an hypotenuse smaller than the height, so NO 8 27 Yes b/c side opposite of angle is smaller
= 30 , = = Practice: Solve ABC given, 15, and 5 A a b C Even though the side opposite is shorter, the triangle may not be possible b/c it is too short 15 5 300 B A 0 sin 15 sin30 5 15sin30 B= Sin can only be between -1 and 1. It can not be equal to 1.5. So this triangle is not possible 0 B = sin sin Note: Don t bother with finding the height, just use the sine law right away 5 1.5 B =
N 0 0 0 X 0 0 y y 37.5km 22km 22km 25 ??km x x 0 0 0 0 0 0 X 0 0 X 0 0 ??km Port Now, suppose there was a giant fog, we may have an ambiguous case 0 A cruise ship is 37.5 km from a port and 22 km from an oil tanker. The angle created by the cruise and oil tanker is 25degrees at the sin 37.5 lighthouse. How far is the oil tanker from the lighthouse? sin25 22 37.5sin25 22 1 15.8482 sin 46.1 x= Angle x is obtuse 0 sin108.9 ?? sin21.1 sin25 22 sin25 = = 0 0 0 22sin108.9 sin25 22sin22.1 sin25 x = sin = ?? ?? 22 0 = km = = x ?? 49.2 ?? 22 y = 2 x = y = 108.9 133.9 21.1 x = km = 18.7 ??