Trigonometry Concepts: Graphs, Symmetry, and Laws Explained
Explore trigonometry concepts such as sine, cosine, and tangent graphs, symmetry in trigonometric functions, and laws of trigonometric functions. Understand how to determine values using symmetry and graph analysis. Practice identifying values in the range 0 to 360 for sine, cosine, and tangent functions that are equivalent.
Uploaded on Sep 28, 2024 | 0 Views
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
C1: Chapters 8 & 10 Trigonometry Dr J Frost (jfrost@tiffin.kingston.sch.uk) www.drfrostmaths.com Last modified: 1st September 2015
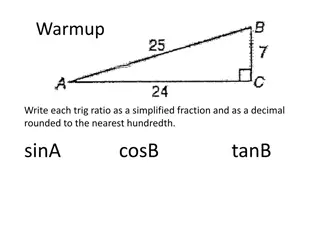
Sin Graph What does it look like? -360 -270 -180 -90 ? 90 180 270 360
Sin Graph What do the following graphs look like? -360 -270 -180 -90 90 180 270 360 Suppose we know that sin(30) = 0.5. By thinking about symmetry in the graph, how could we work out: ? ? ? sin(150) = 0.5 sin(-30) = -0.5 sin(210) = -0.5
Cos Graph What do the following graphs look like? -360 -270 -180 -90 ? 90 180 270 360
Cos Graph What does it look like? -360 -270 -180 -90 90 180 270 360 Suppose we know that cos(60) = 0.5. By thinking about symmetry in the graph, how could we work out: ? ? ? cos(120) = -0.5 cos(-60) = 0.5 cos(240) = -0.5
Tan Graph What does it look like? -360 -270 -180 -90 ? 90 180 270 360
Tan Graph What does it look like? -360 -270 -180 -90 90 180 270 360 Suppose we know that tan(30) = 1/ 3. By thinking about symmetry in the graph, how could we work out: ? ? tan(-30) = -1/ 3 tan(150) = -1/ 3
Laws of Trigonometric Functions We saw for example sin(30) = sin(150) and cos(30) = cos(330). It s also easy to see by looking at the graphs that cos(40) = sin(50). What laws does this give us? sin(x) = sin(180 x) ? cos(x) = cos(360 x) ? sin and cos repeat every 360 ? tan repeats every 180 ? sin(x) = cos(90 x) ? Bro Tip: These 5 things are pretty much the only thing you need to learn from this Chapter!
Practice Find all the values in the range 0 to 360 for which sin/cos/tan will be the same. sin(30) = sin(150) cos(70) = cos(290) ? ? 7 1 cos(30) = cos(330) cos(-25) = cos(25) = cos(335) ? ? ? 2 8 sin(-10) = sin(190) = sin(350) cos(80) = sin(10) 3 ? ? ? 9 ? cos(-40) = cos(40) = cos (320) sin(15) = sin(165) 4 ? 10 sin(20) = cos(70) sin(-60) = sin(240) = sin(300) 5 ? ? ? 11 tan(80) = sin(260) ? 12 sin(80) = sin(100) ? 6
Dr Frosts technique for remembering trig values (once described by a KGS tutee of mine as the Holy Grail of teaching ) I literally picture this table in my head when I m trying to remember my values. All the values in this square are over 2. The diagonals starting from the top left are rational. The other values in the square are not. 0 45 90 30 60 3 2 sin 0 _1_ 2 1 _1_ 2 3 2 cos 1 _1_ 2 0 _1_ 2 3 tan 0 1 _1_ 3 All the surds in this block are 2 All the surds in this block are 3 I remember that out of tan(30) and tan(60), one is 1/ 3 and the other 3. However, by considering the graph of tan, clearly tan(30) < tan(60), so tan(30) must be the smaller one, 1/ 3
Practice 0 45 90 30 60 3 2 ? sin 0 ? _1_ 2 ? 1 ? _1_ 2 ? 3 2 ? cos 1 ? _1_ 2 ? 0 ? _1_ 2 ? 3 ? tan 0 ? 1 ? _1_ 3 ?
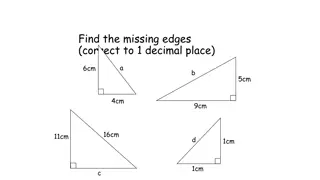
Magic Triangles You can easily work out sin(45), cos(45), sin(30), tan(30) etc. if you were ever to forget. 30 2 2 ? 3 ? 1 60 45 1 1 ? cos(30) = _ 3_ sin(45) = _1_ ? ? 2 2
Angle quadrants Given that sin = 2/5, and that is obtuse, find (without a calculator) the exact value of cos . 21 5 ? cos? = 5 ? Therefore thinking about the graph of cos: 2 ? 21 5 ? cos? = 21 ? Since by thinking about the graph of cos, we can see that cos 180 ? = cos ? Imagine working instead with the acute angle such that sin = 2/5
Angle quadrants Given that tan = 5/12, and that is acute, find the exact value of sin and cos . 1 sin = 5/13, cos = 12/13 ? ? Given that cos = -3/5, and that is obtuse, find the exact value of sin and tan . 2 sin = 4/5, tan = -4/3 ? ? Given that tan = - 3, and that is reflex, find the exact value of sin and tan . 3 Hint: if tan is negative, then is our reflex angle between 180 and 270, or 270 and 360? ? sin = - 3/2, cos = 1/2 ?
The only 2 identities you need this chapter... r y = r sin ? x = r cos ? sin cos = tan sin = y/r and cos = x/r and tan = y/x 1 ? Pythagoras gives you... 2 sin2 + cos2 = 1 ?
Examples of use Simplify sin2 3 + cos2 3 = 1 1 2 Show that: ? cos4? sin4? cos2? 1 tan2? Simplify 5 5sin2 = 5cos2 ? Given that p = 3 cos and q = 2 sin , show that 4p2 + 9q2 = 36. 3 This box is intentionally left blank.
Solving Trigonometric Equations Edexcel May 2013 () = 123.44, 176.57 ? Bro Tips for solving: 1. If 0 < 180, then what range does 2 30 have? 2. Immediately after the point at which you do sin-1 of both sides, list out the other possible angles in the above adjusted range. Recall that sin(x) = sin(180-x) and that sin repeats every 360.
Solving Trigonometric Equations Edexcel June 2010 tan = 0.4 ? a b 0 2x < 720 ? tan 2x = 0.4 2x = 21.801, 201.801, 381.801, 561.801, x = 10.9, 100.9, 190.9, 280.9
Solving Trigonometric Equations Edexcel Jan 2010 (2sin x 1)(sin x + 3) = 0 sin x = 0.5 or sin x = -3 x = 30 , 150 ? Bro Tip: In general, when you have sin and cos, and one is squared, change the squared term to be consistent with the other.
Exercises Edexcel Jan 2009 Edexcel Jun 2009 ? = 45 ,135 ,23.6 ,156.4 ? = 41.4 ,318.6 ? ? 284.5, 435.5, 644.5 ? Edexcel Jun 2008 Edexcel Jan 2008 ? 65, 155 40 80 160 200 280 320 ? Edexcel Jan 2013 = 230.785, 309.23152, 50.8, 129.2 ? 41.2, 85.5, 161.2 ?
Things to remember If you square root both sides, don t forget the . You ll probably lose 2 marks otherwise. 1 sin23? =1 1 2 sin3? = 2 2 Don t forget solutions. If you have sin, you ll always be able to get an extra solution by using 180 x. If you have cos you can get an extra one using 360-x. Remember that tan repeats every 180, sin/cos every 360. 3 If you had sin2x and cos x, you d replace the sin2 x with 1 cos2 x. You d then have a quadratic in terms of cos x which you can factorise. 4 5 Check whether the question expects you to give your answers in degrees or radians. If they say 0 ? ?, then clearly they want radians.