Trigonometry and Vector Illustrations in Physics
Explore applications of trigonometry and vectors in solving real-world problems such as directing a helicopter pilot to a stranded camper, measuring the height of a tree, analyzing displacement vectors, and adding vectors using graphical and component methods.
Download Presentation
Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
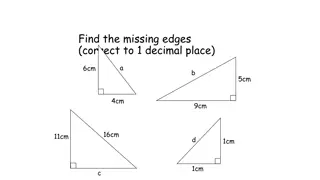
Trigonometry Right-Triangle Pythagorean Theorem sin cos tan Starting from the base, a camper do the following walks and gets lost. First 3 miles north, then 4 miles east, and then 6 miles south. How will you direct a helicopter pilot to reach the stranded camper? A park ranger wanted to measure the height of a tall tree. The ranger stood 9.50 m from the base of the tree; and he observed that his line of sight made an angle of 65.2 above the horizontal as he looked at the top of the tree. The park ranger's eyes are 1.80 m above the ground. What is the height of the tree?
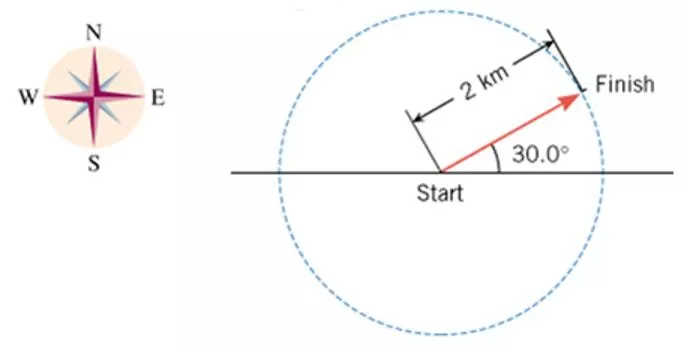
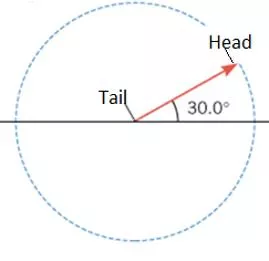
Vector Illustration Consider the following displacement vector: 2 km @ 300 North of East. The length of the vector arrow is proportional to the magnitude of the vector and the arrow represents the direction. In the text, bold face is used for vectors and italics is used for scalars. When hand written an arrow is placed above the symbol. A vector can be moved, keeping its magnitude and direction. Head and Tail of a vector are:
Components of a Vector Consider the following vector, A: How can we replace vector A by two perpendicular components?
Components of a Vector Aadj=A Cos and Aopp=A Sin
Chapter 3, Problem 15 15. Find the north and east components of the displacement from San Francisco to Sacramento shown in Figure 3.59.
Adding Vectors:Head-to-Tail Method Example 3.1: A Woman Takes a Walk (Page 91, Text) Use the graphical technique for adding vectors to find the total displacement of a person who walks the following three paths (displacements) on a flat field. First, she walks 25.0 m in a direction 49.0 north of east. Then, she walks 23.0 m heading 15.0 north of east. Finally, she turns and walks 32.0 m in a direction 68.0 south of east.
Vector Addition and Subtraction B + A B A A B A B
Addition of Vectors using Vector Components A jogger runs 145 m in a direction 20.0 east of north (displacement vector A) and then 105 m in a direction 35.0 south of east (displacement vector B). Using components, determine the magnitude and direction of the resultant vector C.
Analytical/Component Method Find the resultant and equilibrant of the three vectors in the drawing by means of the component method. The magnitudes of the vectors are A = 250-g, B = 300-g, and C = 260-g.
