Understanding Angles and Measurements in Trigonometry
Exploring the conversion of angles to decimals and degrees, as well as the relationships between radians and degrees in trigonometry. Discover how to calculate arc lengths in circles and the significance of angles in radians.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
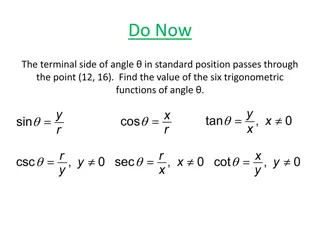
Section 6.1 Angles and Their Measure
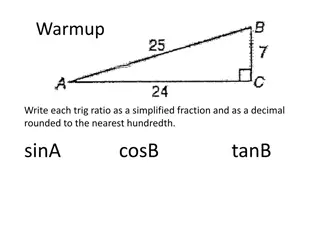
(a) Convert 40125 to a decimal in degrees. Round the answer to four decimal places. (b) Convert 78.562 to the D M S form. Round the answer to the nearest second. 1 1 and 1 = 60 1 60 60 1 = 1 60 1 60 60 1 (a) 40 + 12 + 5 = 40 + 12 + 5 = 40 + 0.2 + 0.0014 40.2014 = ( )( + ) 0.562 60 ) 78 = + = ( 0.72 60 + )( 33.72 = (b) 78 0.562 + 78 = + + = + + 78 78 33 0.72 = + 78 33 43 78 33 33 43.2
Find the length of the arc of a circle of radius 4 meters subtended by a central angle of 0.5 radian. ( )( 4 0.5 ) s = = 2 meters
(a) 30 (b) 120 (c) 60 (d) 270 (e) 104 radian 30 radians 180 6 = = radian 180 2 0 0 (a) (b) 12 0 radians 3 = radian 180 3 = radian 180 0 0 (d) 27 0 radians (c) 6 0 radians 2 3 radian 180 0 (e) 104 1.815 radians
5 (a) radian (b) radian (c) radians (d) 5 radians 3 2 6 = 180 180 = (a) 60 (b) 90 3 2 = 180 = 5 180 (d) 5 286.48 (c) 150 6
= must be in radians. r In order to use s Figure 13 (a) Figure 13 (b)
Find the area of the sector of a circle of radius 5 feet formed by an angle of 60 . Round the answer to two decimal places. In order to use the equation for the area of a sector, must be in radians. = = 60 180 3 1 2 25 ( ) 5 2 = = 13.09 square feet A 3 6
Linear Speed Angular Speed
A child is spinning a rock at the end of a 3-foot rope at the rate of 160 revolutions per minute (rpm). Find the linear speed of the rock when it is released. 3 160 revolutions 1 minute 2 radians 1 revolution radians minute = = 320 v = r = 3 feet 320 radians feet minute minute 3016 feet minute 1 mile 5280 feet 60 minutes v 3016 34.3 mph 1 hour