Trigonometry Basics for Robotics: Understanding Triangles and Interior Angle Addition
Explore the fundamentals of trigonometry in robotics through an in-depth look at triangles, their components, and the interior angle addition theorem. Learn about vertices, sides, angles, and the centroid of a triangle, as well as special right triangles. Enhance your understanding with detailed examples and illustrations.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
Math Basics for Robotics Trigonometry Long Wang 1
Triangles Q1: What does a triangle have? 2
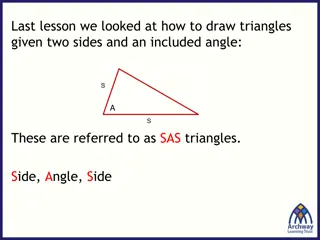
Triangles ? Q1: What does a triangle have? ?1 ? 3 vertices: ?,?,? 3 sides: ?1,?2,?3 3 angles (interior): ?,?,? ? ? ?2 ?3 ? ? 3
Triangles ? Q1: What does a triangle have? ?3 ? 3 vertices: ?,?,? 3 sides: ?1,?2,?3 3 angles (interior): ?,?,? ? ? ?1 ?2 ? ???????? ? 4
Interior Angle Addition ? ?1 Fact #1 to remember: ? The sum of the measures of the interior angles of a triangle is always 180 degrees ? ? ?2 ?3 ? ? + ? + ? = 180 ? 5
Centers of a Triangle - Centroid Def. #1 to remember: What is a centroid? Think it as center of mass, geometric center, or average position of all the vertices. ? ?1 ? The centroid of a triangle - the intersection of the three medians. ? ? ?2 ?3 ? ? 6
Centers of a Triangle - Centroid Def. #1 to remember: What is a centroid? Think it as center of mass, geometric center, or average position of all the vertices. ? ?1 ? The centroid of a triangle - the intersection of the three medians. ? ? ?2 ?3 ? ? 7
Example 1 1) Pick arbitrary 3 vertices; 2) Connect them with 3 edges; 3) Label vertices, edges and angles; 4) Measure all of them. 5) Find the centroid of your triangle ? ?1 ? ? ? ?2 ?3 ? ? 8
Special Triangle Right Triangle ? A right triangle is a triangle in which one angle is a right angle (that is, a 90-degree angle). ?3 ? ?1 ? ? ? ?2 Hypotenuse - the side opposite to the right angle. Legs - the sides adjacent to the right angle. Adjacent/opposite leg need to pick an angle first. 9
Special Triangle Right Triangle ? A right triangle is a triangle in which one angle is a right angle (that is, a 90-degree angle). ?3 ? ?1 ? ? ? ?2 Hypotenuse - ?3 Legs - ?1,?2 For angle ?, the adjacent leg is ?2, and the opposite leg is ?1. 10
Special Triangle Right Triangle ? Fact #2 to remember: ?3 ? ?1 ? and ? are complementary. ? Complementary angles are angle pairs whose measures sum to one right angle ? + ? = 90 ? ? ?2 11
Special Triangle Right Triangle ? Fact #2 to remember: ?3 ? ?1 ? and ? are complementary. ? Complementary angles are angle pairs whose measures sum to one right angle ? + ? = 90 ? ? ?2 Quiz: what was the previous fact to remember? Can we use it to prove this fact? 12
Special Triangle Right Triangle ? Fact #3 to remember: ?3 ? ?1 The square of the hypotenuse is equal to the sum of the squares of the other two sides. ? ? ? ?2 Pythagorean Theorem 2+ ?2 2= ?3 2 ?1 13
Example 2 ? ?3 ? ?1 ? ? ? ?2 1) Use ruler/triangle/grid paper to draw a right triangle whose legs are: 3 and 4 5 and 12 8 and 15 2) Measure the length of hypotenuse 3) Are your findings consistent with Fact #3? 14
Cosine, Sine, Tangent ? ? ? sin? = cos? = tan? = ?/? ? ? Sine = Opposite / Hypotenuse Cosine = Adjacent / Hypotenuse Tangent = Opposite / Adjacent Reminder: sine, cosine, tangent are defined only in right triangles 15
Example 3 ? Given ?1= 1 ?2= 2 ?3 ? ?1 ? Solve cos?=? ?=? ? ? ?2 16
Example 3 ? Given ?1= 1 ?2= 2 ?3 ? ?1 ? ? ? ?2 Solve 2+ ?2 ?1?3= 1/ 5 2= ?3= cos? = ? = arctan(?1/?2) = arctan1 ?1 5 2= 26.6 17
Special Triangle - Equilateral Fact #4 to remember: Special properties of equilateral triangle All three sides have equal lengths Each angle = 60 Median = Altitude = Bisector 18
Special Triangle - Equilateral Fact #4 to remember: Special properties of equilateral triangle All three sides have equal lengths Each angle = 60 Median = Altitude = Bisector =30 , =60 19
Special Triangle - Equilateral Fact #4 to remember: Special properties of equilateral triangle All three sides have equal lengths Each angle = 60 Median = Altitude = Bisector =30 , =60 Example 4 In the equilateral triangle above, if the length of each side is 10 cm, what is the length of the red line segment. 20
Law of Cosine Law of Cosine ?2= ?2+ ?2 2??cos? How do we use this equation? 21
Law of Cosine Law of Cosine ?2= ?2+ ?2 2??cos? cos? =?2+ ?2 ?2 OR 2?? Law of Cosine tells us: 1) the third side can be solved if we know two sides and the angle between them 2) Each angle can be solved if we know all three sides 22
Example 5 ? = 2 Solve ? =? ? = 3 ? = 4 23
Example 5 ? = 2 Solve ? =? ? = 3 ? = 4 Solve cos? =?2+?2 ?2 ? = arccos11 =22+42 32 2 2 4 =11 2?? 16= 46.6 16 24
Coordinates system A coordinate system is a system which uses one or more numbers, or coordinates, to uniquely determine the position of a point. 25
Coordinates system (-1,3) A coordinate system is a system which uses one or more numbers, or coordinates, to uniquely determine the position of a point. (3,1) (3,-2) 26
Coordinates system Def. #2 to remember: (-1,3) The two axes divide the plane into four infinite regions, called quadrants. (3,1) (3,-2) 27
Coordinates system Def. #2 to remember: (-1,3) II (-,+) I The two axes divide the plane into four infinite regions, called quadrants. (+,+) (3,1) III (-,-) IV (+,-) 28
Coordinates system ? Q1: How to find the coordinates (numbers) of a point that is not on the grid? (?1,?1) ?1 ? ?1 ? 29
Coordinates system ? Q1: How to find the coordinates (numbers) of a point that is not on the grid? (?1,?1) Q2: How to find the distance from origin to point (?1,?1)of a point that is not on the grid? ?1 ? ?1 ? 30
Coordinates system ? Q1: How to find the coordinates (numbers) of a point that is not on the grid? (?2,?2) ?2 (?1,?1) Q2: How to find the distance from origin to point (?1,?2)of a point that is not on the grid? Q3: How to find the distance from point (?1,?2) to point (?2,?2)? ?1 ? ?1 ? ?2 31
Coordinates system ? (?2,?2) ?2 |?2 ?1| (?1,?1) ?1 |?2 ?1| ? ?1 ?2 ? Solutions ?1= ?12= 2+ ?1 ?2 ?1 2 ?1 2+ ?2 ?1 2 32
Example 6 ? Given the side length ?, find the coordinates of three vertices. The coordinate system origin is at the centroid, and the y axis is aligned with one median. ? 33
Example 6 ? (?2,?2) ?2= 0 ?2= ? ? ? 2 ?1= ?cos30 ?1= ?sin30 ? = (?1,?1) ? 2 (?3,?3) ?3= ?cos30 ?3= ?sin30 ? = ? 2cos30 ? 2cos30 34
Redefine cosine, sine, tangent unit circle Question: How do we define cosine, sine and tangent, if the angle is bigger than 90 , or even bigger than 180 ? 35
Redefine cosine, sine, tangent unit circle A unit circle is a circle with a radius of one. ? ? 36
Redefine cosine, sine, tangent unit circle A unit circle is a circle with a radius of one. ? = 45 cos? =? sin? =? (?,?) ? ? ? 37
Redefine cosine, sine, tangent unit circle A unit circle is a circle with a radius of one. ? = 135 cos? =? sin? =? (?,?) ? ? ? 38
Redefine cosine, sine, tangent unit circle A unit circle is a circle with a radius of one. ? = 225 cos? =? sin? =? (?,?) ? ? ? 39
Redefine cosine, sine, tangent unit circle A unit circle is a circle with a radius of one. ? = 315 cos? =? sin? =? (?,?) ? ? ? 40
Special lines of a Triangle Median - a line segment joining a vertex to the midpoint of the opposing side. ? ?1 ? ? ? Altitude - a line segment through a vertex and perpendicular to the opposing side. ?2 ?3 ? ? 41