Understanding Radian Measure in Trigonometry
Explore the concept of radian measure, converting between degrees and radians, calculating arc lengths, sectors, and segments, and understanding the importance of radians in trigonometry. Discover formulas and examples illustrating the application of radians in various mathematical calculations.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
Radian Measure
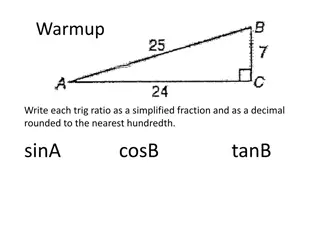
Trigonometry: Radians BAT convert fluently between degrees and radians BAT calculate arcs, sectors and segments KUS objectives Starter: Simplify (without a calculator) sin30 1 + 3 + 3cos60 2sin45 + cos45 1 + 2 tan150 1 + 3 3tan120
Introduction to Radian measure1 For a circle of radius r, the angle at the centre, which subtends an arc of length r, is equal to 1 radian If arc AB has length r, then angle AOB is 1 radian (1cor 1 rad) A r O 1c r r As the circumference of the circle is 2 r the total angle is 2 radians Radians are an alternative to degrees. Some calculations involving circles are easier when Radians are used, as opposed to degrees. B r 2 r 360 180 = 1c 45 135 225 0 30 60 90 120 150 180 210 = = = c c 2 2 c 0? ??
Converting radians and degrees Radians Degrees ??= 180 WB 1 Convert the following angles to degrees ? ? 4? 15 7? 8 a) b) c) 1.8? =7? 8 180 = 1.8 180 =4? 15 180 ? ? ? =1260? 8? =720? 15?= 48 = 103 = 157.5
Converting degrees to radians Degrees Radians ??= 180 WB 1 Convert the following angles to radians d) e) f) 110 350 150 ? ? ? = 110 = 35 = 150 180 180 180 ? =5? 11? 18 =7? 36= 0.611? = 6 ? is often unwritten The superscript Now do skills 249
SECTOR area The Area of a Sector and Segment can be worked out using Radians Area of Sector Total Area Angle at Centre Total Angle = X r A = 2 2 Multiply by O X r X = 2 2 Multiply by r2 2 r B = X 2 1 2r = 2 X This is the formula s usual form
WB 2a arc length Arc AB of a circle, with centre O and radius r, subtends an angle of radians at O. The Perimeter of sector AOB is 10 cm. Express r in terms of . Length AB = r ????????? = ?? + 2? 10 = ?(? + 2) 10 A ? + 2= ? r 10 r r = O ? + 2 r B
WB 3 arc length Calculator in Radians O opp hyp 1.2 2 0.6 Finding the length of an arc is easier when you use radians = sin x (H) x 2m x = sin The border of a garden pond consists of a straight edge AB of length 2.4m, and a curved part C, as shown in the diagram below. The curved part is an arc of a circle, centre O and radius 2m. Find the length of C. C x = sin A B 1.2m (O) Inverse sine = 0.6435 x rad Double for angle AOB = 2 1.287 x rad Angle = 2 1.287 Angle = 4.996 rad = r 2 4.996 9.99 m l l = l = 2m 2m O B A 2.4m (We need to work out angle )
WB 4 sector area In the diagram, the area of the minor sector AOB is 28.9cm2. Given that angle AOB is 0.8 rad, calculate the value of r. 1 2 = r 2 A Put the numbers in 1 2r = 2 28.9 (0.8) x 0.8 = 0.4 = 2 28.9 0.4r A Divide by 0.4 r cm = 2 72.25 r 0.8c O Square root B = 8.5cm r
WB 5 sector area A plot of land is in the shape of a sector of a circle of radius 55m. The length of fencing that is needed to enclose the land is 176m. Calculate the area of the plot of land. The length of the arc must be 66m (adds up to 176 total) = 66 55 = 1.2c = r l Put the numbers in Divide by 55 A 55m 1 2 = r 2 A 66m 1.2c O Put the numbers in 1 55 2 55m = 2 A B (We need to work out the angle first) A= 2 1815 m Now do skills 251
Segment area Work out the area of a segment using radians. Area of a Segment Area of Sector AOB Area of Triangle AOB Area of Sector AOB 1 2 Area of Triangle AOB 1 2 a = b = r C = = r 2 A O = sin A ab C r r 1r sin 2 = 2 A A B Area of the Segment 1r 2 1r 2 1r sin 2 2 2 Factorise 2 ( sin ) It is not necessary too memorise this formula use common sense
WB 6 segment area Calculate the Area of the segment shown in the diagram below. 1r 2 2 ( sin ) Substitute the numbers in 12.5 2 2 sin 3 3 Work the parts out ( ) 0.1811... 3.125 Only round the final answer O 2.5cm 2 0.57 cm 3
WB 7 segment area In the diagram AB is the diameter of a circle of radius r cm, and angle BOC = radians. Given that the Area of triangle AOC is 3 times that of the shaded segment, show that 3 4sin = 0 Area of the shaded segment ( 2r 1 ) 2 sin Area of triangle AOC 1 2ab sin C a = b = r Angle = - 1 2r 2 sin( ) Remember, sin x = sin (180 x) C 1 2r 2 sin AOC = 3 x shaded segment Cancel out 1/2r2 Multiply out the brackets 1 2r 1 2r ( ) = 3 2 2 sin sin A B 0 ( ) sin = 3 sin = sin 3 3sin Subtract sin = 3 4sin 0
WB 8 Figure 2 shows a plan of a patio. The patio PQRS is in the shape of a sector of a circle with centre Q and radius 6 m. Given that the length of the straight line PR is 6 3 m, (a) find the exact size of angle PQR in radians. (b) Show that the area of the patio PQRS is 12 m2. (c) Find the exact area of the triangle PQR. (d) Find, in m2 to 1 decimal place, the area of the segment PRS. (e) Find, in m to 1 decimal place, the perimeter of the patio PQRS.
KUS objectives BAT convert fluently between degrees and radians BAT calculate arcs, sectors and segments self-assess One thing learned is One thing to improve is