Understanding Geometry: Lateral Area, Total Area, and Volume of Right Prisms
This educational content covers the concepts of lateral area, total area, and volume calculation for right prisms, specifically focusing on rectangular, triangular, trapezoidal, and hexagonal right prisms. It includes detailed explanations, formulas, and examples to help you grasp these geometric principles effectively.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
Geometry/Trig Unit 10 Lateral Area, Total Area and Volume Name: _________________________ Lateral Area, Total Area, & Volume page 2
Day 1: Rectangular Prism Notes Date: ____________________________________ Rectangular Prism: _____________________________________________________________ ___________________________________________________________________________ Total Area: ___________________________________________________________________ ____________________________________________________________________________ Lateral Area: _________________________________________________________________ 6cm How do we find Total Area? Example 1 Find the area of each face: Front: ___________ Back: ____________ Top: ____________ Bottom: __________ Left Side: ________ Right Side: _______ Total: ___________ How do you find the Lateral Area? _________________________________________ Formula for the Lateral Area: ________________________________ Formula for the Total Area of a Rectangular Prism: ________________ 8cm 10cm Lateral Area: ___________ Total Area: __________ Example 3 Find the lateral area: ________________ Find the total area: _______________ Example 2 Find the lateral area: ________________ Find the total area: _______________ 9in 6m 9in 6m 20m 9in Volume: _____________________________________________________________________ Units: ______________________________________________________________________ Formula for Volume of a Rectangular Prism: ___________________________________________ Revisit Example 1: Dimensions: 6cm, 8cm, 10cm Find the volume: __________________ Revisit Example 2: Dimensions: 6m, 6m, 20m Find the volume: __________________ Revisit Example 3: Dimensions: 9in, 9in, 9in Find the volume: __________________ page 3
Other Right Prisms - Notes Date: ____________________________________ page 4 Volume (V) = Area of the Base x height of the prism (V = Bh) Perimeter of Base (p) Lateral Area (L.A) = Perimeter of Base x height (L.A = ph) height (h) Total Area (T.A) = Lateral Area + 2(Area of the Base) (T.A. = L.A. + 2B) Area of Base (B) Example 1 Triangular Right Prism Example 2 Triangular Right Prism 12m 12m 10m 10m 6m 4m 8m 7m 8m 7m 16m 14m 12m Volume: ________________ Volume: ________________ Lateral Area: ________________ Lateral Area: ________________ Total Area: ________________ Total Area: ________________ Example 3 Trapezoidal Right Prism 30m Example 4 Hexagonal Right Prism 6cm Base is a Regular Hexagon 10m 6cm 40m 12cm 14m 18m 8m Volume: ________________ Volume: ________________ Lateral Area: ________________ Lateral Area: ________________ Total Area: ________________ Total Area: ________________
Unit 10, Worksheet 1 Find the Volume, Lateral Area, and Total Area of each figure. Date: ____________________________________ 1. 2. 100m 42m 42m 24m 20m 28m 36m 18m 12m 18m 24m Volume: ________________ Volume: ________________ Lateral Area: ________________ Lateral Area: ________________ Total Area: ________________ Total Area: ________________ 3. 4. 100m 60m 16m 75m 40m 24m 70m 28m Volume: ________________ Volume: ________________ Lateral Area: ________________ Lateral Area: ________________ Total Area: ________________ Total Area: ________________ page 5
Unit 10, Worksheet 2 Find the Volume, Lateral Area, and Total Area of each figure. Date: ____________________________________ page 6 1. 8cm 2. Base is a Regular Hexagon 10m 6m 6m 5m 15cm 7.5m 12.5m Volume: ________________ Volume: ________________ Lateral Area: ________________ Lateral Area: ________________ Total Area: ________________ Total Area: ________________ 3. 4. 4in 70m 16in 20m 24m 22m 28m 50m Base is a square. Volume: ________________ Volume: ________________ Lateral Area: ________________ Lateral Area: ________________ Total Area: ________________ Total Area: ________________
Day 2: Cylinder Notes Date: ____________________________________ page 7 A cylinder is like the right prisms with which we have been working this week, except that the bases of a cylinder are circles. The volume and total area can be calculated in a very similar manner. In a cylinder, the formula for Volume is exactly the same. Multiply the Area of the Base (B) by the height (h). In this case the base is a circle. Recall that the area of a circle is calculated by using A = ________. The Lateral Area and Total Area is calculated in a similar manner. However we must replace perimeter of base with ____________________________________________, use _________ Therefore, to find the Total Area and Volume of a cylinder you must still calculate the same three pieces of information: 1. ________________ of the base 2. ________________ of the base _____________ 3. Height of the object given Example 1 Example 2 14m 7m 10in 4in Radius ___________ Height - ____________ Radius ___________ Height - ____________ Area of Base _________________________ Area of Base _________________________ Circumference of Base __________________ Circumference of Base __________________ Volume _____________________________ Volume _____________________________ Lateral Area - __________________________ Lateral Area - __________________________ Total Area - ___________________________ Total Area - ___________________________
Unit 10, Worksheet 3 Date: ____________________________________ page 8 1. 2. 2yd 47ft 24ft All answers must be in feet. 13ft Volume: ________________ Volume: ________________ Lateral Area: ________________ Lateral Area: ________________ Total Area: ________________ Total Area: ________________ 3. 4. 25m 10ft 23ft 10m 22m 23ft 13ft 15m 15m 25ft 15m Volume: ________________ Volume: ________________ Lateral Area: ________________ Lateral Area: ________________ Total Area: ________________ Total Area: ________________
Day 3: Cones Notes Date: ____________________________________ Volume - _______________________________________________________________ Lateral Area - __________________________________________________________ Total Area - ____________________________________________________________ Therefore, now we need to find the four key pieces of information first: 1. Area of the base ___________ 2. Circumference of the base - _______________ 3. Height - ____________________ 4. Slant height - __________________________ Example 1: Radius - _________________________________________ Area of the base _________________________________ Circumference of the base __________________________ Height - _________________________________________ Slant height _____________________________________ Lateral Area - ____________________________________ Total Area - ______________________________________ Volume - _________________________________________ Radius - ______________________________________________ Area of the base ______________________________________ Circumference of the base _______________________________ Height - ______________________________________________ Slant height _________________________________________ Lateral Area - _________________________________________ Total Area - __________________________________________ Volume - _____________________________________________ 10m 6m Example 2 24cm 26cm page 9
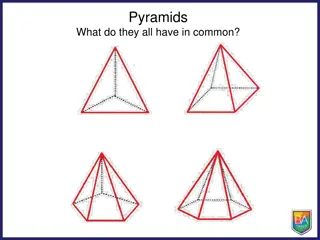
Day 3: Pyramid Notes We will be looking at square pyramids only. Date: ____________________________________ page 10 Lateral Area - _________________________________________________________________ Total Area - __________________________________________________________________ Volume - _____________________________________________________________________ Therefore, we need to find the following four pieces of information for each problem: 1. Area of the base A = e2 2. Perimeter of the base P = 4e 3. Height h 4. Slant height - l Example 1 Base Edge - ___________________________________ Height ______________________________________ Slant Height _________________________________ Area of the base ______________________________ Perimeter of the base - __________________________ Lateral Area - _________________________________ Total Area - ___________________________________ Volume - ______________________________________ 10in = l 12in = e Example 2 Base Edge - ___________________________________ Height ______________________________________ Slant Height _________________________________ Area of the base ______________________________ Perimeter of the base - __________________________ Lateral Area - _________________________________ Total Area - ___________________________________ Volume - ______________________________________ 15ft 16ft
Unit 10, Worksheet 4 Cones & Pyramids Date: ___________________________ page 11 1. 2. 24ft 17in 20ft 8in Volume: ________________ Volume: ________________ Lateral Area: ________________ Lateral Area: ________________ Total Area: ________________ Total Area: ________________ 3. 4. 61m 25in 60m 14in Volume: ________________ Volume: ________________ Lateral Area: ________________ Lateral Area: ________________ Total Area: ________________ Total Area: ________________
Unit 10, Worksheet 5 Mixed Review - Day 4 Date:___________________________ page 12 1. 2. Area of square base is 324mm2. Circumference of Base is 60 ft. 40ft 12mm Volume: ________________ Volume: ________________ Lateral Area: ________________ Lateral Area: ________________ Total Area: ________________ Total Area: ________________ 3. 4. 12in 6in 18in 10in 7in 7in A = 36 in2 8in Volume: ________________ Volume: ________________ Lateral Area: ________________ Lateral Area: ________________ Total Area: ________________ Total Area: ________________
Day 5: Sphere Notes Date: ____________________________________ Sphere - _______________________________________________________________ ______________________________________________________________________ Volume - ____________________________________ Total Area - _________________________________ Example 1 Find the Total Area and Volume of the Sphere Radius - __________________________________ 6in Volume - _________________________________ Total Area - _____________________________ Example 2 Find the Total Area and Volume of the spherical model of a planet The length of the equator is 30 mm. Radius - __________________________________ Volume - _________________________________ Total Area - _____________________________ page 13
Unit 10, Worksheet 6 Mixed Review Date: __________________________ page 14 1. 2. The area of the circular base is 64 m2. 4m 17m Volume: ________________ Volume: ________________ Lateral Area: ________________ Total Area: ________________ Total Area: ________________ 3. 4. The circumference of the circular base is 8 cm. The height is twice the length of the diameter. 12m 5m 11m 13m Volume: ________________ Volume: ________________ Lateral Area: ________________ Lateral Area: ________________ Total Area: ________________ Total Area: ________________
page 15 Unit 10, Worksheet 6 - Mixed Review - Continued Date: ___________________________ 1. 2. 3in 2in 6m 7m Area = 70m2 6in 1.5in 6in Volume: ________________ Volume: ________________ Lateral Area: ________________ Lateral Area: ________________ Total Area: ________________ Total Area: ________________ 3. The area of the square base is 100m2. 4. The circumference is 22 ft. 13m Volume: ________________ Lateral Area: ________________ Volume: ________________ Total Area: ________________ Total Area: ________________
Geometry/Trig Day 6; Similar Solids Name: ____________________________________ Date: ____________________________________ Theorem 12-1 If the scale factor of two similar solids is a:b, then 1. The ratio of their perimeters is a:b. 2. The ratio of their base areas, lateral areas, and total areas is a2:b2. 3. The ratio of their volumes is a3:b3 Given the solids, determine the ratio of their totals areas and their volumes. Example 1: Scale Factor: _________________ Ratio of Total Areas: ___________ 6 10 Cubes Ratio of Volumes: ______________ All cubes are _________________. Example 2: Scale Factor: _________________ Similar Cylinders Ratio of Total Areas: ___________ 3 2 Ratio of Volumes: ______________ Example 3: All spheres are ________________. Scale Factor: _________________ 8 2 Ratio of Total Areas: ___________ Ratio of Volumes: ______________ Spheres page 16
Geometry/Trig Ratios Practice Unit 9 & 10 Name: __________________________ Date: ___________________________ 1. Two similar polygons have a scale factor of 3:5. What is the ratio of the perimeters? What is the ratio of the areas? 2. Two circles have radius lengths 4 and 12. What is the scale factor? What is the ratio of circumferences? What is the ratio of areas? 3. The ratio of areas of two squares is 9:16. What is the ratio of their perimeters? What is the ratio of their side lengths? 4. The ratio of areas of two similar polygons is 9:625. The perimeter of the smaller polygon is 12. What is the perimeter of the larger polygon? 5. The ratio of perimeters of two similar polygons is 4:15. The area of the smaller polygon is 64. What is the area of the larger polygon? 6. Two similar solids have a scale factor of 3:7. What is the ratio of their lateral areas? What is the ratio of their total areas? What is the ratio of their volumes? 7. Two spheres have diameters with lengths 10 and 20. What is the ratio of their radii? What is the ratio of their circumferences? What is the ratio of their total areas? What is the ratio of their volumes? 8. The ratio of volumes of two cubes is 27:2744. What is the scale factor? What is the ratio of lateral areas? What is the ratio of total areas? 9. The ratio of lateral areas of two similar solids is 25:36. What is the ratio of their volumes? 10. The ratio of total areas of two similar solids is 64:81. If the volume of the smaller solid is 1024, what is the volume of the larger solid? 11. The ratio of volumes of two similar solids is 1:729. The lateral area of the larger solid is 324, what is the lateral area of the smaller solid? page 17
Word Problems Draw a picture for each scenario. Leave all answers in terms of unless otherwise indicated. Date: ____________________________________ 1. A cylinder has a volume of 1728 . If the height equals the radius, find the total area of the cylinder. 2. If the lateral area of a cone equals 125 and the slant height is 5, find the radius. 3. If the ratio of areas of two similar prisms is 4:169, find the ratio of volumes. 4. The total area of a cube is 2400m2. Find the volume. 5. A solid metal cylinder with radius 3 and height 3 is recast (melted down and then remolded) as a solid cone with radius 3. Find the height of the cone. 6. A solid metal ball with radius 4 cm is melted down and recast as a solid cone with the same radius. What is the height of the cone? 7. A manufacturer wants to pack cans of soup into a box. Assume the cans are cylinders and the box is a rectangular prisms and that the box is designed to fit as closely as possible around the cans. The manufacturer would like to fit 12 cans into one box (3 rows of 4 cans each). The diameter of each can is 6cm and the height of each can is 9cm. Determine the size of the necessary box and the amount of wasted space in the box. (Convert answer of wasted space into a decimal and round to the nearest tenth.) page 18
8. The surface area (total area) of a sphere is cm2. Find the diameter of the sphere. page 19 9. Two similar cylinders have lateral areas 81 and 144 . Find the ratio of the heights. If the height of the smaller cylinder is 12, find the height of the larger cylinder. 10. A regular square pyramid has a base edge of 3cm and a volume of 135cm2. Find the height. 11. Find the volume and total area of a sphere that has a circumference of 122 . 12. A cylinder with radius 7cm has a total area of 168 cm2. Find its height. 13. A cone has a diameter of 18 cm and a slant height of 15 cm. How much water can fit in this cone? (1cm3 = 1mL) 14. If two similar cones have a ratio of lateral areas of 144:25, find the ratio of the volumes. If the volume of the larger cone is 6912, find the volume of the smaller cone. 15. If two similar prisms have a ratio of volumes of 8:2197, find the ratio of total areas. Suggested Book Word Problems: p. 479 #23-28; p. 493 #18-21; p. 494 #22-24; p. 495 Challenge; p. 501 #19-20, 23, 24; p. 511 #1-8
Geometry/Trig Day 7:Total Area and Volume Application Questions Date: ____________ Directions: Work with your group to solve each application problem. You will be assigned one problem to present to the class. Be sure to label all answers and round any values appropriately. Name: __________________________ page 20 1. A manufacturer needs to fit 6 soccer balls in a box for shipping. The balls are organized in 3 columns of 2 balls each. Each soccer ball has a radius of 4 inches. If the box fits around the balls as closely as possible, find the volume of the box and the wasted space inside the box. How much cardboard (in square inches) is required to create the box? (Assume the soccer balls are perfect spheres.) Volume of Box: ___________________ Wasted Space: ___________________ Amount of Cardboard: _____________ 2. A spherical scoop of ice cream with a diameter of 6cm is placed in an ice cream cone with a diameter of 5cm and a height of 12cm. Is the cone big enough to hold all of the ice cream if it melts? If it is, how much extra space will be available? If it is not, how much more space would be needed to hold all of the ice cream? Is the cone big enough? _________________ Extra Space or Needed Space: ____________ 3. A solid metal cone with radius 3cm and height 2cm is melted down and recast as a solid cylinder with height of 1.5cm. Find the radius of the cylinder. What is the total area of the original cone and the new cylinder? Radius of Cylinder: ________________ Total Area of Cone: _______________ Total Area of Cylinder: _____________
Geometry/Trig Total Area and Volume Application Questions 4. A manufacturing company has constructed 10,000 solid metal spherical balls with a circumference of 16 cm for use in a product. After feedback from their customers, they have determined that a cone shaped solid with the same volume and same radius would be more effective. If each solid metal ball is melted down and recast as a solid cone, what is the height of each new cone? Name: __________________________ Date: ____________________ page 21 Height of Cone: ____________ Customers have also requested that the cones be painted yellow to match the product. Each quart of special paint costs $43.98 and advertises that it covers 12m2. How many quarts will be needed? What will the cost be to paint the newly constructed set of solid cones? Number of Quarts: __________ Cost: ____________________ 5. You are going to paint a barn. The barn is made up of two solids: a square pyramid sitting on a rectangular prism. The base of the pyramid and rectangular prism are congruent. The base edge of the square pyramid is 24 feet. The height of the rectangular prism is 10 feet. The height of the entire barn is 15 feet. Find the total area to be painted. (Assume there are no windows and that you paint the roof.) One gallon of paint covers approximately 350 square feet of space. How many gallons of paint will you need? If each gallon of paint costs $24.75, what will be the total cost for paint? Total Area to be Painted: _________________ Number of Gallons Required: ______________ Cost: ________________________________
Geometry/Trig Total Area and Volume Application Questions Name: __________________________ Date: ____________________ page 22 6. A silo barn consists of a cylinder and a hemisphere (half of a sphere). (The base of the cylinder and hemisphere are congruent). Find the volume and total area of the silo if the height of the cylinder is 20ft and the height of the silo barn is 25ft. Volume; ____________________ Total Area: _________________ You want to paint the barn. (Assume there are no windows and that you do paint the roof.) One gallon of paint covers approximately 350 square feet of space. What is the total area that needs to be painted? How many gallons of paint will you need? If each gallon of paint costs $22.50, what will be the total cost for paint? Total Area to be Painted: _____________ Gallons of Paint: ____________________ Total Cost: ________________________ 7. Water is pouring into a conical (cone-shaped) container at the rate of 1.8 m3 per minute. Find the volume of the cone. Find, to the nearest minute, then number of minutes it will take to fill the container. Volume of the Cone: _______________ Number of Minutes: ______________ The container has been emptied. A hole has been punctured in the bottom of the container and is allowing the water to escape at a rate of 1.2 m3 per minute. Now how long will it take to the nearest minute to fill the container? Number of Minutes: ______________