Understanding Surface Area and Volume in Geometry
Gain insights into calculating surface area and volume of geometric shapes, specifically focusing on rectangular prisms. Learn how to find surface area and lateral area using formulas, and explore examples illustrating these calculations step by step with images.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
Surface Area & Volume Geometry/Trig 2 Fall 2007
Rectangular Prism A three dimensional object with two rectangular bases and four rectangular lateral faces. Base (B) Base (B) R i g h t L e f t Front Back
Surface Area the sum of the areas of all of the faces (bases and lateral faces) of a solid. Lateral Area the sum of the areas of the lateral faces of a prism
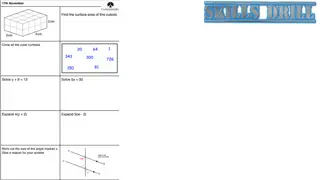
How do we find Surface Area? Example 1 Find the area of each face: Front: ____________ Back: ____________ Top: _____________ Bottom: __________ Left Side: ________ Right Side: _______ Surface: ___________ 376cm2 6cm 80cm2 80cm2 60cm2 60cm2 48cm2 48cm2 8cm 10cm
How do we find Lateral Area? Example 1 Find the area of each face: Front: ____________ Back: ____________ Top: _____________ Bottom: __________ Left Side: ________ Right Side: _______ Lateral Area: ___________ 6cm 80cm2 80cm2 60cm2 60cm2 48cm2 48cm2 8cm 10cm 256cm2
Formula for Lateral Area: Lateral Area = Perimeter of Base x Height L.A. = ph Formula for Surface Area: Surface Area = Lateral Area + 2(Area of the Base) T.A. = L.A. + 2B
Example 2 Find the Lateral Area and the Surface Area 6m 6m 20m L.A. = Perimeter of Base x Height p = 20 + 20 + 6 + 6 = 52m L.A. = 52 x 6 = 312m2 L.A. = ph h = 6m T.A. = L.A. + 2(Area of the Base) T.A. = L.A. + 2B L.A. = 312m2 B = 20 x 6 = 120m2 T.A. = 312 + 2(120) = 552m2
Example 3 Find the Lateral Area and the Surface Area 9in 9in 9in L.A. = Perimeter of Base x Height p = 9 + 9 + 9 + 9 = 36in L.A. = 36 x 9 = 324in2 L.A. = ph h = 9in T.A. = L.A. + 2(Area of the Base) L.A. = 324in2 B = 9 x 9 = 81in2 T.A. = 324 + 2(81) = 486in2 T.A. = L.A. + 2B
Volume - the amount of space that an object occupies The units for Volume are always cubed. Examples: in3, m3, cm3. Formula for Volume of a Rectangular Prism: V = Area of the base x height V = Bh
V = Area of the base x height V = Bh Example 1 6cm B = 6 x 10 = 60cm2 h = 8cm 8cm 10cm V = 60 x 8 = 480cm3
V = Area of the base x height V = Bh Example 2 6m 6m B = 6 x 20 = 120m2 h = 6m 20m V = 120 x 6 = 720m3
V = Area of the base x height V = Bh Example 3 9in B = 9 x 9 = 81in2 h = 9in 9in 9in V = 81 x 9 = 729in3
Example 4 Find the Lateral Area, Surface Area, and Volume of the rectangular prism. p = 7 + 7 + 13 + 13 = 40in h = 5in B = 7 x 13 = 91in2 7in 5in 13in L.A. = ph T.A. = L.A. + 2B V = Bh L.A. = 40 x 5 T.A. = 200 + 2(91) T.A. = 382in2 V = 91 x 5 V = 455in3 L.A. = 200in2
We use the same formulas for lateral area, surface area and volume when dealing with other right prisms. L.A. = ph T.A. = L.A. + 2B V = Bh Trapezoidal Prism Triangular Prism Hexagonal Prism
Example 1 12m L.A. = ph = (27)(12) L.A. = ph = 324m2 4m 8m 7m 12m T.A. = L.A. + 2B = 324m2 + 2(24) T.A. = 372m2 V = Bh = (24)(12) V = 288m3 Area of the Base (B) = (12)(4) = 24m2 Perimeter of Base (p) = 12 + 8 + 7 = 27m Height (h) = 12m
Example 2 10m 10m 6m 16m 14m V = Bh = (48)(14) V = 672m3 L.A. = ph = (36)(14) L.A. = 504m2 T.A. = L.A. + 2B = 504 + 2(48) T.A. = 600m2
30m Example 3 B = h(b1 + b2) B = 10(8 + 30) B = 190m2 10m 40m 18m 14m 8m V = Bh = (190)(40) V = 7600m3 L.A. = ph = (70)(40) L.A. = 2800m2 T.A. = L.A. + 2B = 2800 + 2(190) T.A. = 3180m2
6cm Example 4 V V = Bh 648 54 ( 3 . L . L 2 + . A . 3 3 )( 12 ) = = cm A A B = ph 432 432 432 . . 36 ( cm 2 + 108 )( 12 ) = = = 12cm 2 T A . . . L A T . 54 ( 3 3 ) 2 = = cm + Area of Base (B) = (36) = 54 3 3 3 6cm 30 3 3 Area of Base (B) = ap 3cm
Cylinders Cylinders are very similar to the right prisms that we have been examining. The only difference is that instead of polygons (rectangle, triangle, trapezoid, hexagon) as bases, a cylinder has circular bases. The formulas to calculate lateral area, Surface area, and volume will be nearly the same as prisms.
The formula for Volume remains the same. (V = Bh). Because in this case the base is a circle, we must use the formula for finding area of a circle. Recall that area of a circle is calculated by using A = r2 The Lateral Area and Surface Area are calculated in a similar manner. However we must replace perimeter of base with circumference of base. C = 2 r
Therefore, to calculate Surface Area and Volume of a cylinder you must find three key pieces of information: 1. Area of the Base r2 2. Circumference of the Base 2 r 3. Height of the object - given
Example 1 Find the Lateral Area, Surface Area, and Volume of the Cylinder. Radius Area of Base = Circumference = Height = Volume = L.A. = T.A. = 80 + 2(16 ) 4in 10in 16 in2 8 in 4in 10in (16 )(10) 160 in3 = = (8 )(10) 80 in2 = 112 in2
Example 2 7m Radius Area of Base = Circumference = Height = Volume = L.A. = T.A. = 196 + 2(49 ) 7m 14m 49 m2 14 m 14m (49 )(14) (14 )(14) 686 m3 196 m2 294 m2 = = =
Example 3 75cm Radius Area of Base = Circumference = Height = Volume = L.A. = T.A. = 7500 + 2(2500 ) 50cm 2500 cm2 100 cm 100cm 75cm (2500 )(75) 187500 cm3 (100 )(75) = 7500 cm2 = = 12500 cm2
Example 4 You can have decimals in your answers, if there are decimals in the problems. 22.8in Radius Area of Base = 182.25 in2 Circumference = Height = Volume = L.A. = T.A. = 615.6 + 2(182.25 ) 13.5in 27in 27 in 22.8in (182.25 )(22.8) (27 )(22.8) 4155.3 in3 = = 615.6 in2 = 980.1 in2
Homework Answers: p. 6 1. V = 9072m3 2. V = 60000m3 L.A. = 11200m2 T.A. = 12400m2 3. V = 525000m3 L.A. = 25500m2 T.A. = 39500m2 p. 7 4. V = 19200m3 L.A. = 5520m2 T.A. = 6160m2 5. V = L.A. = 720cm2 T.A. = 6. V = 68600m3 L.A. = 8120m2 T.A. = 10080m2 L.A. = 3276m2 T.A. = 3708m2 ( ) 1440 cm 3 3 720 + 192 3 cm 2
Homework Answers: p. 11 1. V = 7943 ft3 L.A. = 1222 ft2 T.A. = 1560 ft2 2. V = 864 ft3 L.A. = 288 ft2 T.A. = 360 ft2 3. V = 180 in3 L.A. = 60 in2 T.A. = 132 in2
Day 2 Pyramids & Cones A cone has one circular base. Slant Height (l) Height (h) Radius (r)
Formula for Volume Volume = Area of the Base x Height 3 1 1 V = Bh 3 Formula for Lateral Area L.A. = Circumference of Base x Slant Height 1 . A . L = r 2 C = Cl . L A . rl = 2 Formula for Surface Area (Surface Area) Surface Area = Lateral Area + Area of Base B . A . L . A . T + =
Therefore, to calculate Surface Area and Volume of a Cone you must find four key pieces of information: 1. Area of the Base r2 2. Circumference of the Base 2 r 3. Height of the object h 4. Slant Height - l
Example 1 6m Radius (r) Area of the Base (B) Circumference of Base (C) Height (h) Slant Height (l) Lateral Area (L.A.) 36 m2 10m 8m 12 m 6m 8m 10m 1 1 12 ( )( 10 ) 60 m2 . L A . Cl = 2 2 Surface Area (T.A) 60 + 36 = 96 m2 T A . . . L A . B = + 1 1 36 ( )( 8 ) Volume (V) - V = Bh 3 3 96 m3
Example 2 9m Radius (r) Area of the Base (B) Circumference of Base (C) Height (h) Slant Height (l) Lateral Area (L.A.) L.A. = 135 m2 81 m2 15m 12m 18 m 9m 12m 15m 1 1 18 ( )( 15 ) . L A . Cl = 2 2 Surface Area (T.A) 135 + 81 = 216 m2 T A . . . L A . B = + 1 1 81 ( )( 12 324 m3 ) Volume (V) - V = Bh 3 3
Example 3 Lateral Area 260 cm2 Surface Area 360 cm2 Volume 800 cm3 Example 4 Lateral Area 15 in2 Surface Area 24 in2 Volume 12 in3
A regular square pyramid has a square base. Slant Height (l) Height (h) Base Edge (e)
Formula for Volume Volume = Area of the Base x Height 3 1 1 V = Bh 3 Formula for Lateral Area L.A. = Perimeter of Base x Slant Height 1 . A . L = pl 2 Formula for Surface Area Surface Area = Lateral Area + Area of Base B . A . L . A . T + =
Therefore, to calculate Surface Area and Volume of a Pyramid you must find four key pieces of information: 1. Area of the Base e2 2. Perimeter of the Base 4e 3. Height of the object h 4. Slant Height - l
Example 1 12in Base Edge (e) Height (h) Slant Height (l) Area of Base (B) Perimeter of Base (p) Lateral Area (L.A.) 10in = l 8in 8in = h 10in 144in2 12in = e 48in 48 ( 240in2 1 1 )( 10 ) . L A . pl = 2 2 Surface Area (T.A) 240 + 144 = 384in2 T A . . . L A . B = + 1 1 144 ( )( 384in3 8 ) V = Bh Volume (V) - 3 3
Example 2 24in = h 20in Base Edge (e) Height (h) Slant Height (l) Area of Base (B) Perimeter of Base (p) Lateral Area (L.A.) 26in 26in = l 24in 24in 26in 10in 400in2 20in = e 80in 80 ( 1 1040in2 1 )( 26 ) . L A . pl = 2 2 Surface Area (T.A) 1040 + 400 = 1440in2 T A . . . L A . B = + 1 1 ( 400 )( 24 3200in3 ) V = Bh Volume (V) - 3 3
Example 3 height = 12m Lateral Area 260m2 Surface Area 360cm2 Volume 400cm3 Example 4 Lateral Area 544ft2 Surface Area 800ft2 Volume 1280ft3
Sphere the set of all points a given distance away from a center point. Volume - 4 V r 3 = 3 Surface Area - T A . . 4 r 2 =
Example 1 Find the Surface Area and Volume of the Sphere Radius 6in Volume - 3 in 288 V = 6in 4 4 V 6 3 V r 3 = = 3 3 T.A = 4 r2 Surface Area - T.A = 4 62 T.A = 144 in2
Example 2 r = 15mm V = 4500 mm3 T.A. = 900 mm2 Example 3 r = 1cm V = T.A. = 4 cm2 Example 4 r = 13.1ft V = 2997.5 ft3 T.A. = 686.4 ft2 4 cm 2 3
Similar Solids Theorem 12-1 If the scale factor of two similar solids is a:b, then 1. The ratio of their perimeters is a:b. 2. The ratio of their base areas, lateral areas, and Surface areas is a2:b2. 3. The ratio of their volumes is a3:b3.
Cubes 6 10 Scale Factor: 6 to 10 = 3 to 5 9 to 25 Ratio of Surface Areas: All Cubes are Similar. Ratio of Volumes: 27 to 125
Similar Cylinders 2 3 3 to 2 Scale Factor: Ratio of Surface Areas: 9 to 4 Ratio of Volumes: 27 to 8
Spheres 8 2 8 to 2 = 4 to 1 Scale Factor: Ratio of Surface Areas: 16 to 1 Ratio of Volumes: 64 to 1 All Spheres are Similar.
Warm Up The circumference of the earth is approximately 40820km. If you assume that the Earth is a perfect sphere, find its volume and Surface area. (Use = 3.14). Answer for Volume will need to be in scientific notation.
Homework Answers: p. 30 1. Sphere 256 V m 3 = 3 T.A. = 64 m2 2. Cone L.A. = 136 m2 V = 320 m3 T.A. = 200 m2 3. Cylinder L.A. = 17.2 cm2 T.A. = 25.2 cm2 V = 17.2 cm3