Understanding Measurement: Calculating Perimeter, Area, and Volume

Dive into the world of measurement with a focus on calculating perimeter, area, and volume for shapes like rectangles, triangles, circles, and prisms. Learn key formulas and methods to determine these crucial metrics through practical examples and step-by-step calculations.

• Measurement
• Perimeter
• Area
• Volume
• Formulas

teodor Follow

Uploaded on Jul 15, 2024 | 1 Views

Download Presentation

Please find below an Image/Link to download the presentation.

The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.

Presentation Transcript

1. TOPIC TOPIC 2 2: Measurement : Measurement Section 2.4: Calculating perimeter, area and volume Section 2.4: Calculating perimeter, area and volume

2. The following sections will be covered: 2.1 Conversions 2.2 Time 2.3 Length, distance and weight 2.4 Calculating perimeter, area and volume 2

3. In section 2.4, learners will be able to: Calculate the perimeter and area of rectangles, triangles and circles using given formulae Calculate the volume of rectangular prisms and cylinders using given formulae Calculate the surface area of rectangular box and a cylinder using given formulae. 3

4. Let us remind ourselves of the following formulae: Perimeter of rectangle = l b Perimeter of triangle = s + s + s (sum of 3 sides) Circumference of circle = ? diameter or 2?r Area of rectangle = 2(l + b) Area of triangle = b h Area of circle = ??2 NOTE: ? = 3,142

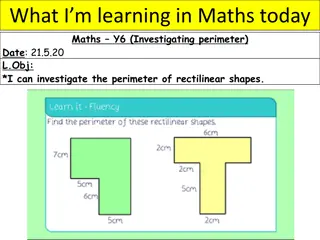
6. 1. Calculate the perimeter and area of the polygon below: 2. Calculate the circumference and area of the circle with diameter of 6 m 6 m

7. 1. Step : To calculate the perimeter you add all the sides. (if one side does not have a measurement, use the properties of the shape to find the other side) In this shape have a combination of rectangle and triangle. In a rectangle opposite sides is equal a = 5 cm Perimeter of rectangle will be = 3 + 5 + 5 + 2 + 2 P = 17 cm Perimeter of triangle = 2 + 2 + 3 P = 7 cm

8. Step: To calculate the area: Calculate the area of each shape; Area of Rectangle = l x b = 3 5 = 15 ??2 Area of Triangle = b h = 3 1,5 = 2,25 ??2 Calculate the total area by adding the two areas A(total) = A (rectangle) + A (triangle) = 15 + 2,25 = 17,25 ??2

9. In a circle, we call perimeter the circumference (C) 2. If the diameter = 6, then the radius (r) = 3 because the diameter = 2 radius Note for ? we use 3,142 A = ??2 = 3,142 32 = 28, 278 ?2 C = ? diameter OR C = 2?r = 3,142 6 = 2 3,142 3 = 18,852 m = 18,852 m

10. 1. (a) Calculate the area of the unshaded part for the shape below (b) Calculate the area of the shaded part for the shape below

11. 2. Calculate the area of the shape below with radius of 4 cm

12. 1. (a) A (unshaded part) = 4 3 (b) A (circle) = ??2 A (shaded part) = A (circle) A (unshaded part) = 19,6 ?2 - 12 ?2 = 7,6 ?2 The shape is of the full circle Area = ??2 = 3,142 42 = 37 7 ?2 = 12 ?2 = 3,142 2,52 = 19, 6375 19,6 ?2 2.

13. Volume is the space occupied by a three-dimensional (3-D) object Volume is measured in cubic units e.g. ??3, ?3

14. Calculate the volume of the following shapes: 1. r = 20 cm 2. 120 cm 80 cm80 cm

15. 1. The first shape is a cylinder Volume of cylinder = ? ?2 h = 3,142 202 60 = 75 408 ??3 2. Volume of box = l b h = 80 80 120 = 768 000 ??3

16. The surface area of an object is the area of all its faces.

17. Calculate the surface area of rectangular prism with 1. length = 12 cm, width = 90 cm and height = 0,1m Calculate the total surface area of the refuse bin 2. without lid, with diameter 24 cm and height 26 cm. Calculate the total surface area of a candle which is 3. 22,5 cm tall and has a radius of 5,5 cm.

18. l = 12 cm, w = 90 cm height = 0,1 m 1. Change all units to cm l = 12 cm, w = 9 cm, height = 10 cm Total surface area = 2 (l w) + 2 (w h) + 2 (l h) = 2 (12 9) + 2 (9 10) + 2 (12 10) = 216 + 180 + 240 = 636 ??2 Diameter = 24 cm, and radius (r ) = 12 cm Total surface area = ? ?? + 2?rh (refuse bin is without lid, so it is not closed) = 3,142 122 + 2 3,142 12 26 = 452,448 + 1 960,608 = 2 413,056 ??2 2.

19. 3. Height = 22,5 cm and radius (r ) = 5,5 cm Total surface area = 2 ? ?? + 2?rh = 2 3,142 5,52 + 2 3,142 5,5 22,5 = 190,091 + 777,645 = 967,736 ??2 = 967,7 ??2

20. Piet uses a cylindrically shaped bucket to carry water from a large rectangular tank. The tank has length = 2,5 cm, breadth = 3 m and height = 2 m. See figure 5 below and answer the questions in next slide.

21. Calculate the volume of the tank in ?3 Convert the answer in question 1 above to litres of water. 1 ??3 = 1 ml and 1 ? = 1 000 ml Calculate the volume of the bucket if the diameter is 30 cm and the height is 28,5 cm Convert the volume of the bucket to litres and round the answer to the nearest litre. How many full buckets of water can be poured from the large water tank? 1. 2. 3. 4. 5.

22. V = l b 1. = 2,5 3 2 = 15 ?3 V = 15 ?3 = 15 (100)3??3 = 15 1 000 000 ml = 15 1 000 ? = 15 000 ? 2.

23. 3. V = ??2 h = 3,142 152 28,5 = 20 148,075 ??3 4. V = 20 148,075 ??3 = 20 148,075 ml = 20,148075 ? 20 ? 5. Number of buckets = volume of tank volume of bucket = 15 000 20 = 750 buckets

24. The ______ of an object is the area of all its faces. Perimeter A. Circle B. Surface area C. Volume D.

25. Volume is measured in _______ units: Square A. Cubic B. Metric C. Imperial D.

26. The circumference of the round bottle top with radius 16 mm = : 10,05 ??3 A. 100,5 ??2 B. 100,5 C. 100,5 mm D.

27. The total surface area of a plastic pipe without top and base, and with a diameter of 4 cm and height of 10 cm will be: 125,68 ??2 A. 125,68 cm B. 213,65 ??2 C. 131,964 cm D.

28. The area of the shape below with a diameter of 12 cm is 75,408 ??2 A. 84,8 ??2 B. 113,1 ??2 C. 28,3 ??2 D.

29. The surface area of a rectangular prism with length =12 cm, width 90 mm and height 0,1 m is: 318 ??2 A. 618 ??2 B. 528 ??2 C. 631 ??2 D.

30. Below is a diagram of a tin of Italian tomatoes. The label is pasted around the tin but does not over lap at all. The radius of the tin is 3,5 cm and the height is 10,5 cm. What is the volume of the tin? A: 404,14 ??3 B: 404,14 ??2 C: 161 ??3 D: 161,0 ??2

31. Calculate the length of the label of the tin in the previous slide 3,5 cm A. 7 cm B. 10,5 cm C. 14 cm D.

32. Calculate the breadth of the label of the tin in slide no. 30 10,5 cm A. 7 cm B. 3,5 cm C. 14 cm D.

33. 2 500 l = ____ ?3 0,25 A. 25 B. 2,5 C. 250 D.

34. C B D A B D A C B 1. 2. 3. 4. 5. 6. 7. 8. 9. 10. C