Proving Triangles Congruent: Methods and Examples
SECTION 4.4
MORE WAYS TO PROVE
TRIANGLES CONGRUENT
POSTULATE 19
•
Angle-Side-Angle (ASA) Congruence Postulate
•
If two angles and the included side of one triangle are
congruent to two angles and the included side of a second
triangle, then the two triangles are congruent.
C
<A = <D; AC = DF; <C =<F;
F
∆ABC≌∆DEF
THEOREM 4.7
•
Angle-Angle-Side (AAS) Congruence Theorem
•
If two angles and a non-included side one triangle are congruent
to two angles and the corresponding non-included side of a
second triangle, then the two triangles are congruent.
<A
≌<D, <B
≌<E, BC ≌ EF then ∆ABC ≌∆DEF
EXAMPLE 1:
Is it possible to prove these triangles are congruent? If
so, state the postulate or theorem you would use.
Explain your reasoning.
EXAMPLE 2:
Is it possible to prove these
triangles are congruent? If
so, state the postulate or
theorem you would use.
Explain your reasoning.
EXAMPLE 2:
In addition to the congruent
segments that are marked,
NP
NP. Two pairs of
corresponding sides are
congruent. This is
not
enough information
to
prove the triangles are
congruent.
PROVE
L
A
J
K
EX. 2 PROVING TRIANGLES ARE
CONGRUENT
Given: AD
║EC, BD
BC
Prove:
∆ABD
∆EBC
Plan for proof: Notice that
ABD and
EBC are
congruent. You are given
that
BD
BC
. Use the fact that
AD
║EC to
identify a pair of
congruent angles.
PROOF:
Statements:
1.
BD
BC
2.
AD
║ EC
D
C
ABD
EBC
5.
∆ABD
∆EBC
Reasons:
1.
PROOF:
Statements:
1.
BD
BC
2.
AD
║ EC
D
C
ABD
EBC
5.
∆ABD
∆EBC
Reasons:
1.
Given
2.
Given
3.
Alternate Interior
Angles
4.
Vertical Angles
Theorem
Explore different methods such as ASA and AAS postulates and theorems to prove triangles congruent. Detailed examples and proofs provided for better understanding.
Download Presentation
Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author.If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
You are allowed to download the files provided on this website for personal or commercial use, subject to the condition that they are used lawfully. All files are the property of their respective owners.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author.
E N D
Presentation Transcript
SECTION 4.4 MORE WAYS TO PROVE TRIANGLES CONGRUENT
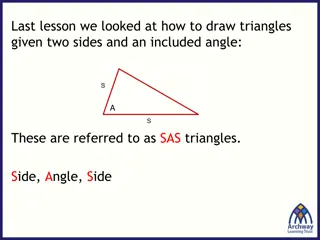
POSTULATE 19 Angle-Side-Angle (ASA) Congruence Postulate If two angles and the included side of one triangle are congruent to two angles and the included side of a second triangle, then the two triangles are congruent. C F E B D A <A = <D; AC = DF; <C =<F; ABC DEF
THEOREM 4.7 Angle-Angle-Side (AAS) Congruence Theorem If two angles and a non-included side one triangle are congruent to two angles and the corresponding non-included side of a second triangle, then the two triangles are congruent. F C E B D A <A <D, <B <E, BC EF then ABC DEF
EXAMPLE 1: Is it possible to prove these triangles are congruent? If so, state the postulate or theorem you would use. Explain your reasoning.
EXAMPLE 2: Is it possible to prove these triangles are congruent? If so, state the postulate or theorem you would use. Explain your reasoning.
EXAMPLE 2: In addition to the congruent segments that are marked, NP NP. Two pairs of corresponding sides are congruent. This is not enough information to prove the triangles are congruent.
PROVE Given: KL LA, KJ JA, AK bisects <LAJ Prove: ??? ??? L K A J
EX. 2 PROVING TRIANGLES ARE CONGRUENT Given: AD EC, BD BC Prove: ABD EBC C A Plan for proof: Notice that ABD and EBC are congruent. You are given that BD BC B E D . Use the fact that AD EC to identify a pair of congruent angles.
C A B PROOF: E D Statements: 1. BD BC 2. AD EC 3. D C 4. ABD EBC 5. ABD EBC Reasons: 1.
C A B PROOF: E D Statements: 1. BD BC 2. AD EC 3. D C 4. ABD EBC 5. ABD EBC Reasons: 1. Given 2. Given 3. Alternate Interior Angles 4. Vertical Angles Theorem
