Multiple Linear Regression: An In-Depth Exploration
Explore the concept of multiple linear regression, extending the linear model to predict values of variable A given values of variables B and C. Learn about the necessity and advantages of multiple regression, the geometry of best fit when moving from one to two predictors, the full regression equat
4 views • 31 slides
Multiple Regression in Statistics
Introduction to multiple regression, including when to use it, how it extends simple linear regression, and practical applications. Explore the relationships between multiple independent variables and a dependent variable, with examples and motivations for using multiple regression models in data an
1 views • 19 slides
Overview of Linear Regression in Machine Learning
Linear regression is a fundamental concept in machine learning where a line or plane is fitted to a set of points to model the input-output relationship. It discusses fitting linear models, transforming inputs for nonlinear relationships, and parameter estimation via calculus. The simplest linear re
0 views • 14 slides
Interpolation Techniques in Computer Analysis & Visualization
Explore the concepts of interpolation and curve fitting in computer analysis and visualization. Learn about linear regression, polynomial regression, and multiple variable regression. Dive into linear interpolation techniques and see how to apply them in Python using numpy. Uncover the basics of fin
3 views • 44 slides
Least-Squares Regression Line in Statistics
The concept of the least-squares regression line is crucial in statistics for predicting values based on two-variable data. This regression line minimizes the sum of squared residuals, aiming to make predicted values as close as possible to actual values. By calculating the regression line using tec
0 views • 15 slides
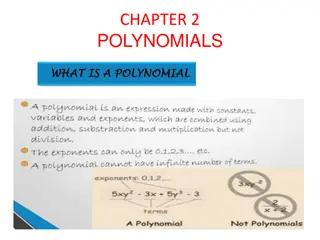
Introduction to Arithmetic Operations on Polynomials
This unit focuses on developing an understanding of polynomials in mathematical expressions. You will learn about the parts of a polynomial, polynomial operations, and representing polynomials. The topics cover performing arithmetic operations on polynomials, identifying variables in expressions, le
1 views • 71 slides
Polynomial Functions and Operations
Polynomial functions are mathematical functions in the form of an expression involving variables and coefficients. They can be manipulated through operations like addition, subtraction, multiplication, and division. Learn about polynomial degrees, identifying polynomials, and performing various oper
0 views • 20 slides
Introduction to Binary Logistic Regression: A Comprehensive Guide
Binary logistic regression is a valuable tool for studying relationships between categorical variables, such as disease presence, voting intentions, and Likert-scale responses. Unlike linear regression, binary logistic regression ensures predicted values lie between 0 and 1, making it suitable for m
7 views • 17 slides
Linear Regression: Concepts and Applications
Linear regression is a statistical method for modeling the relationship between a dependent variable and one or more independent variables. It involves estimating and predicting the expected values of the dependent variable based on the known values of the independent variables. Terminology and nota
0 views • 30 slides
Polynomial Degrees and Special Names
The degree of a polynomial is determined by its highest exponent, with specific names for each degree level. From the basic constant to the nth degree polynomial, this guide showcases the different degrees and their characteristics, helping you grasp the concept of polynomial functions easily.
1 views • 18 slides
Polynomials: Types, Degrees, and Zeroes
Polynomial expressions consist of terms with non-zero coefficients. They can have any number of terms and different degrees. Linear polynomials have a degree of one, quadratic polynomials have a degree of two, and cubic polynomials have a degree of three. Zeroes of a polynomial are the values of the
0 views • 17 slides
Binary Logistic Regression and Its Importance in Research
Binary logistic regression is an essential statistical technique used in research when the dependent variable is dichotomous, such as yes/no outcomes. It overcomes limitations of linear regression, especially when dealing with non-normally distributed variables. Logistic regression is crucial for an
0 views • 20 slides
Polynomial Functions with Real Zeros
Learn how to identify and write polynomial functions that include real zeros, find zeros of given functions, explore the Fundamental Theorem of Algebra, and apply the Number of Zeros Theorem. Practice writing polynomial functions satisfying specific conditions.
0 views • 11 slides
Arctic Sea Ice Regression Modeling & Rate of Decline
Explore the rate of decline of Arctic sea ice through regression modeling techniques. The presentation covers variables, linear regression, interpretation of scatterplots and residual plots, quadratic regression, and the comparison of models. Discover the decreasing trend in Arctic sea ice extent si
1 views • 9 slides
Overdispersed Data in SAS for Regression Analysis
Explore the concept of overdispersion in count and binary data, its causes, consequences, and how to account for it in regression analysis using SAS. Learn about Poisson and binomial distributions, along with common techniques like Poisson regression and logistic regression. Gain insights into handl
0 views • 61 slides
Conditional and Reference Class Linear Regression: A Comprehensive Overview
In this comprehensive presentation, the concept of conditional and reference class linear regression is explored in depth, elucidating key aspects such as determining relevant data for inference, solving for k-DNF conditions on Boolean and real attributes, and developing algorithms for conditional l
0 views • 33 slides
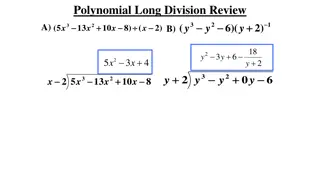
Polynomial Long Division Review and Practice
This content provides a detailed review on polynomial long division including step-by-step instructions, examples, and synthetic division practice problems. It covers topics such as descending polynomial order, solving binomial divisors, writing coefficients, determining remainders, and obtaining fi
0 views • 4 slides
Polynomial Division Methods and Examples
Dividing polynomials involves using methods like long division or equating coefficients. By applying these techniques, you can determine whether a polynomial divides exactly or leaves a remainder. The process is similar to long division of numbers, where the dividend is divided by the divisor to obt
0 views • 10 slides
Polynomial Division and Remainder Theorems Explained
Learn how to use long division to find quotients and remainders in polynomial problems. Understand when to use long division or synthetic division. Discover how the remainder theorem works by finding remainders when dividing specific polynomials by different factors. Explore the factor theorem and i
0 views • 6 slides
Curve Fitting and Regression Techniques in Neural Data Analysis
Delve into the world of curve fitting and regression analyses applied to neural data, including topics such as simple linear regression, polynomial regression, spline methods, and strategies for balancing fit and smoothness. Learn about variations in fitting models and the challenges of underfitting
0 views • 33 slides
Linear Regression and Gradient Descent
Linear regression is about predicting continuous values, while logistic regression deals with discrete predictions. Gradient descent is a widely used optimization technique in machine learning. To predict commute times for new individuals based on data, we can use linear regression assuming a linear
0 views • 30 slides
Multiclass Logistic Regression in Data Science
Multiclass logistic regression extends standard logistic regression to predict outcomes with more than two categories. It includes ordinal logistic regression for hierarchical categories and multinomial logistic regression for non-ordered categories. By fitting separate models for each category, suc
0 views • 23 slides
Strong List Coloring and the Polynomial Method in Graph Theory
Exploring the Polynomial Method in the context of Strong List Coloring, Group Connectivity, and Algebraic tools. This method involves proper coloring of graphs based on polynomial assignments, highlighting the significance of Strong Choosability and the Co-graphic case. The applications and proofs a
0 views • 34 slides
Methods for Handling Collinearity in Linear Regression
Linear regression can face issues such as overfitting, poor generalizability, and collinearity when dealing with multiple predictors. Collinearity, where predictors are linearly related, can lead to unstable model estimates. To address this, penalized regression methods like Ridge and Elastic Net ca
0 views • 70 slides
Polynomial Identity Testing in Algorithm Design
Explore the concept of polynomial identity testing as a powerful tool in algorithm design. Learn how to determine if a polynomial is identically zero by choosing random points and applying the Schwartz-Zippel Lemma. Discover the application of this technique in finding perfect matchings in bipartite
0 views • 26 slides
Approximating Knapsack Problem in Polynomial Time
In the recent discussion, we explored approximating the Knapsack problem in fully polynomial time. By utilizing a polynomial-time approximation scheme (PTAS), we aim to find a set of items within a weight capacity whose value is within a certain range of the optimal value. This approach involves lev
0 views • 22 slides
Signatures, Commitments, and Zero-Knowledge in Lattice Problems
Explore the intricacies of lattice problems such as Learning With Errors (LWE) and Short Integer Solution (SIS), and their relation to the Knapsack Problem. Delve into the hardness of these problems and their applications in building secure cryptographic schemes based on polynomial rings and lattice
2 views • 44 slides
Decision Problems in Polynomial Time Complexity
Decision problems play a crucial role in computational complexity theory, especially in the context of P and NP classes. These problems involve questions with yes or no answers, where the input describes specific instances. By focusing on polynomial-time algorithms, we explore the distinction betwee
0 views • 32 slides
Advanced Techniques in Secret Sharing Schemes
Explore the advancements in polynomial secret-sharing schemes and their applications in cryptography. Discover how polynomial schemes provide efficient solutions for sharing secrets among multiple parties while maintaining security. Learn about the construction of polynomial conditional disclosure p
0 views • 16 slides
Rational Functions Through Divided Differences and Newton Polynomial
Explore the mathematical approach of using divided differences and Newton Polynomial to determine an equation for a rational function passing through given points. The process involves creating a system of linear equations and utilizing Newton Polynomial to establish relationships between points. Va
0 views • 20 slides
Linear Regression Analysis: Testing for Association Between X and Y Variables
The provided images and text explain the process of testing for association between two quantitative variables using Linear Regression Analysis. It covers topics such as estimating slopes for Least Squares Regression lines, understanding residuals, conducting T-Tests for population regression lines,
0 views • 26 slides
Polynomials Operations: Adding, Subtracting, Multiplying - Unit 7 Days 1 and 2
Dive into the world of polynomial operations in this engaging unit covering adding, subtracting, and multiplying polynomials. Explore methods to combine like terms, distribute negative signs, and apply polynomial operations to solve problems. Practice sorting gumballs with like terms and creating nu
0 views • 23 slides
P, NP, NP-Hard, NP-Complete Problems and Amortized Analysis
This comprehensive study covers P, NP, NP-Hard, NP-Complete Problems, and Amortized Analysis, including examples and concepts like Reduction, Vertex Cover, Max-Clique, 3-SAT, and Hamiltonian Cycle. It delves into Polynomial versus Non-Polynomial problems, outlining the difficulties and unsolvability
0 views • 32 slides
Data Analysis and Regression Quiz Overview
This quiz covers topics related to traditional OLS regression problems, generalized regression characteristics, JMP options, penalty methods in Elastic Net, AIC vs. BIC, GINI impurity in decision trees, and more. Test your knowledge and understanding of key concepts in data analysis and regression t
0 views • 14 slides
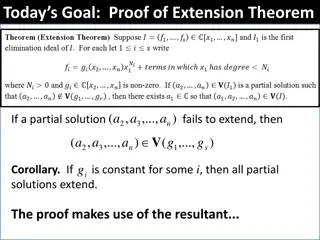
The Extension Theorem in Polynomial Mathematics
Explore the proof of the Extension Theorem, specializing in resultant calculations of polynomials and their extensions. Learn about Sylvester matrices, resultants, and how to make conjectures based on polynomial interactions. Take a deep dive into specializations and their implications in polynomial
0 views • 14 slides
Multivariate Adaptive Regression Splines (MARS)
Multivariate Adaptive Regression Splines (MARS) is a flexible modeling technique that constructs complex relationships using a set of basis functions chosen from a library. The basis functions are selected through a combination of forward selection and backward elimination processes to build a smoot
0 views • 13 slides
Zeroes of Polynomials - Grade 9 Chapter 2
Zeroes of a polynomial are the values of the variable that make the polynomial equal to zero. This concept is explored in Grade 9 Chapter 2, where students learn how to find the zeroes of a polynomial by equating it to zero. Through examples like p(x) = x - 4, students understand how to determine th
0 views • 10 slides
Multivariate Adaptive Regression Splines (MARS) in Machine Learning
Multivariate Adaptive Regression Splines (MARS) offer a flexible approach in machine learning by combining features of linear regression, non-linear regression, and basis expansions. Unlike traditional models, MARS makes no assumptions about the underlying functional relationship, leading to improve
0 views • 42 slides
Introduction to Machine Learning: Model Selection and Error Decomposition
This course covers topics such as model selection, error decomposition, bias-variance tradeoff, and classification using Naive Bayes. Students are required to implement linear regression, Naive Bayes, and logistic regression for homework. Important administrative information about deadlines, mid-ter
0 views • 42 slides
Algebraic Complexity and Equational Proofs in Arithmetic Formulas
Explore the intricacies of polynomial identity testing (PIT), equational proofs, and arithmetic formulas in the context of algebraic complexity. Learn about the minimal number of operations needed to compute the zero polynomial and derive new identities using derivation rules and axioms in polynomia
0 views • 39 slides