Polynomial Division and Remainder Theorems Explained

Learn how to use long division to find quotients and remainders in polynomial problems. Understand when to use long division or synthetic division. Discover how the remainder theorem works by finding remainders when dividing specific polynomials by different factors. Explore the factor theorem and its implication on polynomial functions. Get ready for upcoming quizzes and tests with practice exercises provided in the announcement section.

• Polynomial division
• Remainder theorem
• Factor theorem
• Synthetic division
• Polynomial functions

constable_m Follow

Uploaded on Sep 14, 2024 | 0 Views

Download Presentation

Please find below an Image/Link to download the presentation.

The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.

Presentation Transcript

1. Warm Up 9-1 Use long division to find the quotient and remainder for the problems below. 1. 3792 5 2. 4296 4

2. Announcements Quiz Friday, Test Wednesday Homework 2.4 pg. 205 Exercises # s 1-24 even

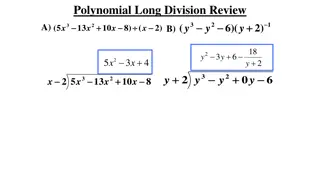
3. Long and Synthetic Division When is it better to use each one? Answers in Polynomial Form (Divisor)*(Quotient) +Remainder Fraction Form Quotient + Remainder/Divisor

4. Remainder Theorem If a polynomial is divided by x-k, then the remainder is r = f(k). Plug the zero into the function and you will get the remainder. What is the remainder when f(x)= 3x2+ 7x 20 is divided by the following? a) x 2 b) x + 1 c) x + 4

5. Factor Theorem A polynomial function f(x) has a factor x k if and only if f(k) = 0.

6. Homework 2.4 pg. 205 Exercises # s 1-24 even