Introduction to Arithmetic Operations on Polynomials

This unit focuses on developing an understanding of polynomials in mathematical expressions. You will learn about the parts of a polynomial, polynomial operations, and representing polynomials. The topics cover performing arithmetic operations on polynomials, identifying variables in expressions, learning about polynomial vocabulary such as variables and coefficients, and more.

• Polynomials
• Arithmetic Operations
• Mathematical Expressions
• Variables
• Coefficients

khadijah Follow

Uploaded on Jul 31, 2024 | 2 Views

Download Presentation

Please find below an Image/Link to download the presentation.

The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author.If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.

You are allowed to download the files provided on this website for personal or commercial use, subject to the condition that they are used lawfully. All files are the property of their respective owners.

The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author.

Presentation Transcript

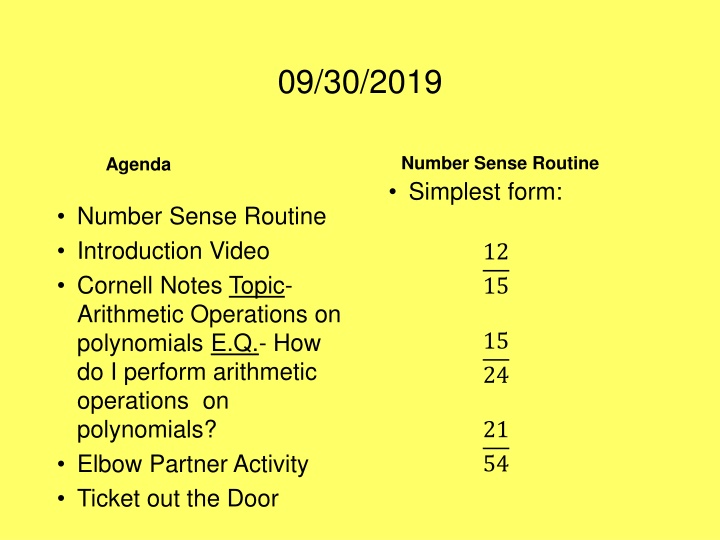
1. 09/30/2019 Number Sense Routine Simplest form: Agenda Number Sense Routine Introduction Video Cornell Notes Topic- Arithmetic Operations on polynomials E.Q.- How do I perform arithmetic operations on polynomials? Elbow Partner Activity Ticket out the Door 12 15 15 24 21 54

2. Mental Math Mental Math Mental Math Mental Math circle the variable(s) in each expression: 3x2 2x + 5 7a x2+ 3 + 2y

3. Polynomials Polynomials Polynomials Polynomials - Parts, Operations, & Representations Parts, Operations, & Representations - Approximately January 14th to February 24th

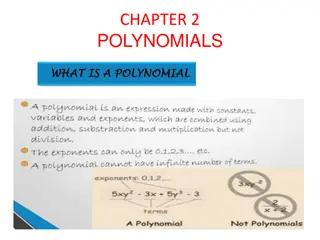
4. Intro Intro Intro Intro This unit will help you develop an understanding of polynomials, a form of mathematical expression. We will learn how to work with: - Parts of a Polynomial - Polynomial Operations (+)(-)(x)( ) - Representing Polynomials

5. Polynomials Polynomials Polynomials Polynomials Polynomial Polynomial http://t3.gstatic.com/images?q=tbn:ANd9GcTsAz03eV0C328qAX4gqywG9XqSq-TCPj9eolGicS6awE4O8DQW - Poly -many many - Nomial -terms terms

6. Polynomials Polynomials Polynomials Polynomials Polynomial Polynomial http://t3.gstatic.com/images?q=tbn:ANd9GcTsAz03eV0C328qAX4gqywG9XqSq-TCPj9eolGicS6awE4O8DQW - A numerical expression written with: -one term one term OR -sum/difference of sum/difference of terms terms -variables that variables that have whole have whole- - number number exponents exponents

7. Polynomials Polynomials Polynomials Polynomials - - Polynomial Vocabulary Polynomial Vocabulary - - - Variable Variable -Any letter that is used to represent a changing value (ex) 3x x2 - 2x x +5

8. Polynomials Polynomials Polynomials Polynomials - - Polynomial Vocabulary Polynomial Vocabulary - - - Coefficient Coefficient -Any number found at the beginning of a term containing a variable (ex) 3 3x2 - 2 2x +5

9. Polynomials Polynomials Polynomials Polynomials - - Polynomial Vocabulary Polynomial Vocabulary - - - Terms Terms -Each individual portion of the expression - Can be a number, variable, or the product of a number & a variable (ex) 3x2 - 2x +5

10. Polynomials Polynomials Polynomials Polynomials - - Polynomial Vocabulary Polynomial Vocabulary - - - Constant Constant -Any number by itself, the number does not change (ex) 3x2 - 2x +5 5

11. Polynomials Polynomials Polynomials Polynomials - - Polynomial Vocabulary Polynomial Vocabulary - - - Degree Degree -Refers to how big the exponent is (ex) 3x2 - 2x +5 - 3x2 = Degree of 2 - 2x = Degree of 1 - 5 = Degree of 0

12. Polynomials Polynomials Polynomials Polynomials Polynomial Example Polynomial Example - Variables x3, x2, -x - Coefficients -6, 4 - Constants 7 - # of Terms 4 - -6x 6x3 3 + 4x + 4x2 2 x + 7 x + 7 - Degree (H-L)

13. Polynomials Polynomials Polynomials Polynomials Polynomial Example Polynomial Example - Variables a3, b2, c, -x - Coefficients 3, -5 - Constants - - # of Terms 4 3a 3a3 3 - - 5b 5b2 2 + c + c - - x x - Degree (H-L)

14. Polynomials Polynomials Polynomials Polynomials - - Special Case Polynomial Vocabulary Special Case Polynomial Vocabulary - - - Monomial Monomial -Polynomial with one term (ex) 2m (ex) 8 (ex) n2

15. Polynomials Polynomials Polynomials Polynomials - - Special Case Polynomial Vocabulary Special Case Polynomial Vocabulary - - - Binomial Binomial -Polynomial with two terms (ex) 3x + 6 (ex) 5m2 2m

16. Polynomials Polynomials Polynomials Polynomials - - Special Case Polynomial Vocabulary Special Case Polynomial Vocabulary - - - Trinomial Trinomial -Polynomial with three terms (ex) 2x2 + 4x + 7

17. Polynomials Polynomials Polynomials Polynomials Polynomial Example Polynomial Example Identify each as a monomial, binomial, or trinomial: -5x2 4m2 + m + 6 5 x - 3

18. Polynomials Polynomials Polynomials Polynomials Guided Practice Guided Practice http://www.math- aids.com/cgi/pdf_viewer_2.cgi?script_na me=pre- algebra_mono_poly_id_type.pl&case_1=1 &case_2=1&case_3=1&case_4=1&case_ 5=1&case_6=1&language=0&memo=&an swer=1&x=162&y=37

19. Mental Math Mental Math Mental Math Mental Math Re-write, then label the following for each polynomial: (variable, constant, coefficient) 3x2 2x + 5 7a x2 + 3 + 2y

20. Polynomials Polynomials Polynomials Polynomials 1 1 Algebra Tiles Algebra Tiles x x2 2 x x - Polynomial expressions can be modeled using algebra tiles - -1 1 - -x x - -x x2 2

21. Polynomials Polynomials Polynomials Polynomials Algebra Tiles Algebra Tiles - Model example 3x2 2x + 5 x x2 2 - -x x - -x x x x2 2 x x2 2

22. Polynomials Polynomials Polynomials Polynomials Algebra Tiles Algebra Tiles Who can show how to model: 4m2 + m + 6

23. Polynomials Polynomials Polynomials Polynomials Algebra Tiles Algebra Tiles Who can show how to model: 5m2 2m

24. Polynomials Polynomials Polynomials Polynomials Mental Math Mental Math Please show with algebra tiles: 2m2 +3m 2 -6m 2 -2x2 +4m 6

25. 10.01.2019 Agenda Number Sense Routine Introduction Video Cornell Notes Topic- Arithmetic Operations on polynomials E.Q.- How do I perform arithmetic operations on polynomials? Elbow Partner Activity Ticket out the Door Number Sense Routine Simplest form: 18 20 6 16 35 45

26. Polynomials Polynomials Polynomials Polynomials A) A) - -16 B) x B) x 8 8 C) 4x C) 4x D) 2x D) 2x2 2 8x + 3 E) E) - -5x + 5 5x + 5 F) 5x F) 5x2 2 G) G) - -2x 2x2 H) H) - -3x 3x2 16 Monomial Monomial Binomial Binomial Monomial Monomial Trinomial Trinomial Binomial Binomial Monomial Monomial Trinomial Trinomial Binomial Binomial 8x + 3 2 + 2x + 2x 3 3 + 8 2 + 8

27. Mental Math Mental Math Mental Math Mental Math Please show with algebra tiles: 5m2 +2m 8 -2m 9 -3x2 +7m + 5

28. Mental Math Mental Math Mental Math Mental Math In each polynomial, identify which terms have the same variable, then identify which terms have the same degree (exponent). 7a + 3b2 -2a + 5 -b2 + 0 -9x2 +7m + x2 - 2 + 1m 2k

29. Polynomials Polynomials Polynomials Polynomials Simplifying Simplifying - Like terms Like terms can be simplified in a polynomial -Likes Terms have: -The same variable variable -The same degree degree (ex ex) x2 and 2x2 are like terms x2 -3 - x2 - 2x + 2 + x2 - x + x

30. Mental Math Mental Math Mental Math Mental Math Simplify the following polynomials by grouping like terms together, remember to represent it from Highest Degree to Lowest Degree: 7a + 3b2 -2a + 5 -b2 + 0 -9x2 +7m + x2 - 2 + 1m 2k

31. Polynomials Polynomials Polynomials Polynomials Polynomial Operations Polynomial Operations - - Addition & Subtraction Addition & Subtraction - - - When adding two or more polynomials together, each polynomial is sectioned off with brackets off with brackets sectioned (ex ex) 7s + 14 added to 6s2 + 2 6 is written as (7s + 14) + (-6s2 + 2 6 )

32. Polynomials Polynomials Polynomials Polynomials Polynomial Operations Polynomial Operations - - Addition & Subtraction Addition & Subtraction - - - Drop the brackets & combine like terms combine like terms - You should order your terms from highest degree to lowest degree highest degree to lowest degree (ex ex) (7s + 14) + (-6s2 + 2 6 )

33. Polynomials Polynomials Polynomials Polynomials Polynomial Operations Polynomial Operations - - Addition & Subtraction Addition & Subtraction - - Addition Practice One Addition Practice One (3x2 + 6) + (4x2 8)

34. Polynomials Polynomials Polynomials Polynomials Polynomial Operations Polynomial Operations - - Addition & Subtraction Addition & Subtraction - - Addition Practice Two Addition Practice Two 3x + 2 Find the perimeter of the following shape. 2x + 1

35. Polynomials Polynomials Polynomials Polynomials Polynomial Operations Polynomial Operations - - Addition & Subtraction Addition & Subtraction - - - When subtracting, it is important to remember your integer rules integer rules (ex ex) 2 (5) = 2 + (-5) = -3

36. Polynomials Polynomials Polynomials Polynomials Polynomial Operations Polynomial Operations - - Addition & Subtraction Addition & Subtraction - - - When subtracting, it is important to remember your integer rules integer rules (ex ex) 4 (-3) = 4 + 3 = 7

37. Polynomials Polynomials Polynomials Polynomials Polynomial Operations Polynomial Operations - - Addition & Subtraction Addition & Subtraction - - - When subtracting, it is important to remember your integer rules integer rules (ex ex) 5 (8-2) = 5 8 + 2 = -3 + 2 = -1 Check: 5 (8-2) = 5 (6) = 5 + (-6) = -1

38. Polynomials Polynomials Polynomials Polynomials Polynomial Operations Polynomial Operations - - Addition & Subtraction Addition & Subtraction - - Remember all of the positives (+) being subtracted change to negatives (-) and all the negatives (-) being subtracted change to positives (+)

39. Mental Math Mental Math Mental Math Mental Math Find the perimeter of the following shape. Please show ALL your steps. 1x + 6 x + 7 1x + 6 x 2x + 1 2x + 1 3x + 2

40. Polynomials Polynomials Polynomials Polynomials Polynomial Operations Polynomial Operations - - Addition & Subtraction Addition & Subtraction - - Subtraction Integer Rule Practice One Subtraction Integer Rule Practice One -(2x+6) = -2x - 6

41. Polynomials Polynomials Polynomials Polynomials Polynomial Operations Polynomial Operations - - Addition & Subtraction Addition & Subtraction - - Subtraction Integer Rule Practice One Subtraction Integer Rule Practice One -(3x+1) = -3x - 1

42. Polynomials Polynomials Polynomials Polynomials Polynomial Operations Polynomial Operations - - Addition & Subtraction Addition & Subtraction - - Subtraction Integer Rule Practice One Subtraction Integer Rule Practice One -(-4a2+2ab + 5b -9) = 4a2 - 2ab - 5b +9

43. Polynomials Polynomials Polynomials Polynomials Polynomial Operations Polynomial Operations - - Addition & Subtraction Addition & Subtraction - - - Like addition, drop brackets and group like terms (ex ex) (-2a2 + a -1) (a2 - 3a + 2) -2a2 + a -1 - a2 + 3a 2 -2a2 - a2 + a + 3a 1 2 -3a2 + 4a - 3

44. Polynomials Polynomials Polynomials Polynomials Polynomial Operations Polynomial Operations - - Addition & Subtraction Addition & Subtraction - - Addition Practice One Addition Practice One (4m2 + 4m -5) + (2m2 2m + 1)

45. 10.02.2019 Agenda Number Sense Routine Introduction Video Cornell Notes Topic- Arithmetic Operations on polynomials E.Q.- How do I perform arithmetic operations on polynomials? Elbow Partner Activity Ticket out the Door Number Sense Routine Simplest form: Simplest form: 16 62 16 62 15 20 32 24 15 20 32 24

46. Mental Math Mental Math Mental Math Mental Math Complete the following addition and subtraction problems, please show ALL of your steps: (2x2- 4y + 2y2) - (8x2- 5y + 7y2) (6a2- 7ab + 12b2) + (13a2) + (5ab + 2b2)

47. Polynomials Polynomials Polynomials Polynomials Polynomial Operations Polynomial Operations - - Addition & Subtraction Addition & Subtraction - - Addition Practice Addition Practice (4m2 + 4m -5) + (2m2 2m + 1)

48. Polynomials Polynomials Polynomials Polynomials Polynomial Operations Polynomial Operations - - Addition & Subtraction Addition & Subtraction - - Subtraction Practice Subtraction Practice (1- 3r + r2) - (4r + 5 3r2)

49. Polynomials Polynomials Polynomials Polynomials Textbook Questions Textbook Questions Addition Addition Pg 228-229 Qs 3, 6, 8(a,c,e,g), 10(i, iii), 15(a,b) Subtraction Subtraction Pg 235-236 Qs 7(a,b), 8(a,c,e,g), 13(a,b), 15(a,b)

50. Mental Math Mental Math Mental Math Mental Math Complete the following addition and subtraction problems, please show ALL of your steps: (2k2- 3k + 2) + (-3k2- 3k + 2) (3x2- 2x + 3) - (2x2 + 4)

Load More ...