Ginzburg Landau phenomenological Theory
The Ginzburg-Landau phenomenological theory explains superconductivity and superfluidity as distinct thermodynamic phases. It focuses on phase transitions characterized by singularities in specific heat at the transition temperature. Derived from BCS theory, it quantifies condensation energy, emphas
1 views • 38 slides
Understanding Temperature Effects on Donor and Acceptor Ionization in Semiconductors
Temperature plays a crucial role in the ionization of donor and acceptor atoms in semiconductors. In N-type semiconductors, the Fermi level lies below the conduction band, while in P-type semiconductors it lies above the valence band, with the position depending on temperature and impurity atoms. Do
1 views • 13 slides
Understanding the Impact of Temperature on Fermi Level in Semiconductors
The Fermi level plays a crucial role in determining the behavior of electrons in semiconductors at different temperatures. As temperature increases, the Fermi level shifts, affecting the generation of free electrons and holes in the valence and conduction bands. In intrinsic semiconductors, electron
2 views • 53 slides
Understanding Density Functional Theory in Chemistry
Density Functional Theory (DFT) plays a crucial role in chemistry by uniquely determining molecular properties based on electron density. The Hohenberg-Kohn Theorem establishes the foundation, with the goal of finding an exact energy functional expressed in terms of density. Various concepts like th
0 views • 19 slides
Experimental Reconstruction of Primary Hot Fragment in Heavy Ion Collisions
Investigation into primary hot fragment reconstruction at Fermi energy heavy ion collisions, utilizing experimental data and simulations to reconstruct excitation energy, mass, and charge of primary fragments. Techniques like kinematical focusing and isotope identification were employed, with a focu
0 views • 36 slides
Investigation of QGP Formation at Fermi Lab CO Collider
Investigation led by L. Gutay at Purdue University, in collaboration with other researchers, presented evidence for de-confinement in high-energy collisions. The E-735 experiment at 1.8 TeV utilized advanced detectors and percolation theory to analyze multiparticle production, showcasing a potential
3 views • 20 slides
Exploring Nuclear Equations and Beta Decay in Physics
Understanding the development of scientific theories like beta decay and nuclear equations through the work of Enrico Fermi and Chien-Shiung Wu. Discover the collaborative nature of scientific progress, where experiments validate theories and correct errors. Scientists worldwide, including George Ga
0 views • 7 slides
Linking Soft-Ray Pulsar and Fermi LAT Pulsar Populations
This research presentation explores the connection between the soft-ray pulsar population and the Fermi LAT pulsar population, focusing on observational data and methodologies for increasing the sample size to enhance our understanding of high-energy pulsars. The study outlines the identification pr
0 views • 13 slides
Introduction to Reactor Physics and Nuclear Fission
Explore the fundamentals of reactor physics, neutron interaction, and nuclear fission in this virtual training course on criticality safety management. Delve into the history of nuclear fission, symbolisms for atoms, and the significance of critical reactors like Fermi's Chicago Pile. Gain insights
0 views • 73 slides
Understanding Silicon Detector Technology
Silicon is a remarkable material with low energy requirements for creating e-hole pairs, long mean free paths, high mobility for fast charge collection, and well-developed technology for fine lithography. Silicon detectors operate based on carrier band diagrams, density of states, and Fermi-Dirac di
0 views • 21 slides
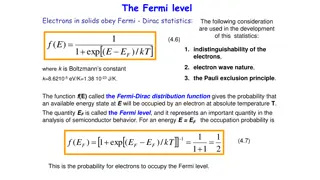
Understanding Fermi-Dirac Statistics in Solids
Electrons in solids obey Fermi-Dirac statistics, governed by the Fermi-Dirac distribution function. This function describes the probability of electron occupation in available energy states, with the Fermi level representing a crucial parameter in analyzing semiconductor behavior. At different tempe
0 views • 9 slides
Understanding Fermi Liquid Theory in Interacting Fermion Systems
Fermi liquid theory, also known as Landau-Fermi liquid theory, is a theoretical model that describes the normal state of metals at low temperatures. Introduced by Landau and further developed by Abrikosov and Khalatnikov, this theory explains the similarities and differences between interacting ferm
0 views • 23 slides
Understanding Band Population in Semiconductors
Exploring the concepts of electron and hole population in semiconductor bands through the Fermi-Dirac function, density of states, and Fermi energy. Learn how the Fermi function influences carrier concentration, the difference between metals and semiconductors in band structure, and the behavior of
0 views • 14 slides
Fermi Problems and Estimation Techniques in Science
Understand Enrico Fermi's approach to problem-solving through estimation in science as demonstrated by Fermi Problems. These problems involve making educated guesses to reach approximate answers, fostering creativity, critical thinking, and estimation skills. Explore the application of Fermi Problem
0 views • 23 slides
Fun Fermi Estimation Quiz Questions
Test your estimation skills with these quirky Fermi estimation quiz questions like guessing the number of black cabs in London or the length of toilet roll used worldwide daily. Can you make accurate guesses on topics ranging from tennis balls at Wimbledon to burgers from a cow?
0 views • 7 slides
The Fascinating Life and Work of Physicist Enrico Fermi
Enrico Fermi, a renowned physicist, made significant contributions to the field of quantum mechanics and nuclear physics. Born in Rome in 1901, he went on to receive the Nobel Prize in 1938 for his work on slow neutrons. Fermi's innovative approach as a theorist and experimenter set him apart in the
0 views • 16 slides
Understanding Insulators and Energy Bands in Materials
Explore the concept of insulators and energy bands in materials, focusing on the forbidden gap, Fermi energy levels, and the classification of solids based on electrical conductivity. Learn about the role of insulators, the energy gap in insulators, and examples of insulating materials like rubber a
0 views • 48 slides
Enrico Fermi's Lectures in South America: 1934 Overview
In 1934, Enrico Fermi embarked on a lecture tour in Argentina, Brazil, and Uruguay, delivering insightful talks on nuclear physics. Despite language barriers, his lectures attracted large audiences and covered groundbreaking topics such as beta decay theory and neutron-induced artificial radioactivi
0 views • 9 slides
Understanding Extrinsic Semiconductors: Fermi Level and Doping Effects
Extrinsic semiconductors play a crucial role in modern electronics by allowing controlled addition of impurities to tailor conductivity. The Fermi level in extrinsic semiconductors shifts based on the number of electrons and holes in the conduction and valence bands, influencing conductivity. Doping
0 views • 14 slides
Exploring Cosmic Ray Sources Using Gamma-Ray Emission Data
This study focuses on investigating ultrahigh energy cosmic ray (UHECR) sources by analyzing extragalactic diffuse gamma-ray emission data. Techniques such as examining UHECR mass composition and arrival directions, as well as studying interactions with cosmic microwave and extragalactic background
0 views • 16 slides
Quantum Statistics and Applications in Fermi Gases
Exploring the fascinating world of quantum statistics and applications in degenerate Fermi gases, electrons in metals, neutron stars, and 3He. Dive into concepts like Fermi energy, particle behavior in boxes, counting states, and more at varying temperatures. Understand the comparison between free e
0 views • 19 slides
EEE Runs Update: Statistics and Performance Summary as of June 3, 2019
Total tracks acquired, days of data taking, candidate tracks per day, performance metrics, and duty cycle information for the EEE telescope network up to Run 5, as reported by Fabrizio Coccetti at Centro Fermi Museo Storico della Fisica e Centro Studi e Ricerche Enrico Fermi in Rome, Italy. The netw
0 views • 16 slides
EVMS Training CAM eToolBox and Change Control Tools Overview
Explore the essentials of EVMS Training with a focus on CAM eToolBox and Change Control Tools, presented by Mohammed Elrafih. Learn about obtaining a Fermi Services account, accessing Fermi Systems, and essential tools for project monitoring and control. Gain insights into generating reports, managi
0 views • 43 slides
Financial Overview of Fermilab - Insights from Fiscal Year 2015
Fermilab, operated by Fermi Research Alliance LLC, receives over 99% of its funding from the Federal Government, mainly through the Office of High Energy Physics. The FY2015 financial results show total revenue of $382,272,583 with expenses covering various categories like salaries, subcontracts, an
0 views • 7 slides
Fixed Point Collisions in Luttinger Semimetals and Field Theories
Exploring fixed point collisions and tensorial order parameters in Luttinger semimetals and various field theories, such as chiral symmetry breaking in QED and Interacting O(N) field theory. The research delves into the condensed matter motivation behind quadratic band touching and the Luttinger Ham
0 views • 39 slides
Grand Canonical Ensemble in Statistical Mechanics: Fermi-Dirac Distribution
Exploring the Fermi-Dirac distribution function and the Bose-Einstein distribution in the context of the grand canonical ensemble for non-interacting quantum particles. The lecture delves into the impact of particle spin on energy spectra, enumeration of possible states, self-consistent determinatio
0 views • 33 slides
Nanoelectronics Lecture Notes Highlights
The image highlights key concepts from nanoelectronics lecture notes, covering topics such as density of states in different dimensions, classical and quantum statistics, Fermi distribution, and carrier concentration in materials. The visuals aid in understanding complex theoretical aspects of nanoe
0 views • 14 slides
Understanding Semiconductors: Types, Properties, and Differences
Semiconductors bridge the gap between insulators and conductors, exhibiting varying levels of electrical conductivity based on temperature. This tutorial covers intrinsic semiconductors, differences between silicon and germanium, and the essential properties of semiconductors. Learn about the roles
0 views • 25 slides
Insights into Quasiparticles in Normal and Superfluid Fermi Liquids
This content discusses quasiparticles in normal and superfluid Fermi liquids, covering topics such as the Landau Fermi-liquid idea, BCS theory, Bogoliubov-de Gennes approach, Majorana fermions, conservation quantities in liquids, consequences of conservation for response functions, and applications
0 views • 23 slides
Development of Quantum Statistics in Quantum Mechanics
The development of quantum statistics plays a crucial role in understanding systems with a large number of identical particles. Symmetric and anti-symmetric wave functions are key concepts in quantum statistics, leading to the formulation of Bose-Einstein Statistics for bosons and Fermi-Dirac Statis
1 views • 15 slides
Insights into Semiconductor Behavior and Mobility Influence
Understanding the intrinsic and extrinsic behavior of semiconductors with respect to temperature dependence, carrier concentration, and mobility. Exploring the impact of temperature and doping on carrier mobility through lattice and impurity scattering effects. Examining the invariance of Fermi leve
0 views • 8 slides
Understanding the Location of Gamma-Ray Emission Zones in Blazars
This research explores the determination of the GeV emitting zone in fast, bright blazars through studies conducted during the AAS Meeting in January 2014. The focus is on locating the gamma-ray emission zone within blazars and investigating the critical differences between various energy scales lik
0 views • 23 slides
Understanding Signatures, Commitments, and Zero-Knowledge in Lattice Problems
Explore the intricacies of lattice problems such as Learning With Errors (LWE) and Short Integer Solution (SIS), and their relation to the Knapsack Problem. Delve into the hardness of these problems and their applications in building secure cryptographic schemes based on polynomial rings and lattice
0 views • 44 slides
Addressing the Gas Crisis in Rome: Solutions and Strategies
The gas crisis at Centro Fermi in Rome on 4th July 2019 highlighted the need for reducing harmful gas emissions. Regulations have been in place since the 1990s to ban gases with high ozone depletion power. The European Community is progressively banning gases with high global warming power, leading
0 views • 8 slides
Advanced LIGO and Short Gamma-Ray Burst Search Overview
Advanced LIGO is focused on achieving a lower noise level and higher sensitivity to detect gravitational waves. The search involves analyzing data from the 1st Advanced LIGO Science Run, collaboration with Fermi, and seeking coincident detections of gravitational waves and gamma-ray bursts, particul
0 views • 13 slides
Magnet Development Program Test Plan and Status Meeting Overview
The Magnet Development Program Test Plan and Status Meeting held on March 18, 2020, at Fermi National Accelerator Laboratory focused on studying the effects of increased pre-load and altered end-support on magnet performance. The goals included measuring ramp rate, quench current, field quality, and
0 views • 12 slides
Theory of Computation: Decidability and Encoding in CSE 105 Class
Explore the concepts of decidability, encoding, and computational problems in CSE 105 Theory of Computation class. Learn about decision problems, encodings for Turing Machines, framing problems as languages of strings, and examples of computational problems and their encodings. Gain insights into th
0 views • 26 slides
Some Fermi News from Lab Status Meetings - Updates and Reports - January 2017
Updates from the recent TD/MSD/M&A Group Meeting on January 26, 2017, covering incidents, accelerator operations, future schedules, and important updates on various projects like MicroBooNE, MINERvA, NOvA, SeaQuest, g-2, LZ, and more. The report also includes notes on computing operations, LCLS-II d
0 views • 7 slides
Insights into NP-Hard Problems in Molecular Biology and Genetics
Understanding the complexity of NP-Hard Problems arising in molecular biology and genetics is crucial. These problems involve genome sequencing, global alignment of multiple genomes, identifying relations through genome comparison, discovering dysregulated pathways in human diseases, and finding spe
0 views • 24 slides
Understanding P, NP, NP-Hard, NP-Complete Problems and Amortized Analysis
This comprehensive study covers P, NP, NP-Hard, NP-Complete Problems, and Amortized Analysis, including examples and concepts like Reduction, Vertex Cover, Max-Clique, 3-SAT, and Hamiltonian Cycle. It delves into Polynomial versus Non-Polynomial problems, outlining the difficulties and unsolvability
0 views • 32 slides