Grand Canonical Ensemble in Statistical Mechanics: Fermi-Dirac Distribution
Exploring the Fermi-Dirac distribution function and the Bose-Einstein distribution in the context of the grand canonical ensemble for non-interacting quantum particles. The lecture delves into the impact of particle spin on energy spectra, enumeration of possible states, self-consistent determinations, isotropic properties, and low-temperature behaviors. Mathematical derivations and concepts related to quantum statistics are discussed in detail.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
PHY 770 -- Statistical Mechanics 12:00* -1:45 PM TR Olin 107 Instructor: Natalie Holzwarth (Olin 300) Course Webpage: http://www.wfu.edu/~natalie/s14phy770 Lecture 14 Chap. 6 Grand canonical ensemble Fermi-Dirac distribution function Bose-Einstein distribution *Partial make-up lecture -- early start time 3/18/2014 PHY 770 Spring 2014 -- Lecture 14 1
3/18/2014 PHY 770 Spring 2014 -- Lecture 14 2
Reminder: Please think about the subject of your computational project due next week. Suggestions available upon request. 3/18/2014 PHY 770 Spring 2014 -- Lecture 14 3
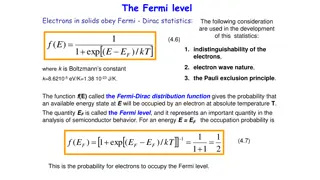
Examples of grand canonical ensembles ideal (non-interacting) quantum particles in a cube of length L with periodic boundary conditions -- Fermi-Dirac case In the absence of a magnetic field, the particle spin does not effect the energy spectrum, and only effects the enumeration of possible states spin (g) g 1 p p ( ) ( ) n = = ( ) F DT V H N , , Tr Z e e p p i i = 0 n i i ( ) g p ( ) = + 1 e p i i ( ) g p ( ) ( ) = + , , ln 1 T V k T e p i FD B i ( ) p ( ) + = ln 1 k Tg e p i B i 3/18/2014 PHY 770 Spring 2014 -- Lecture 14 4
Fermi-Dirac case -- continued ( ) p ( ) ( ) = + , , ln 1 T V k Tg e p i FD B i Self-consistent determination of : = g p = N FD ( ) + 1 e p i , T V i Recall: 3 L V p = 3 2 p 4 since is isotropic in d p p dp ( ) p 3 2 2 0 3/18/2014 PHY 770 Spring 2014 -- Lecture 14 5
Fermi-Dirac case -- continued g p = = N FD ( ) + 1 T V e p i , i Vg z = = 2 Let : 4 z e N p dp ( ) 2 3 2 + /2 p m e z 2 0 2 p Vg = 2 Let ( ) z x N f 3/2 T 3 T 2 m 2 mk T B 4 z ( ) + 1 2 + = 2 x Here: ( ) z ln 1 1 f dx x ze 5/2 5/2 0 = 0 ( ) z 4 d f z z ( ) + 1 = = 2 5/2 dz ( ) z 1 f dx x z 3/2 3/2 2 + x e z 0 = 0 3/18/2014 PHY 770 Spring 2014 -- Lecture 14 6
Fermi-Dirac case -- continued Low temperature behavior: for 0 z for 0 T 2 m Vg = 2 4 N p dp ( ) 3 2 0 3/2 N V 2 2 6 = = ( 0) T F 2 m g 3/18/2014 PHY 770 Spring 2014 -- Lecture 14 7
Fermi-Dirac case -- continued Keeping more terms in low temperature expansion: = + 2 2 k T + ( ) T 1 ... B F 12 F 2 2 3 5 5 12 k T + 1 .. . U H N B F F 2 B 2 N k C T V 2 F 3/18/2014 PHY 770 Spring 2014 -- Lecture 14 8
Fermi-Dirac case -- continued Behavior of occupancy parameter: g n e + gz = p ( ) + 1 e z p p 3/18/2014 PHY 770 Spring 2014 -- Lecture 14 9
Examples of grand canonical ensembles ideal (non-interacting) quantum particles in a cube of length L with periodic boundary conditions -- Bose-Einstein case (assumed to have spin 0) p p ( ) ( ) n = = ( ) BET V H N , , Tr Z e e p p i i = 0 n i i 1 p = ( ) 1 e p i i 1 p ( ) = , , ln T V k T BE B ( ) 1 e p i i ( ) p ( ) = ln 1 k T e p i B i 3/18/2014 PHY 770 Spring 2014 -- Lecture 14 10
Bose-Einstein case Fermi-Dirac case p g p p ( ) 1 ( ) n = BET V , , Z e p p ( ) p p i i ( ) n = FDT V , , Z e p p i i = 0 n i = 0 n i i i 1 ( ) p g p ( ) = + = 1 e p i ( ) 1 e ( p i i i ( ) ) p ( ) ( ) ( ) ( ) + , , = ln 1 T V k Tg e , , = ln 1 p T V k T e p i i F D B B E B i i ( FD T V gk TV k TV ) ( ) z ( ) ( ) ( ) z , , = f B , , = ln 1 T V k T z g B 5/2 3 T 5/2 B E B 3 T 4 ( ) z 4 2 + 2 x ( ) z where ln(1 ) f x dx ze 2 2 x where ln(1 ) g x dx ze 5/2 5/2 0 0 2 2 mk T 2 2 mk T T T B B 3/18/2014 PHY 770 Spring 2014 -- Lecture 14 11
2 2 mk T Bose-Einstein case Fermi-Dirac case T B 4 2 ) ze 4 ( ) z 2 + 2 ( ) z x ln(1 ) f x dx ze 2 x ln(1 g x dx 5/2 5/2 0 0 4 z 4 z ( ) z 2 ( ) z f x dx 2 g x dx 3/2 2 + x e z 3/2 2 x e z 0 0 = gkT kT V kT ( ) z = = ( ) ( ) z P f FD V = + ln 1 P z g BE 5/2 3 T 5/2 3 T V N V gkTf N V 1 V z kTg ( ) z = ( ) z = + 3/2 3 T 3/2 3 T 1 z 3/18/2014 PHY 770 Spring 2014 -- Lecture 14 12
Case of Bose particles Non-interacting spin 0 particles of mass m at low T moving in 3-dimensions in large box of volume V=L3: Assume that each state ek is singly occupied. 1 = = e k k N n ( ) k 1 k ( ) + mL + 2 2 x 2 y 2 z h n n n = 3 , 2 , 1 = , , n n n , , n n n x y z 2 8 x y z 2 2 k In the limit , L k 2 m 3 L k = ( 3 ) d k d g B 2 / 3 2 2 V m ( = ) g B 4 2 2 3/18/2014 PHY 770 Spring 2014 -- Lecture 14 13
Case of Bose particles at low T n N k 1 k = = + n ( ) 0 k 1 e k 0 1 = + ( ) N n d g ( ) 0 B 1 e 0 Note that for low T consistent a solutions exists such that N n 0 1 1 1 = = assuming small n ( ) 0 1 1 1 e kT In this case, N 3/18/2014 PHY 770 Spring 2014 -- Lecture 14 14
Critical temperature for Bose condensation: 0 + = 1 ( ) N n d g ( ) B 1 e 0 condensate normal state 1 = ( ) If , there is no "condensate" N d g ( ) B 1 e 0 The temperature at which the above equality is satisfied is called the Einstein condensation temperature Approximate value of : E T 0 3/2 2 2.612 4 2 . T E 3/2 3/2 2 2 1 1 mkT V m V = N d dx x E 2 2 2 2 x 4 1 4 1 e e E 0 2/3 2 / 2 mkT V N V = N kT E E 2 2 2.612 m 3/18/2014 PHY 770 Spring 2014 -- Lecture 14 15
Case of Bose particles at low T n N k 1 k = = + n ( ) 0 k 1 e k 0 1 = + ( ) N n d g ( ) 0 B 1 e 0 Note that for low T consistent a solutions exists such that N n 0 1 1 1 = = assuming small n ( ) 0 1 1 1 e kT In this case, N 3/18/2014 PHY 770 Spring 2014 -- Lecture 14 16
Critical temperature for Bose condensation: 0 + = 1 ( ) N n d g ( ) B 1 e 0 condensate normal state 1 = If ( ) there , is no " condensate " N d g ( ) B 1 e 0 temperatu The at which t re above he equality satisfied is is Einstein the called condensati on tempera ture T . E Approximat value e : / 3 2 / 3 2 2 1 2 1 V m 2 V mkT = E N d dx x 2 2 2 x 4 1 4 1 e e E 0 0 / 3 2 3 / 2 2 2 / 2 V mkT N . 2 V = . 2 612 E N kT E 2 2 4 2 612 m 3/18/2014 PHY 770 Spring 2014 -- Lecture 14 17
Summary: 1/2 2 2 mkT Define 1 z e T The Landau potential for the Bose system can be written: ( ) 4 kT V 2 = + 2 x ( , , ) T V ln(1 ) ln 1 kT z dx x ze BE 3 T / L T 4 z V z = = + 2 N dx x B 3 T 2 1 z x e z / L T 0 n 3/18/2014 PHY 770 Spring 2014 -- Lecture 14 18
Some convenient integrals 4 ) ( z g ( 1 ) n z = n 0 2 = 2 x ln dx x ze / 5 2 / 5 2 n 1 n 4 z d z = n 0 = = 2 ( ) ( ) g z dx x z g z / 3 2 / 5 2 / 3 2 2 dz n x e z 1 g3/2(z) g5/2(z) z 3/18/2014 PHY 770 Spring 2014 -- Lecture 14 19
/ 1 2 2 2 mkT z = where 1 n z e 0 T 1 z / L 4 4 V z V z T = = 2 2 ( ) N n dx x g z dx x 0 / 3 2 3 T 3 T 2 2 x x e z e z 0 / L T V = ( ) note that ( ) . 2 612 N n g z g z lim z 0 / 3 2 / 3 2 3 T 1 0 n g3/2(z) z 3/18/2014 PHY 770 Spring 2014 -- Lecture 14 20
Equation for : z V = + ( ) N n g z 0 / 3 2 3 T Define Einstein t V emperature V = = ) 1 ( .612 2 N g / 3 2 3 T 3 T E E V = For , and ( solution a has ) for 1 T T n N N g z z 0 / 3 2 E 3 T V = + For , 1 and ) 1 ( T T z N n g 0 / 3 2 E 3 T / 3 2 3 T n T 0 = = For 1 1 T T E E 3 T N T E 3/18/2014 PHY 770 Spring 2014 -- Lecture 14 21
n0 N T/TE 3/2 3 T n N T T = = 0 1 1 E 3 T E 3/18/2014 PHY 770 Spring 2014 -- Lecture 14 22
http://www.colorado.edu/physics/2000/bec/three_peaks.html 87Rb atoms (~2000 atoms in condensate) 3/18/2014 PHY 770 Spring 2014 -- Lecture 14 23
3/18/2014 PHY 770 Spring 2014 -- Lecture 14 24
Other systems with Bose statistics Thermal distribution of photons -- blackbody radiation: In this case, the number of particles (photons) is not conserved so that =0. 1 e = = = = n k 1 k ck h k 3 L V = 3 2 ( ) d d k ck d 2 3 3 2 c k Distributi on of radiated energy : 3 3 8 V hV = = = E n d d k k 2 3 3 3 h 1 1 c e c e k ( ) 4 5 8 V kT = E ( )3 hc 15 3/18/2014 PHY 770 Spring 2014 -- Lecture 14 25
Blackbody radiation distribution: T3>T2 T2>T1 T1 3/18/2014 PHY 770 Spring 2014 -- Lecture 14 26
Other systems with Bose statistics Thermal distribution of vibrations -- phonons: In this case, the number of particles (phonons) is not conserved so that =0. 1 = n k 1 e k = k fundamenta the solid, Einstein For frequency l vibrates directions 3 in e for all particles. N 1 1 = + 3 E N 1 2 2 kT E e = = 3 C Nk ( ) 2 T 1 e 3/18/2014 PHY 770 Spring 2014 -- Lecture 14 27
Other systems with Bose statistics -- continued Thermal distribution of vibrations -- phonons: 1 = n k 1 e k = k = the be to here fundamenta the solid, Debye For frequency l where , c k c 3 denotes the speed of sound (assumed same in directions ). 3 / T T 3 3 3 V d T x dx D D 0 0 = = 9 E NkT 2 3 x 2 1 1 c e T e D 3/18/2014 PHY 770 Spring 2014 -- Lecture 14 28
Effects of interactions between particles classical case ( ) 1 N N p N ( ) ij r = + + N N i H V T V 2 m = 1 , i i j 1 N 1 ( ) T = = H Canonical partition function : Tr ( , ) T V Z e Q ( ) N N 3 N ! T N V r r d e r where ( , ) T V ... . Q d d 1 2 N N Grand canonical partition func tion : 1 ! N 1 ( ) T ( ) T = = N N ( , ) T V Z Z e Q e ( ) N N 3 N = = 1 1 N N T 3/18/2014 PHY 770 Spring 2014 -- Lecture 14 29
Effects of interactions between particles classical case -- continued ( ) 1 N N ( ) ij r = N V V , i j N V r r d e r ( , ) T V .... Q d d 1 2 N N ( ) 1 N N ( ) ij r V r r r = .... d d d e , i j 1 2 N ( ) r r r r , ... r r .... d d d W 1 2 1 2 N N N ( 1) N N ( ) + r r r , ... ( 1) W f 1 2 N N i j , i j ( ) ) 1 ij r ex p( f V ij 3/18/2014 PHY 770 Spring 2014 -- Lecture 14 30
Effects of interactions between particles classical case -- continued ( ) i r exp( ) 1 f V i j j 12 6 V V 0 ij r r ij ij 3/18/2014 PHY 770 Spring 2014 -- Lecture 14 31
Effects of interactions between particles classical case -- continued 1 N 1 = ( ) N r r d W r , ... r r r ( , ) = Z T V ... . e d d 1 2 1 2 N N N 3 N ! 0 N T ( 1) N N ( ) + r r r , ... ( 1) W f 1 2 N N i j , i j ( ) ) 1 ij r ex p( f V ij Note that: W = ( ) 1 + r r 1 , =1 + + W 12 f 1 2 + 1 2 ( ) ( = 1 )( )( ) , , r r r 1 W 12 f 13 f f 3 1 2 3 23 3/18/2014 PHY 770 Spring 2014 -- Lecture 14 32
Effects of interactions between particles classical case -- continued 1 1 ( , ) = ! In terms of cumulant expansion: ( ) N r r d W r , ... r r r .... Z T V e d d 1 2 1 2 N N N 3 N N = 0 N T 1 1 ( ) r r d U r , .. r r r ( , ) =exp Z T V .... . e d d 1 2 1 2 3 ! = 0 T Typical cluster functions: U W = = ( ) ( r r ( ) 1 W r r 1 1 1 ) ( ) ( ) 1 r ( ) 2 r r r , , U W W 2 1 2 2 1 2 1 1 3/18/2014 PHY 770 Spring 2014 -- Lecture 14 33