Quantum Statistics and Applications in Fermi Gases

Exploring the fascinating world of quantum statistics and applications in degenerate Fermi gases, electrons in metals, neutron stars, and 3He. Dive into concepts like Fermi energy, particle behavior in boxes, counting states, and more at varying temperatures. Understand the comparison between free electron models and experimental measurements in different metals.

• Quantum
• Statistics
• Fermi Gases
• Applications
• Metals

alie_156 Follow

Uploaded on Sep 14, 2024 | 0 Views

Download Presentation

Please find below an Image/Link to download the presentation.

The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.

Presentation Transcript

1. Week 12 Quantum statistics, applications

2. Degenerate Fermi gas Electrons in metal Neutron star 3He ? + ? ? + ?? From Wikipedia

3. Degenerate Fermi gases Very low temperature ??= ?3, ? = 2???? Boltzmann statistics breaks down when ? ? ?? or 3?/? ?. For electrons in metal, ? = 9.109 10 31kg, at ? = 300K, 1/3 ? ? ? = 4.3 nm, = 0.2 nm

4. Zero temperature, Fermi particles in a box ?FD Fermi energy ?? ?(? = 0) Computing ?? 1 0 ? ? = ?? ??=2? ??, ?, ??= ? = 1,2,3, ? = ?3 ?2 2?= 2 2+ ?? 2+ ?? 2 ? = 8??2?? ?

5. Particle in a box, fixed boundary condition vs periodic boundary condition standing wave travelling wave ??2???/? sin 2??/?? ? = 1 ? = 1 ? = 0 0 ?

6. Counting the number of states ??= 2?max 8??2 2 Each triplet of positive integers (??,??,??) represents a pair of definite-energy electron states (one with each spin orientation). The set of all independent states fills the positive octant of ?-space. ??~5 eV, ?~10 31kg, ?~0.1m, ~10 34J s ? = 2 volume of eighth sphere = 2 1 = ??max 3 8 4 3 3??max 3 ?max~108

7. Fermi energy, total energy 2/3 ??= 2 3? ?? 1/8 of total solid angle is 1 8 4? 8? Total energy ? 2 ? 2 ?max ???2sin??(?) ? = 2 ? ??,??,?? = 2 ? ? ?????????= 2 ?? ?? 0 ??,??,?? ? 2 8??2 0 0 ?max ?4?? =? 2?max 5 2 2?max 8??2 =3 2 40??2=3 ??max 3 = 5??? 5 0 In spherical coordinates (?,?,?), the infinitesimal volume element is ?? ??? ?sin??? .

8. Fermi gas at ? = 0, pressure, bulk modulus 2/3 ??= 2 3? ?? 8? Total energy ? =3 5??? Pressure 2 3 3 5? 2 ?? ?? = ? 3? ? 3 =2??? =2 ? ? ? 2 ? = ?? 8? 5? 3 ?,? Bulk modulus (inverse of compressibility) ? =1 ?? ?? =10 ? ? ?= ? 9 ?

9. Compare free electron model with experiments Metal Free electron B 23.9 9.23 3.19 2.28 1.54 63.8 34.5 228 Measured B Bulk modulus ? = 1 ?? ?? Li Na K Rb Cs Cu Ag Al 11.5 6.42 2.81 1.92 1.43 134.3 99.9 76.0 ? 1 atm 105 N/m2 From Table 2.2, Ashcroft/Mermin, Solid State Physics , page 39. Units for B is in 109 N/m2.

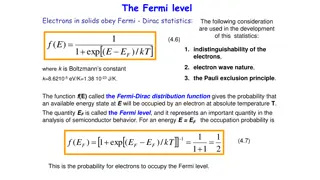
10. Fermi distribution, small T ?FD 1 ?(? ?)/(??)+ 1 at 300K ?? is 0.026 eV ?FD= ? 0 1 ?? ? ? Additional energy when ? > 0 (number of affected electrons) (energy acquired by each) ??? (??) ? ??2 ?? 5???+?2 ??2 ?? ?2??2? 2?? ? 3 ?? ?? 4? , ??= ?

11. Density of states 2 8??2 2 8??2 2 1 8??2?2, ? = ? = ?, ?? = 2 ??? ?max ?? 3/2 8??2 2 ? 2 ? ? ?2?? = ? = ? ? ? ?? (? = 0) 0 0 Density of states - number of states with energy (?,? + ??) is ? ? ?? ? ? = ?0 ? 3/2 ? ? =? 8? 2 ? ? 2

12. Particle number and energy in terms of ?(?) ?(?) ? ? ? = ? ? ?FD? ?? = ??+1?? ? 0 0 ? ?(?) ? ? ? = ?? ? ?FD? ?? = ??+1?? ? 0 0 ? ? ??

13. Chemical potential 2 = 1 ?2 ?(?) ?? ?? ?? + 12

14. Sommerfeld expansion Step 1) integration by parts 3 2 ?FD(?) 3 2 ? ?FD ? 2 3?0? +2 ? = 3?0 0 ?? ?? 0 ?? ? ??? 1 ??+12, ? =? ? = ?? ?? ?? ? ?FD/?? is peaked at ? and decays very fast to zero away from it.

15. Sommerfeld expansion Step 2) make a change of integration variable to ? =? ? . Step 3) Taylor expanding around ? for the factor ?3/2 ??, extend lower bound to 3 2 3 2 ?2? ??2 ?? ?? ?=?+1 8? 1/2? ?2+ ?3/2= ?3/2+ ? ? =?3/2+3 2? ?2 ?=?+ 2?1/2? ? +3

16. Step 4) using integrals 1 if ? = 0 0 if ? = 1 ?2 3 if ? = 2 + ???? ??+ 12?? = 3/2 + ??2 ??2 3/2?1/2+ ? ?? ? = 8 ?? Step 5) cancel ?, solve for ? we get (for small ?) 2 = 1 ?2 ?(?) ?? ?? ?? + 12

17. Sommerfeld expansion formula + ? 6 ? ? ?? +?2 ??2? ? +7?4 ?? ? 360??4? ? + ? ? ? ?FD? ?? = 6 Same steps for ? leads to 5???+?2 ??2 ?? ? =3 4? +

18. Tutorial problems

19. Tutorial problem 7.28