Understanding the Law of Sines for Solving Oblique Triangles
Explore the Law of Sines to solve oblique triangles efficiently, covering cases like AAS, ASA, and SSA. Learn how to find areas of triangles and apply the Law of Sines to real-life scenarios. Discover the significance of knowing at least one side and two other measures of a triangle.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
Objectives Use the Law of Sines to solve oblique triangles (AAS or ASA). Use the Law of Sines to solve oblique triangles (SSA). Find the areas of oblique triangles. Use the Law of Sines to model and solve real-life problems.
Plan for the day When to use law of sines Applying the law of sines
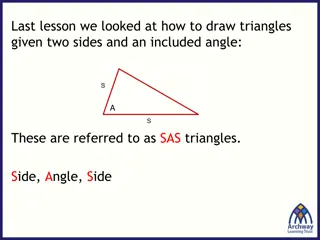
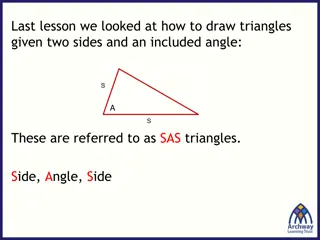
Introduction In this section, we will solve oblique triangles triangles that have no right angles. As standard notation, the angles of a triangle are labeled A, B, and C, and their opposite sides are labeled a, b, and c. To solve an oblique triangle, we need to know the measure of at least one side and any two other measures of the triangle either two sides, two angles, or one angle and one side.
Introduction This breaks down into the following four cases: 1. Two angles and any side (AAS or ASA) 2. 3. Two sides and an angle opposite one of them (SSA) Three sides (SSS) 4. Two sides and their included angle (SAS) The first two cases can be solved using the Law of Sines, whereas the last two cases require the Law of Cosines.
Introduction The Law of Sines can also be written in the reciprocal form: .
Given Two Angles and One Side AAS For the triangle below m C = 102 , m B = 29 , and b = 28 feet. Find the remaining angle and sides. 49 The third angle of the triangle is: m A = 180 m B m C m A = 180 29 102 m A = 49
49 28 ? sin29 = sin49 28 ? sin29 = 28 * sin 49 = a * sin 29 sin 29 sin102 sin 29 28 * sin 102 = c * sin 29 sin 29 a 43.59 ft. sin 29 c 56.49 ft.
Law of Sines B For non-right triangles 67 45 a c Law of sines a sin b c = = 70 43 sin sin A B C A C b Try this: m A = 43 , m B = 67 , c = 45 mm The third angle of the triangle is: m C = 180 m A m B m C = 180 43 67 m C = 70
B 45 67 a c ? ? ? 70 43 sin?= sin?= A C sin? b ? 45 sin67 = sin70 ? 45 sin43 = sin70 b * sin 70 = 45 * sin 67 sin 70 sin 70 a * sin 70 = 45 * sin 43 sin 70 sin 70 b 44.08 mm a 32.66 mm
Example Single-Solution CaseSSA For the triangle below, a = 22 inches, b = 12 inches, and m A = 42 . Find the remaining side and angles. ? ? ? sin?= sin?= sin? 22 12 sin42 = sin? sin B = some number Push 2nd SIN Push 2nd ( ) for ANS ??? 1 (ANS) ENTER 22 * sin B = sin (42) * 12 22 22 m B 21.40
Law of Sines 116.6 21.4 The third angle of the triangle is: m C = 180 m A m B m C = 180 42 21.4 m C = 116.6 22 * sin 116.6 = c * sin (42) sin 42 22 ? sin42 = sin 42 sin116.6 c 29.40
Objectives: Use the Law of Cosines to solve oblique triangles (SSS or SAS). Use the Law of Cosines to model and solve real-life problems.
Warm-up: Solve for side a in ABC when m A = 29 , m B = 62 and c = 11.5 B The third angle of the triangle is: m C = 180 m A m B m C = 180 29 62 m C = 89 11.5 62 c a 89 29 C b A 11.5 sin89= ? 11.5 * sin 29 = a * sin 89 sin 89 sin29 sin 89 11.5 sin89= ? a 5.58 b 10.16 sin62
Introduction Four cases. 1. Two angles and any side (AAS or ASA) 2. 3. Two sides and an angle opposite one of them (SSA) Three sides (SSS) 4. Two sides and their included angle (SAS) The first two cases can be solved using the Law of Sines, whereas the last two cases require the Law of Cosines.
Law of Sines For non right triangles Law of sines B ? ? ? sin?= sin?= a c sin? A b C
Law of Cosines: Introduction Two cases remain in the list of conditions needed to solve an oblique triangle SSS and SAS. If you are given three sides (SSS), or two sides and their included angle (SAS), none of the ratios in the Law of Sines would be complete. In such cases, you can use the Law of Cosines.
Law of Cosines B Side, Angle, Side c a A C b = + 2 2 2 2 a b c bcCos 2 A = + 2 2 2 b a c acCos B = + 2 2 2 2 c a b abCos C
Try these: Solve ABC. Round angle measures to the nearest degree and side measures to the nearest tenth. 1. m A = 52o b = 6 c = 8 B a2 = b2 + c2 2bc cos A 8 c a a2 = 62 + 82 2(6)(8) cos 52 52 a2 = 36 + 64 96 cos 52 C b 6 A a2 = 100 96 cos 52 a 6.4
? ? ? sin?= sin?= B sin? 8 6.4 48 c 6.4 sin52= 6 a sin? 52 6.4 * sin B = 6 * sin 52 6.4 m B 48 C b 6 A 6.4 sin B = some number Push 2nd SIN Push 2nd ( ) for ANS ??? 1 (ANS) ENTER The third angle of the triangle is: m C = 180 m A m B m C = 180 52 48 m C 80
2. mB = 58o a = 9 c = 14 B b2 = a2 + c2 2ac cos B 14 58 9 c a b2 = 92 + 142 2(9)(14) cos 58 A C b 12.0 b2 = 81 + 196 252 cos 58 b2 = 277 252 cos 58 b 12.0
2. mB = 58 a = 9 c = 14 B 12 9 sin58= 14 58 9 sin? c a 12 * sin A = 9 * sin 58 12 m A 39 12 A C b 12.0 sin C = some number Push 2nd SIN Push 2nd ( ) for ANS ??? 1 (ANS) ENTER The third angle of the triangle is: m C = 180 m A m B m C = 180 39 58 m C 83
Law of Cosines B Side, Side, Side c a A C b
Law of Cosines cos A = ?2+ ?2 ?2 SSS 2?? cos B = ?2+ ?2 ?2 2?? cos C = ?2+ ?2 ?2 2??
Try these: Solve ABC. Round angle measures to the nearest degree. 3. a = 21 b = 16.7 c = 10.3 B 10.3 21 c a cos A = ?2+ ?2 ?2 2?? A C b 16.7 cos A = 16.72+ 10.32 212 2(16.7)(10.3) cos A = 278.89 + 106.09 441 344.02
B cos A = 278.89 + 106.09 441 10.3 344.02 21 c a cos A = 56.02 99 344.02 A C b m A 99 16.7 The third angle of the triangle is: m C = 180 m A m B m C = 180 99 52 21 16.7 sin? sin99= 21 * sin B = 16.7 * sin 99 21 m C 29 21 m B 52
4. a = 19 b = 24.3 c = 21.8 B 21.8 19 c a cos A = ?2+ ?2 ?2 2?? A C b 16.7 cos A = 24.32+ 21.82 192 2(24.3)(21.8) cos A = 590.49 +475.24 361 1059.48
B cos A = 590.49 + 475.24 361 21.8 1059.48 19 c a cos A = 704.73 48 1059.48 A C b m A 48 24.3 The third angle of the triangle is: m C = 180 m A m B m C = 180 48 59 m C 75 19 24.3 sin? sin48= 19 * sin B = 24.3 * sin 48 19 21 m B 59
Applications of the Law of Cosines The pitcher s mound on a women s softball field is 43 feet from home plate and the distance between the bases is 60 feet (The pitcher s mound is not halfway between home plate and second base.) How far is the pitcher s mound from first base?
Solution In triangle HPF, m H = 45 (line HP bisects the right angle at H), f = 43, and p = 60. Using the Law of Cosines for this SAS case, you have h2 = f2 + p2 2fp cos H h2 = 432 + 602 2(43)(60) cos 45 . h2 1800.3 So, the approximate distance from the pitcher s mound to first base is h 42.43 feet.
Applications of the Law of Cosines C The leading edge of each wing of the B-2 Stealth Bomber measures 105.6 feet in length. The angle between the wing's leading edges is 109.05 . What is the wing span (the distance from A to C)? B 105.6 ft A 34
C a 105.6 b 109.05 B 105.6 c A b2 = a2 + c2 2ac cos B b2 = 105.62 + 105.62 2(105.6)(105.6) cos 109.05 b = 172.47 ft
Area of an Oblique Triangle The procedure used to prove the Law of Sines leads to a simple formula for the area of an oblique triangle. Referring to the triangles below, that each triangle has a height of h = b sin A. A is obtuse. A is acute.
Area of a Triangle - SAS SAS you know two sides: b, c and the angle between: A B c a h Remember area of a triangle is 1 2 base height Base = b Height = c sin A Area = 1 2 bc(sinA) C A b Looking at this from all three sides: Area = 1 2 ab(sin C) = 1 2 ac(sin B) = 1 2 bc (sin A)
Example Finding the Area of a Triangular Lot Find the area of a triangular lot having two sides of lengths 90 meters and 52 meters and an included angle of 102 . Solution: Consider a = 90 meters, b = 52 meters, and the included angle C = 102 Then, the area of the triangle is Area = 1 2ab sin C 2(90)(52)(sin102 ) 2289 square meters. 1 =
Herons Area Formula The Law of Cosines can be used to establish the following formula for the area of a triangle. This formula is called Heron s Area Formula after the Greek mathematician Heron (c. 100 B.C.).
Area of a Triangle Law of Cosines Case - SSS B SSS Given all three sides c a h Heron s formula: A C b where s = ? + ? +? 2 = ( )( )( ) A s s a s b s c
1. Given the triangle with three sides of 6, 8, 10, find the area. s = 24 s = 12 s = 6 + 8 +10 2 2 s = ( )( )( ) A s a s b s c A = 12 12 6 12 8 (12 10) A = 12 6 4 2 A = 24 units2
2. Given the triangle with three sides of 12, 15, 21, find the area s = 12 +15 +21 2 )( ( s a s s A = s = 48 s = 24 2 )( ) b s c A = 24 24 12 24 15 (24 21) A = 24 12 9 3 A = 7776 units2