Understanding the Binomial Distribution and Probability Calculations
The binomial distribution involves two possible outcomes, success or failure, in a fixed number of trials with a constant probability of success. Examples and probability-based questions illustrate how to calculate probabilities using the binomial distribution and tree diagrams.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
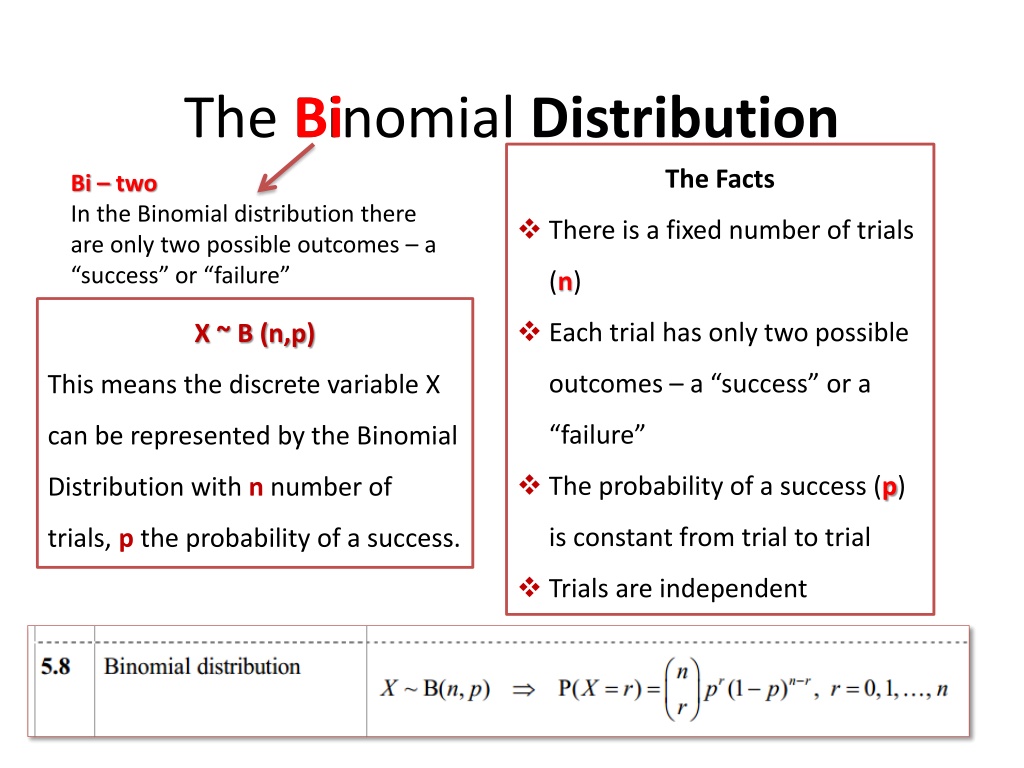
The Binomial Distribution Bi The Facts Bi two In the Binomial distribution there are only two possible outcomes a success or failure There is a fixed number of trials (n) Each trial has only two possible X ~ B (n,p) outcomes a success or a This means the discrete variable X failure can be represented by the Binomial The probability of a success (p) Distribution with n number of is constant from trial to trial trials, p the probability of a success. Trials are independent
Example Determine the probability of getting exactly two heads in three tosses of a biased coin for which the probability of a head = 2 3 We can solve this problem using a tree diagram easily 2 3 H 2 3 P(2 Heads) = P(HHT) + P(HTH) + P(THH) H 1 3 T 2 3 H 2 3 H P(HHT) = P(HTH) = P(THH) 2 3 3 27 1 3 2 1 =4 = T 1 3 T 2 3 P(2 Heads in 3 tosses) = 3(4 H 27) 2 3 H 1 3 1 3 = 4 T T 9 2 3 H 1 3 T 1 3 T
Probability based Questions A fair die is rolled 8 times. Find the probability of: a) No sixes b) Only 3 sixes Q Probability of not rolling a six is 5 a 6 ? 8 5 6 ? ?? ????? = b 3 were six and 5 weren t six. If S means a six was thrown and N means it wasn t throw, one possibility is 3 5 1 6 5 6 SSNNNSNN, which has probability But there s 8 3 8 3 ? 8! = 3!5!ways of arranging 3 S s and 5 N s. So: 1 6 6 3 5 5 ? 3 ????? = = 0.104
Example 2 Determine the probability of getting exactly two heads in sixty-five tosses of a biased coin for which the probability of a head = 2 3 We only use tree diagrams when n is small, so we check to see if we can use the Binomial Distribution There is a fixed number (n) of trials Each trial has two possible outcomes a success or a failure The probability of a success (p) is constant from trial to trial Trials are independent of each other Yes n = 65 Yes success heads, failure = tails Yes p = 2 3 Yes trials are independent
Example 2 Determine the probability of getting exactly two heads in sixty-five tosses of a biased coin for which the probability of a head = 2 3 1. First we need to figure out how many combinations there are: ? ? This represents the number of ways of choosing r items out of n items Remember If there are exactly r success, then there are n-r failures ? ? = 65 65 2 63 = 2080 ? = ? ? Can anyone explain why?
Example 2 Determine the probability of getting exactly two heads in sixty-five tosses of a biased coin for which the probability of a head = 2 3 2. Next we calculate the probability using the following formula: ? ? ??(1 ?)? ? ? ? = ? = Where 1 p, it is the probability of failure 2 63= 8.08 x 10-28 65 2 2 3 1 3 ? ? = 2 =
Eggs are packed in boxes of 12. The probability that each egg is broken is 0.35 Find the probability in a random box of eggs: there are 4 broken eggs 12 ) 4 . 0 = = . 0 = . 0 . 0 4 12 ( 4 8 ( ) 4 35 65 495 35 65 P X 4 significan 3 to 235 . 0 = figures t
Quickfire Questions Show the calculation required to find the indicated probability given the distribution. ?? ? ?.?? ?.?? ? ?~? 10,0.3 ? ? = ? = ?? ? ?.?? ?.?? ? ?~? 10,0.2 ? ? = ? = ? ? ?.?? ?.?? ? ?~? 5,0.1 ? ? = ? = ? ? = ?? = ?.???? ?~? 20,0.45 ? ? ? = ? = ?.???? ?~? 20,0.45 ?
Test Your Understanding ?~? 12,1 Q1 6 What is ?(? = 2)? ? ?? ? ? ? ? ? ?? ? ? ? = ? = = ?.??? What is ?(? 1)? ? ? ? = ? ? = ? + ? ? = ? ? ? = ?.??? ?? ? ?? ? ? ? ? +?? ? = ? I have a bag of 2 red and 8 white balls. ? represents the number of red balls I chose after 5 selections (with replacement). Q2 a How is ? distributed? ? ?~? ?,?.? b Determine the probability that I chose 3 red balls. ? ? ?.???.??= ?.???? ? ? ? = ? = (If you get these quickly, go on to Exercise 1B)
Eggs are packed in boxes of 12. The probability that each egg is broken is 0.35 Find the probability in a random box of eggs: There are less than 3 broken eggs = = + ) 1 = + = ( ) 3 ( ) 0 ( ( ) 2 P X P X P X P X 12 12 12 . 0 . 0 . 0 = . 0 + . 0 + . 0 0 12 ( ) 1 11 ( ) 2 10 ( ) 35 65 35 65 35 65 0 1 2 = 005688 . 0 + . 0 . 0 + . 0 01346 . 0 = 1 11 1 1 12 35 65 66 1225 . 0 0151
Exercise 1B The random variable ?~? 8,1 Find ? ? = 2 = ?.??? ? ? = 5 = ?.???? ? ? 1 = ?.??? A student suggests using a binomial distribution to model the following situations. Give a description of the random variable, state any assumptions that must be made and give possible values for ? and ?. 1 5 3 ? A sample of 20 bolts is checked for defects from a large batch. The production process should produce 1% of defective bolts. ?~? ??,?.?? assuming bolts being defective are independent from each other. ? a The random variable ?~? 15,2 Find ? ? = 5 = ?.????? ? ? = 10 = ?.??? ? 3 ? 4 = ?.????? 3 3 ? ? Some traffic lights have three phases: stop 48% of the time, wait or get ready 4% of the time and go 48% of the time. Assuming that you only cross a traffic light when it is in the go position, model the number of times that you have to wait or stop on a journey passing through 6 sets of traffic lights. ?~? ?,?.?? assuming lights operate independently. ? b ? A balloon manufacturer claims that 95% of his balloons will not burst when blown up. You have 20 balloons. What is the probability that none of them burst? ?.????= ?.??? ?? ??? What is the probability exactly 2 burst? = ?.??? ? 4 When Stephanie plays tennis with Tim on average one in eight of her serves is an ace . How many aces does Stephanie serve in the next 30 serves against Tim? ?~? ??,? ? assuming serves are independent and probability of an ace is constant. ? c ?
Question It is known that 80% of the seeds of particular flowers will germinate in the right conditions. If a packet of 10 seeds is purchased, find the probability that: There is a fixed number (n) of trials Each trial has two possible outcomes a success ora failure The probability of a success (p) is constant from trial to trial Trials are independent of each other a) at most two will fail to germinate. [2] b) exactly 8 will germinate. [2] Hint, you may need to think carefully about what you call a success for each question B(n,p) c) Between 3 and 6 seeds inclusive will germinate [3] Find your factor
Solution a) P(X 2) = 0.6778 Number failing to germinate ~ B(10, 0.2) B1 B1 10 8 0.880.22 = 0.3020 b) M1 A1 cao c) P(X 6) P(X < 3) = 0.1208 Between 3 and 6 inclusive don t germinate M1 M1 A1
Overview So Far These are all based on the parameters we set. ? ? ? ??? ? ? Description Name Params Outcomes Prob Func Number of trials ? 0,1, ,? We count the number of successes after a number of trials, each with two outcomes ( success and failure ). e.g. Number of heads after 10 throws of an unfair coin. Binomial Distribution ? ?,? ? ? = ? ???1 ?? ? ? Probability of success in each trial ? ? ? Still to cover: The Cumulative Distribution Function ?(?) Calculating ? ? and ??? ?