Understanding Binomial Experiments and Probability Calculations
Binomial experiments have specific conditions to be met, such as fixed trials and two outcomes. Symbols and notations help represent these experiments. Calculating probabilities of successes in trials involves using functions like binompdf and binomcdf. Examples like determining defective switches or children's blood types illustrate these concepts in practice.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
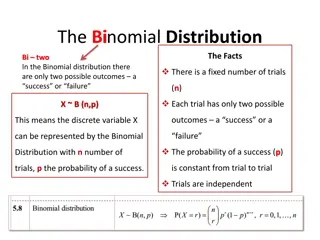
Section 8.1 - Binomial Distributions For a situation to be considered a binomial setting, it must satisfy the following conditions: 1) Experiment is repeated a fixed number of trials and each trial is independent of the others 2) There are only two possible outcomes: success (S) and failure (F). 3) The probability of success, P(S), is the same for each trial 4) The random variable, x, counts the number of successful trials
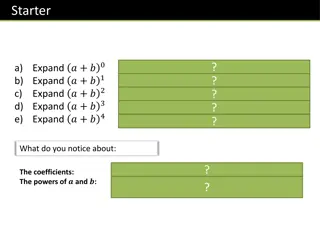
Symbols and Notations for Binomial Settings n = number of trials in the sample p = P(S) probability of success in a single trial x = count of the number of successes in n trials this is called a binomial random variable A binomial experiment can be symbolized as B(n,p) x 0 1 2 3 px The probability distribution of the successes is referred to as a binomial distribution
Are these binomial experiments? (identify n, p, and x) 1) If both parents carry the genes for the O and A blood types, each child has a probability of 0.25 of getting two O genes and therefore having blood type O. 5 children of these parents are chosen to observe their blood type. Success is considered having blood type O. 2) Deal 10 cards from a shuffled deck and count the number, x, of red cards. Success is considered as getting a red card. 3) An engineer chooses a SRS of 10 switches from a shipment of 10,000 switches. Suppose that (unknown to the engineer) 10% of the switches in the shipment are bad. The engineer counts the number, x, of bad switches (success). Choosing a SRS of size n from a population, where the population is much larger than the sample, the count of X successes in the sample is approximately B(n,p)
There are several ways to find the probability of EXACTLY k successes in n trials. There is a formula, but we ll use the calculator. Binompdf(n, p, k) Using the engineer looking for defective switches example which can be approximated as B(10,0.1) What is the probability that no more than 1 switch is defective?
To find the probability of UP TOk successes in n trials. We can also use the calculator. Binomcdf(n, p, k) If both parents carry the genes for the O and A blood types, each child has a probability of 0.25 of getting two O genes and therefore having blood type O. 5 children of these parents are chosen to observe their blood type, a) what is the probability that 2 or less children have blood type O? b) what is the probability that 4 or more have blood type O?
Example: Sample surveys show that fewer people enjoy shopping than in the past. A survey asked a nationwide random sample of 2500 adults if they agreed or disagreed with the statement I like buying new clothes, but shopping is often frustrating and time- consuming. The population that the poll wants to draw conclusions about is all US residents aged 18 and over. Suppose the in fact 60% of all adults US residents would say they agree with the statement. What is the probability that 1520 or more of the sample would agree?