Exploring Binomial and Poisson Distributions in Probability Theory
Understand the fundamentals of binomial and Poisson distributions through practical examples involving oil reserve exploration and dice rolling. Learn how to calculate the mean, variance, and expected outcomes of random variables in these distributions using formulas and probability concepts.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
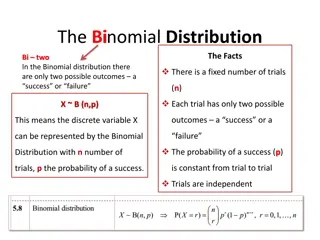
Binomial Distribution BAT use formulas to find the mean and variance of the binomial distribution KUS objectives Starter: A company is searching for oil reserves. The company has purchased the rights to make test drillings at four sites. It investigates these sites one at a time but, if oil is found, it does not proceed to any further sites. At each site, there is probability 0.39 of finding oil, independently of all other sites. The random variable X represents the number of sites investigated. The probability distribution of X is shown below. r 1 2 3 4 P(X = r) 0.39 0.2379 0.1451 0.227 a) Find the expectation and variance of X. b) b) It costs 66000 to investigate each site. Find the expected total cost of the investigation.
Notes derivation of Mean and Variance of a Binomial Distibution If X and Y are two independent random variables then ? ? + ? = ? ? + ? ? And ??? ? + ? = ??? ? + ??? ? So ? ??+ ??+ + ?? = ? ?? + ? ?? + ? ?? And ??? ??+ ??+ + ?? = ??? ?? + ??? ?? + ??? ?? Consider the random variable ?~?(?,?)and the random variables ??(? = 1,2,3, ?) which represent the number of successes on the nth trial. The distribution of each of the ?? will be the same It is easy to calculate ?(??) = ? ?(? = ?) = ? + ? ? = ? And ???(??) = ?? ? ? = ? (?)? = ?? ? ??= ? ? ? X is the total number of successes in the n trials and so ? = ??+ ??+ + ?? So you can apply the above result to get ? ? = ? ?? + ? ?? + ? ??= ? + ? + + ?= ?? ??? ? = ??? ?? + ??? ?? + ??? ??= ?(? ?) + + ?(? ?)= ??(? ?)
Notes If random variable ?~?(?,?) ? = ? ? = ?? ??= ??? ? = ??(? ?)
WB 21 mean and variance ? = ? ? = ?? ??= ??? ? = ??(? ?) A fair cubical die is thrown 36 times and the random variable X represents the number of sixes obtained a) Find the mean and variance of X b) Find ?(? < ? ?) a) The random variable ?~?(??,? ?) = ?? ? random variable ? = ? ? = ?? ?= ? ??= ??? ? = ??(? ?) = ?? ? ? ? ?= ? b) ?(? < ? ?) = ?(? < 6 5) = ? ? < 3.763 = ? ? 3 36 35 34 2 33 3 5 6 5 6 1 5 6 1 6 5 6 1 6 = + 36 6+ 630 + 7140 = 0.0014 + 0.0101 + 0.0355 + 0.0805 = ?.??? (???) Checking this result with a calculator n x = 3 n = 36 p = 1/6 gives ? ? 3 = 0.1277
WB 22 mean and variance ? = ? ? = ?? ??= ??? ? = ??(? ?) A fair, four sided die has the numbers 1, 2, 3 and 4 on its faces. The die is rolled 20 times. The random variable X represents the number of 4s obtained a) Find the mean and variance of X b) Find ?(? < ? ?) a) The random variable ?~?(??,? ?) = ?? ? ?= ? ? ? ? = ? ? = ?? = ?? ? ??= ??? ? = ??(? ?) ?= ?.?? b) ?(? < ? ?) = ?(? < 5 3.75) = ? ? < 3.06 = ? ? 3 Use c????????? ? = ??,? =? = ?.????(???) ?,? = ?
WB 23 mean and variance ? = ? ? = ?? ??= ??? ? = ??(? ?) The probability of a sales representative making a sale on a call is 0.15. Representatives are required to achieve a mean of 5 sales each day. Find the least number of calls each day a representative should make to achieve this target Let X = number of sales each day The random variable ?~? ?,?.?? and mean = 0.15n 0 15 . 5 33 = n n 5 . 1 0 15 3 At least 34 calls
WB 24 solve inequality ? = ? ? = ?? ??= ??? ? = ??(? ?) David believes that 35% of people in a certain town will vote for him in the next election and he commissions a survey to verify this. Find the minimum number of people the survey should ask to have a mean number of more than 100 voting for David. Let n = the number of people asked Let X = the number of people (out of n) voting for David ?~? ?, ?.?? ? = ? ? = ?.??? So ?.??? > ??? ? >??? ?.??= ???.? So the minimum number of people that should be asked is 286
WB 25 solve inequality ? = ? ? = ?? ??= ??? ? = ??(? ?) An examiner is trying to design a multiple choice test. For students answering the test at random, he requires that the mean score on the test should be 20 and the standard deviation should be at least 4. Assuming that each question has the same number of alternative answers, find how many questions and how many alternative answers each question should have. The number of alternatives for each question should be as few as possible. Let n = the number of questions Let p = the probability of guessing a correct answer Let X = the number of questions answered correctly at random ?~? ?, ? ? = ? ? = ?? So ?? = ?? ? ? So ?? ? ? ?? ?? ? ? ?? ? ?.? So the examiner should use ? = ?.? i.e. have 5 alternatives for each question And ?? = ?? with ? = ?.? gives ? = ??? i.e have 100 questions
Practice Ex 1D
KUS objectives BAT use formulas to find the mean and variance of the binomial distribution self-assess One thing learned is One thing to improve is