Understanding the Binomial Theorem
An explanation of binomials, Pascal's Triangle, and the Binomial Theorem with examples and applications in algebra. Special cases and series expansions are covered, providing insights into the manipulation of binomial expressions for various powers and applications. The content illustrates the expansion of different binomial expressions and the formula for (1 + x)^n under certain conditions.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
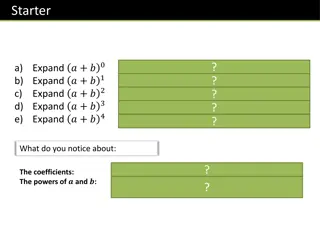
Introduction A binomial is an algebra expression containing 2 terms. For example, (x + y) is a binomial. We know that ? + ? 0 ? + ? 1= ? + ? ? + ? 2= ?2+ 2?? + ?2 ? + ? 3= ?3+ 3??2+ 3?2? + ?3 For higher powers, the expansion gets very tedious. Hence, the binomial theorem gives us the expansion for any positive integer power of (x + y)
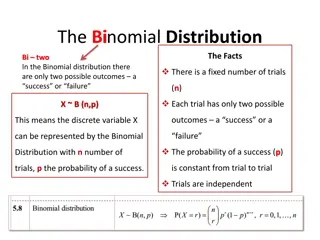
Binomial Theorem For any positive integer n, 1!?? 1? +? ? 1 ??? 1? + ?2 ? + ? ?= ??+? ?? 2?2+ + ?? 2! ??? 2?2+ + ????? ???+ + ?? = ??+ ?1 ? =? ? 1 ? 2 (? ? 1) ?! ???= ? where = ?! ? ? ! ?! ? ? + ? ?= ? ? ?? ??? In summation notation, ?=0 ??+1= ? ? ?? ??? The (r + 1)thterm is
Binomial Theorem (cont) A useful special case of the Binomial theorem is 1!? +? ? 1 ?? + ?2 1 + ? ?= 1 +? ?2+ + ?? 2! ??2+ + ?????+ + ?? = 1 + ?1 ??2+ + ?????+ + ?? = 1 + ?? + ?2 for any positive integer n.
Binomial Theorem (cont) Example 1: use the Binomial theorem to expand the following expressions. a) (x + y)5 b) (1 x2)4 c) (1 1/x)10 d) (2 3x)8
Binomial Series The formula of (1 + x)ncan be extended to all real powers, i.e. 1 + ? ?= 1 +? 2! 1!? +? ? 1 ?2+? ? 1(? 2) ?3+ 3! This expansion is valid for any real number n if |x|< 1. Important notes: A. Binomial theorem deals with a finite expansion, i.e. n is a positive integer B. It cannot use the button for the binomial series. It applies only to power of positive integer. ???
Binomial Series (cont) Example 2: Use the binomial series formula to find the first four terms of the following expansion. a) (1 + x)1/2 b) (4 + x2)1/2 c) (1 2x)-1 d) (2 x)-2