Understanding Radian and Degree Measure for Arc Length and Area of a Sector
Exploring radians and degrees in relation to arc length, sector area of a circle, and converting measurements between degrees and radians. Learn how to find arc length and sector area given central angles and radii, as well as convert degrees to minutes and seconds or vice versa.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
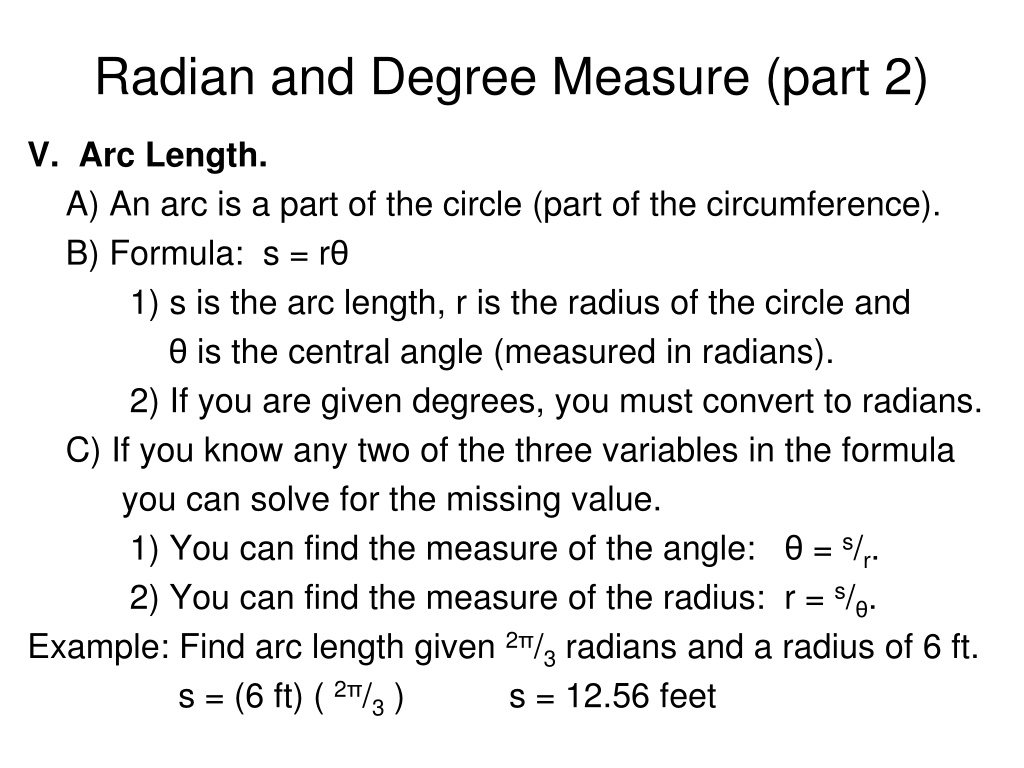
Radian and Degree Measure (part 2) V. Arc Length. A) An arc is a part of the circle (part of the circumference). B) Formula: s = r 1) s is the arc length, r is the radius of the circle and is the central angle (measured in radians). 2) If you are given degrees, you must convert to radians. C) If you know any two of the three variables in the formula you can solve for the missing value. 1) You can find the measure of the angle: = s/r. 2) You can find the measure of the radius: r = s/ . Example: Find arc length given 2 /3radians and a radius of 6 ft. s = (6 ft) ( 2 /3) s = 12.56 feet
Radian and Degree Measure (part 2) VI. Area of a Sector of a Circle. A) A sector is a piece of the interior of the circle (area). B) Formula: A = r2 . 1) A is the Area, r is the radius, is the angle in radians. C) If you know any two of the three variables in the formula you can solve for the missing value. 1) You can find the measure of the central angle: = 2 r A 2 2 A 2) You can find the radius: r =
Radian and Degree Measure (part 2) VII. Evaluating degrees minutes seconds (D M S ) A) The distance between two degrees (ex: 15 & 16 ) can be 1) divided into minutes (60 min = 1 ) 2) and seconds (3600 sec = 1 ). B) To convert into decimal form 1) write the degree number (this is the whole #). 2) divide the minutes # by 60. 3) divide the seconds # by 3600 (60 60). 4) Add all three numbers you found together. Example: 43 18 39 would be 43 + 18/60+ 39/3600 43.31
Radian and Degree Measure (part 2) VIII. Evaluating degrees minutes seconds (D M S ) C) To convert a decimal into degrees-minutes-seconds 1) Take the whole # part (the part in front of decimal). a) It is your degrees number. 2) Take the decimal part and multiply it by 60. a) Take the whole # part. It is your minutes. b) There may be a decimal left over its for seconds. 3) Take the left over decimal number & multiply it by 60. a) Whatever number you get is your seconds. Examples: Convert 40.3472 to degree-min-sec = 40 degrees .3472 60 = 20.832 so 20 minutes .832 60 = 49.92 seconds