Understanding Coulomb Interaction in Many-Particle Hamiltonian
Explore the treatment of Coulomb interaction in a many-particle Hamiltonian, where careful integration is crucial due to divergence issues. Learn about solving the Coulomb Hamiltonian with Slater integrals and expanding the operator on spherical harmonics for analytical solutions. Discover the significance of Coulomb repulsion, Slater integrals, and the radial and angular parts in this intricate process.
- Coulomb Interaction
- Many-Particle Hamiltonian
- Slater Integrals
- Spherical Harmonics
- Analytical Solutions
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
The following slides show you how to treat the Coulomb interaction in a many particle Hamiltonian. As the Coulomb interaction diverges for the case where the two electrons have the same position one needs to be careful on how to integrate. As our basis functions are atom centered radial functions times spherical harmonics (or linear combinations thereof) we can expand the Coulomb interaction on spherical harmonics as well and treat the angular part analytical. The radial part are the Slater integrals. An excellent text book introducing this topic is Ballhausen Ligand field theory chapter 1 and 2.
Coulomb repulsion and Slater Integrals Maurits W. Haverkort Institute for theoretical physics Heidelberg University M.W.Haverkort@thphys.uni-heidelberg.de
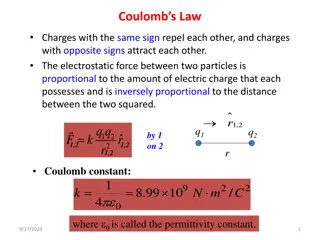
The Coulomb Integral is nasty: The integrant diverges at r1=r2 Coulomb Hamiltonian: In order to create the Hamiltonian as a matrix we need to evaluate the following integral Solution by Slater: Expand the operator on Spherical Harmonics. Solve the angular part analytical and the Radial integral numerical (Slater Integrals.) Also works in solids. (Spherical Harmonics are not eigen-states, but still a valid basis set.
Coulomb interaction Slater Integrals Expansion on renormalized Spherical Harmonics with Useful expansion because our basis functions are (close to) spherical
Coulomb interaction Slater Integrals Integral to calculate Expansion on renormalized Spherical Harmonics
Coulomb interaction Slater Integrals Radial part: Slater integrals Angular part: Analytical solution
Coulomb interaction Slater Integrals Graphical representation
Coulomb interaction Slater Integrals Triangular equations
Coulomb interaction Slater Integrals d - electrons
Coulomb interaction Slater Integrals f - electrons
Coulomb interaction Slater Integrals Core (p) valence (d) interaction direct term
Coulomb interaction Slater Integrals Core (p) valence (d) interaction exchange term