Understanding Binomial Theorem: Expansion, Examples, and Applications
Binomial theorem is a powerful mathematical concept used to expand expressions involving binomials. This presentation explores the basics of binomial expansion, formulae for positive, negative, and fractional indices, along with examples demonstrating its application. By leveraging the binomial theorem, complex algebraic expressions can be simplified efficiently, making calculations more manageable. Dive into the world of binomial theorem to unlock its potential in solving mathematical problems.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
Welcome to my Presentation Hossain Md Waseem Firoz Instructor (Mathematics) Kushtia Polytechnic Institute
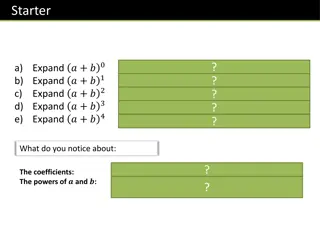
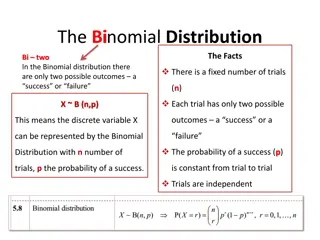
Chapter 3 (Binomial Theorem) What Is Binomial Expansion In Math's? Binomial expansion is to expand and write the terms which are equal to the natural number exponent of the sum or difference of two terms. For two terms x and y the binomial expansion to the power of n is (x + y)n=nC0 0 xny0+nC1 1 xn - 1y1+nC2 2 xn-2y2+nC3.
Binomial theorem primarily helps to find the expanded value of the algebraic expression of the form (x + y)n. Finding the value of (x + y)2, (x + y)3, (a + b + c)2is easy and can be obtained by algebraically multiplying the number of times based on the exponent value. But finding the expanded form of (x + y)17or other such expressions with higher exponential values involves too much calculation. It can be made easier with the help of the binomial theorem. The exponent value of this binomial theorem expansion can be a negative number or a fraction. Here we limit our explanations to only non-negative values. Let us learn more about the terms, formula and the properties of coefficients in this binomial expansion article.
The binomial theorem is also known as the binomial expansion which gives the formula for the expansion of the exponential power of a binomial expression. Binomial expansion of (x + y)nby using the binomial theorem is as follows, (x+y)n=nC0xny0+nC1xn-1y1+nC2xn-2y2+ ... +nCn-1x1yn-1+nCnx0yn