Exploring Equidistant Points and Circle Equations

In this engaging content, we delve into the concept of equidistant points from the origin as well as a specific point. We explore the coordinates of such points, their fitting into equations, and extending this learning to circles with various centers. Furthermore, we discuss writing equations for circles with diverse centers and radii. The class discussion wraps up by summarizing the key takeaways from the lesson.

• Equidistant Points
• Circle Equations
• Coordinates
• Learning Extension
• Summary

gmerr Follow

Uploaded on Sep 26, 2024 | 0 Views

Download Presentation

Please find below an Image/Link to download the presentation.

The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.

Presentation Transcript

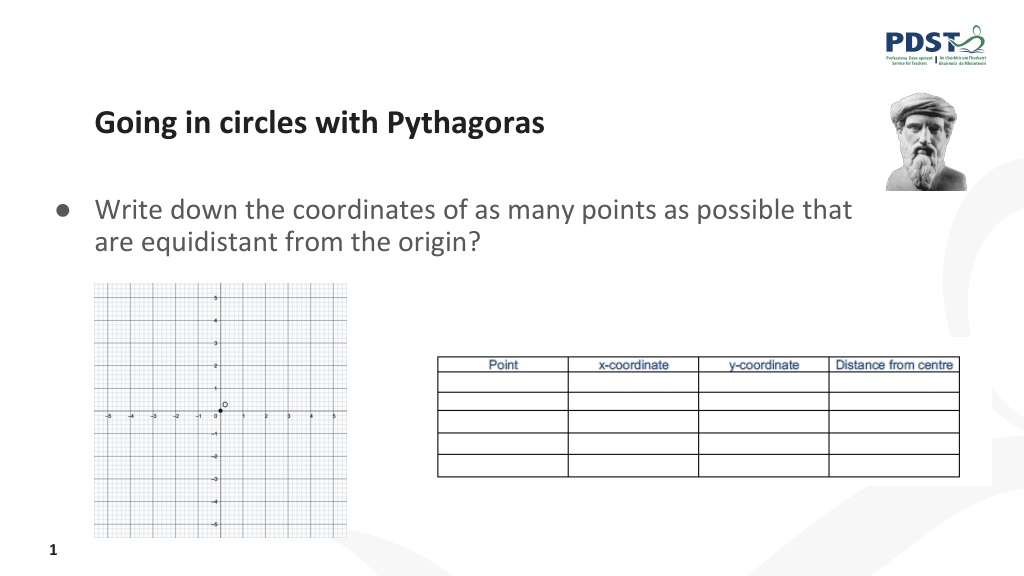
1. Going in circles with Pythagoras Write down the coordinates of as many points as possible that are equidistant from the origin? 1

2. Write down the coordinates of as many points as possible that are equidistant from the point (3, 2)? Do these points fit into an equation? Why does this work? 2

3. Group Discussion Should we be able to extend this learning to any size circle with centre (3,2)? How about any circle, with any centre? Can we write an equation like this for any circle?

4. What is the equation of this circle with centre c and radius 2? A(x,y) 2 c(3,2) 4

5. What is the equation of this circle with centre c and radius r? B(x,y) r c(h,k)

6. Class Discussion Summarise your learning from today s lesson 6