Coordinates on the Unit Circle

Coordinates on the Unit Circle

Based upon the unit circle activity, we have found that the

(x, y) coordinates on the unit circle match to the cos and

sin of the angle.

 This means that if we know the coordinates of any angle

on the

unit circle

, we could find sin, cos, and tan of that

angle.

But what if the point is NOT on the unit circle?

The (x, y) no longer represents the cos and sin of the angle.

We can still use what we observed in the activity to help us…

Any circle can represent the angles of rotation.  If a coordinate lies on

the terminal side of any angle, then those values are the horizontal

and vertical sides of a right triangle.

Example:

  The point (-3, 4) lies on the terminal side of an angle in

standard position.

This means that we have a triangle with a horizontal side of -3

and a vertical side of 4.

Let’s draw this triangle and see if we can find the values of the 3 basic

trigonometric functions.

Slide Note

Embed Share

Download

Exploring how the coordinates on the unit circle correspond to trigonometric functions like sine, cosine, and tangent. Learn how to find these values for angles on the unit circle and how to apply trigonometry principles when points are not on the unit circle.

eshal Follow

Uploaded on Apr 17, 2024 | 9 Views

Download Presentation

Please find below an Image/Link to download the presentation.

The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author.If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.

You are allowed to download the files provided on this website for personal or commercial use, subject to the condition that they are used lawfully. All files are the property of their respective owners.

Download Presentation

The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author.

E N D

Presentation Transcript

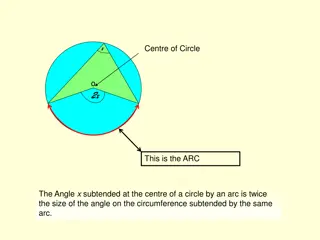
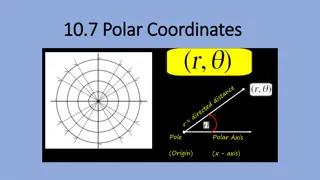
Coordinates on the Unit Circle Based upon the unit circle activity, we have found that the (x, y) coordinates on the unit circle match to the cos and sin of the angle. This means that if we know the coordinates of any angle on the unit circle, we could find sin, cos, and tan of that angle.

Example: Given that the terminal side of an angle, ?, crosses the 3,1 tan ?? 5 UNIT CIRCLE at the point 3, what is the sin ?, cos ?, and Since the point is on the UNIT CIRCLE, the (x,y) matches to (cos ?, sin ?). sin ? = To find the tan ?, we can use what we know about SOH-CAH-TOA. cos ? =

Example cont: Given that the terminal side of an angle, ?, crosses the 5 3,1 tan ?? UNIT CIRCLE at the point 3, what is the sin ?, cos ?, and Another way you can find tan? is by a special relationship, ???? = ??? ? ??? ?. This means if you divide the value of sin by the value of cos, you will get the value of tan. Sin Cos keep, change, flip

But what if the point is NOT on the unit circle? The (x, y) no longer represents the cos and sin of the angle. We can still use what we observed in the activity to help us Any circle can represent the angles of rotation. If a coordinate lies on the terminal side of any angle, then those values are the horizontal and vertical sides of a right triangle.

Example: The point (-3, 4) lies on the terminal side of an angle in standard position. This means that we have a triangle with a horizontal side of -3 and a vertical side of 4. Let s draw this triangle and see if we can find the values of the 3 basic trigonometric functions.