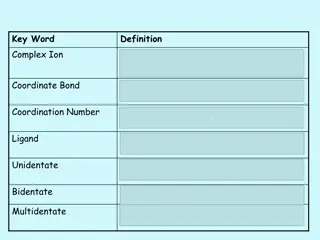
Coordinate-based Polygon Area Calculation Method

Utilize a step-by-step approach to determine the area of a polygon by listing coordinates in columns, calculating trapezium areas, and summing them up. This versatile method can be applied to any polygon, not limited to triangles.

• Polygon Area Calculation
• Coordinate System
• Trigonometry Method
• Geometric Analysis

latt_m Follow

Uploaded on Nov 23, 2024 | 0 Views

Download Presentation

Please find below an Image/Link to download the presentation.

The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author.If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.

You are allowed to download the files provided on this website for personal or commercial use, subject to the condition that they are used lawfully. All files are the property of their respective owners.

The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author.

Presentation Transcript

1. Survey Plot

2. Find the exact area of the polygon. Area = sq. units L

3. ? ?2,?2 What s the area of this triangle? ?3,?3 ?1,?1 ?

4. ? ?2,?2 What s the area of this triangle? ?3,?3 ?1,?1 ? ?1,0 ?2,0 ?3,0

5. ? ???+ ?? ? ???+ ?? ? ???+ ?? ?? ?? ?? ?? ?? ??

6. ? ???+ ?? ? ???+ ?? ? ???+ ?? ?? ?? ?? ?? ?? ??

7. So, list the co-ordinates in columns in a spreadsheet, repeating the first co-ordinates at the end. Then enter a formula for the area of the trapezium formed by the first pair of co-ordinates. Copy down this formula to the last point (point 3). Finally, sum the areas of the trapezium. Point Trapezium area 1 2?2+ ?1 ?2 ?1 1 2?3+ ?2 1 2?1+ ?3 ? ????? ? ????? ?1 ?1 1 ?2 ?2 2 ?3 ?2 ?3 ?3 3 ?1 ?3 ?1 ?1 1 Total

8. So, list the co-ordinates in columns in a spreadsheet, repeating the first co-ordinates at the end. Then enter a formula for the area of the trapezium formed by the first pair of co-ordinates. Copy down this formula to the last point (point 3). Finally, sum the areas of the trapezium. Point Trapezium area 1 2?2+ ?1 ?2 ?1 1 2?3+ ?2 1 2?1+ ?3 ? ????? ? ????? ?1 ?1 1 ?2 ?2 2 ?3 ?2 ?3 ?3 3 ?1 ?3 ?1 ?1 1 Total

9. So, list the co-ordinates in columns in a spreadsheet, repeating the first co-ordinates at the end. Then enter a formula for the area of the trapezium formed by the first pair of co-ordinates. Copy down this formula to the last point (point 3). Finally, sum the areas of the trapezium. Point Trapezium area 1 2?2+ ?1 ?2 ?1 1 2?3+ ?2 1 2?1+ ?3 ? ????? ? ????? ?1 ?1 1 ?2 ?2 2 ?3 ?2 ?3 ?3 3 ?1 ?3 ?1 ?1 1 Total

10. So, list the co-ordinates in columns in a spreadsheet, repeating the first co-ordinates at the end. Then enter a formula for the area of the trapezium formed by the first pair of co-ordinates. Copy down this formula to the last point (point 3). Finally, sum the areas of the trapezium. Point Trapezium area 1 2?2+ ?1 ?2 ?1 1 2?3+ ?2 1 2?1+ ?3 ? ????? ? ????? ?1 ?1 1 Think of this point as point 4 in the list. ?2 ?2 2 ?3 ?2 ?3 ?3 3 ?1 ?3 ?1 ?1 1 Total This process works with ANY polygon, not just a triangle.

11. An example of what your spreadsheet could look like

12. ? This process works with ANY polygon, not just a triangle. And it doesn t have to be convex either! ?

13. This process works with ANY polygon, not just a triangle. And it doesn t have to be convex either!

14. What if the polygon had a hole in it? So to include a hole insert its co-ordinates into the existing list taking care to repeat connecting points (i.e. J and K) and avoid crossing over previous lines. F D G C E N H B hole L R A I O K Q J P M S

15. You should all get the same answer: 1501 sq. units. How was this achieved? The position of A, C, E and G is the same on all worksheets. The points form a parallelogram (although the shape is irrelevant). All triangles with a side of ACEG as a base share the same height (and so the same area).

17. Note to Teacher It is useful to get the pupils to insert a chart of their survey plot. Just highlight the coordinates Insert scatter chart (with points joined by straight lines) They should now see a representation of their worksheeet.

18. RESOURCES

19. Find the exact area of the polygon. Area = sq. units A SIC_28

20. Find the exact area of the polygon. Area = sq. units B SIC_28

21. Find the exact area of the polygon. Area = sq. units C SIC_28

22. Find the exact area of the polygon. Area = sq. units D SIC_28

23. Find the exact area of the polygon. Area = sq. units E SIC_28

24. Find the exact area of the polygon. Area = sq. units F SIC_28

25. Find the exact area of the polygon. Area = sq. units G SIC_28

26. Find the exact area of the polygon. Area = sq. units H SIC_28

27. Find the exact area of the polygon. Area = sq. units I SIC_28

28. Find the exact area of the polygon. Area = sq. units J SIC_28

29. Find the exact area of the polygon. Area = sq. units K SIC_28

30. Find the exact area of the polygon. Area = sq. units L SIC_28

31. Find the exact area of the polygon. Area = sq. units M SIC_28

32. Find the exact area of the polygon. Area = sq. units N SIC_28