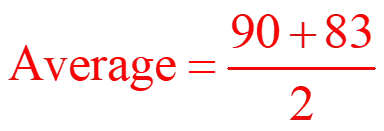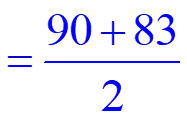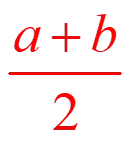Midpoint and Distance in Coordinate Plane

This content covers the concepts of finding midpoints and distances in the coordinate plane. It explains how to calculate the midpoint of line segments on a number line and in a coordinate plane using the midpoint formula. Additionally, it discusses finding the distance between two points using the distance formula based on the Pythagorean theorem. Practice examples and classwork/homework suggestions are also included.

• Midpoint
• Distance
• Coordinate Plane
• Math
• Formulas

wolfe Follow

Uploaded on Aug 21, 2024 | 0 Views

Download Presentation

Please find below an Image/Link to download the presentation.

The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.

Presentation Transcript

1. 1-7: Midpoint and Distance in the Coordinate Plane

2. Mean Average If you had test scores of 90 and 83, how would you find your average? Average Midpoint To find the midpoint of a segment on a number line. 83 + 90 83 2 = = 86.5 90 B A + 90 83 2 173 2 = = = Midpoint of AB 86.5 Number Line Midpoint Formula On a number line, the coordinate of the midpoint of the segment with endpoints a and b is: a b + 2

3. Midpoint in a Coordinate Plane + B(6,8) 6 1 2 = 3.5 (3.5,5.5) + 8 3 2 = 5.5 (6,3) A(1,3) Coordinate Plane Midpoint Formula In the coordinate plane, the midpoint of the segment with endpoints (x1, y1) and (x2, y2) is: + + x x y y = M , 1 2 1 2 2 2

4. AB Ex. 1: Find the coordinates of the midpoint of endpoints A (2, 3) and B (-5, 7). + = 2 with the 2 ( 5) + + 3 7 2 2 ( 5), 2 ( ) = 1.5 ,5 M has coordinates (3, 4). Point A AB Ex. 2: The midpoint of has coordinates (-3, -2). Find the coordinates of B.

5. Distance AB = 6 1 = 5 To find the horizontal or vertical distance: A B Apply same idea to finding distance on the Coordinate Plane: Distance formula based on Pythagorean Theorem: 2 a b + a x x = B = 2 2 2y c c = b y y 2 1 2 1 b A = + 1y 2 2 c a b a = + 2 2 ( ) ( ) c x x y y 2 1 2 1 1x 2x

6. Distance Formula ( ) ( ) , , B x y A x y The distance between two points and is: ( d x x = 2 2 1 1 + 2 2 ) ( ) y y 2 1 2 1 Ex. 3: Find the distance between each pair of points. a). (1, 2), (3, 4) b). (-1, -2), (-3, -4) ( ) ( ) ( ) ( ) 2 2 2 2 d = 3 1 + 4 2 d = 3 ( 1) + 4 ( 2) d = 4 4 + d = 4 4 + d = 8 2.83 d = 8 2.83

7. Classwork: p. 54 #7, 9, 11, 13, 17, 19, 23, 27, 31, 33, 46, 48 Homework: Review for test p. 71 #7-25, 30-37