Review: Design Eq & Conversion
Design equations for batch, flow, CSTR, PFR, and PBR systems are discussed, emphasizing the calculation of conversion and reactor volumes. The importance of understanding reaction rates and stoichiometry in determining reactor sizes is highlighted, along with numerical evaluation techniques for inte
0 views • 25 slides
Integral Calculus: Two Major Approaches & Antiderivatives
In this chapter, we delve into the fundamental concepts of integral calculus, focusing on two major approaches to mathematically generate integrals and assigning physical meanings to them. We explore antiderivatives, differentiation, integration, and the process of taking integration as the inverse
4 views • 59 slides
Tight Binding Model Adapted for Graphene from Kittel Chapter 9
This content discusses the tight binding model adapted for graphene based on Kittel Chapter 9, covering topics such as Bloch conditions, hybridization, sublattices, and overlap integrals. It explores the uniqueness of graphene's structure and its impact on coefficients in the calculations.
2 views • 16 slides
Trigonometric Integrals: Strategies and Identities
Learn useful trigonometric identities and strategies for integrating powers of sine and cosine. Understand when to use Pythagorean, Half or Double Angle Identities, and how to handle odd or even powers efficiently. Examples provided for clarity.
1 views • 14 slides
Calculus I Lecture #13: Volumes of Solids and Solids of Revolution
Exploring the concepts of finding volumes of solids using integrals, by slicing solid objects with parallel planes and calculating cross-sectional areas. Examples include calculating the volume of a pyramid and a curved wedge. The method of solids of revolution using the disk method is also discusse
1 views • 12 slides
Review of Definite Integrals using the Residue Theorem
Singular integrals involving logarithmic and non-integrable singularities are discussed, emphasizing integrability in the principal value sense. Cauchy Principal Value integrals and examples of their evaluations for singularities like 1/x are explored, highlighting the necessity of passing through t
1 views • 48 slides
Overview of Semi-Empirical Methods Based on Hartree-Fock
Semi-empirical methods derived from Hartree-Fock theory aim to reduce computational effort by approximating or eliminating electron repulsion integrals. Strategies include introducing adjustable parameters to replace ERI calculations and utilizing zero differential overlap methods like CNDO, INDO, N
5 views • 11 slides
Accumulation Problems and Definite Integrals in Applied Calculus
Explore the interpretation of definite integrals in accumulation problems, where rates of change are accumulated over time. Learn how to solve accumulation problems using definite integrals and avoid common mistakes by understanding when to use initial conditions. Discover the relation between deriv
0 views • 9 slides
The Residue Theorem in Complex Analysis
The Residue Theorem is a powerful tool in complex analysis that allows us to evaluate line integrals around paths enclosing isolated singularities. By expanding the function in a Laurent series, deforming the contour, and summing residues, we can evaluate these integrals efficiently. This theorem ex
2 views • 31 slides
Integration: Geometrical Interpretation, Properties, and Methods
Integration is the inverse process of differentiation, where we find a function whose differential is given. This process involves basic formulae, methods like integration by parts, and geometrical interpretation. Properties of indefinite integrals and techniques such as integration by substitutions
6 views • 28 slides
Numerical Integration in Density Functional Theory (DFT)
The application of numerical integration techniques in Density Functional Theory (DFT) is crucial for solving the Eigenvalue Problem and evaluating energy functionals. This involves partitioning integrals, approximating integrals at atomic centers, defining partition functions, and ensuring cell fun
0 views • 15 slides
Geometric Probabilities: From Fractions to Integrals
Delve into the realm of geometric probabilities with insights on how to transition from fractions to definite integrals, utilizing technology for enhanced learning experiences. Understand the significance of probability calculations in quantifying likelihood, incorporating geometric representations
0 views • 26 slides
An Lp Theory for Outer Measures: Application to Singular Integrals II
This discussion covers various topics such as tents (or Carleson boxes), outer measures on the open upper half-plane, sizes of functions on tents, outer essential supremum on subsets, outer Lp spaces, embedding theorems, and estimates related to Linfity-Sinfty and weak L1-Sinfty. The content delves
0 views • 34 slides
Coulomb Interaction in Many-Particle Hamiltonian
Explore the treatment of Coulomb interaction in a many-particle Hamiltonian, where careful integration is crucial due to divergence issues. Learn about solving the Coulomb Hamiltonian with Slater integrals and expanding the operator on spherical harmonics for analytical solutions. Discover the signi
0 views • 15 slides
Coulomb Repulsion and Slater Integrals
Dive into the intricate world of Coulomb repulsion and Slater integrals, essential concepts in quantum physics. Explore the challenges posed by the diverging Coulomb integral and the complex calculations required to evaluate these interactions. Discover how Slater integrals play a crucial role in cr
1 views • 14 slides
Applications of Definite Integrals in Finding Areas
Explore the practical applications of definite integrals through the concept of finding areas. These images illustrate how definite integrals are used to calculate areas bounded by curves and lines, offering a visual representation of the integral calculus in action.
1 views • 9 slides
Area, Riemann Sums, and Definite Integrals
Antiderivatives are utilized to find area under curves, where Riemann Sums are employed for approximations. The process involves dividing intervals into rectangles for both approximate and exact area calculations. Definite integrals provide specific, finite values representing total displacement, wi
0 views • 8 slides
Properties of Definite Integrals in Mathematics II Second Semester
Delve into the world of definite integrals in Mathematics II Second Semester, understanding rules, properties, and applications through examples. Learn to find bounds, evaluate integrals, calculate areas under curves, and graph functions for a comprehensive understanding of this mathematical concept
1 views • 15 slides
The Fundamental Theorem of Calculus
Explore the connection between differential calculus and the definite integral through the fundamental theorem of calculus, which allows for the evaluation of complex summations. Discover the properties of definite integrals and how to apply the theorem to find areas under curves. Practice evaluatin
1 views • 15 slides
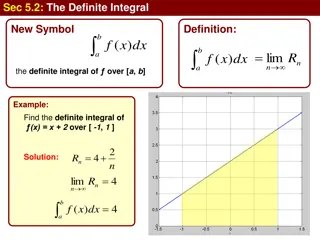
Definite Integrals in Calculus
Explore the concept of definite integrals in calculus, including their new symbol definition, area interpretation, and examples of evaluating integrals in terms of areas. Learn about the limits of integration, the procedure of integration, and how definite integrals can represent areas under curves.
1 views • 34 slides
Definite Integrals Using Graphing Display Calculator for Evaluation
Learn how to use a Graphing Display Calculator (GDC) to evaluate definite integrals step-by-step. Follow the instructions provided in the images to input the function and calculate the result with the GDC. This interactive process helps in understanding how technology can assist in mathematical comp
1 views • 17 slides
Definite Integrals in Mathematics
Explore the concept of definite integrals in mathematics, covering properties, solving methods, and applications in finding areas bounded by curves. Prior knowledge of functions, limits, derivatives, and indefinite integrals is essential to grasp this topic effectively. Dive into the unique value of
1 views • 32 slides
Definite Integration in Mathematics
Delve into the concept of definite integration in mathematics with a focus on finding areas under curves, evaluating definite integrals, and solving exercises that involve calculating areas between curves and the x-axis. Explore the principles and techniques behind finding total areas between specif
0 views • 22 slides
Integration and Antiderivatives
Exploring the concepts of definite and indefinite integrals, Riemann sums, and antiderivatives in calculus. Learn about interpreting the definite integral, Riemann sums as rectangles approximating integrals, and finding general antiderivatives. Discover various formulas for finding antiderivatives o
0 views • 16 slides
Mathematical Foundations: Bounding Summations and Series
Explore the mathematical foundations of bounding summations, including the sum of first n natural numbers and geometric series. Learn about bounding each term of series, monotonically increasing and non-decreasing functions, and approximating summations by integrals. Dive into proofs, examples, and
0 views • 17 slides
Analytical Integration of Functions: A Comprehensive Guide
Functions and their integrals are explored in this guide, covering definitions, simple integration cases, examples, and well-known integrals. Learn about differential equations, inspection integration, and trigonometric functions. Master the techniques with detailed explanations and examples.
2 views • 20 slides
Methods of Integration and Trigonometric Substitution
Explore methods of integration including integration by parts and trigonometric substitution. Learn how to apply these techniques to solve integrals involving logarithmic, trigonometric, and rational functions. Discover step-by-step processes and identities to simplify and evaluate various types of
3 views • 7 slides
Insights into Intra-Beam Scattering Effects and Plasma Dynamics in Particle Colliders
Intra-Beam Scattering (IBS) effects near transition crossing in NICA collider result in energy exchange between different degrees of freedom, impacting beam size, luminosity, and lifetime. The IBS phenomenon is crucial for circular machines, with theoretical developments by Piwinski and Bjorken shap
1 views • 15 slides
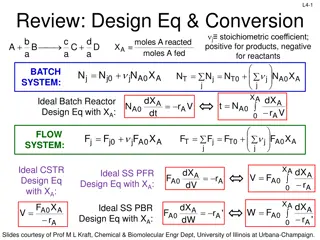
Review of Chemical Reactor Design Concepts and Numerical Integration Methods
This presentation covers various topics related to chemical reactor design, including batch systems, flow systems, ideal reactor design equations, CSTRs, PFRs, and PBRs. It also discusses the numerical evaluation of integrals using different methods such as Simpson's rules. Slides courtesy of Prof.
0 views • 25 slides
Advancing Phase-Space Integrands Decomposition in Particle Physics
Towards a universal approach for disentangling complex phase-space integrands in particle collisions, researchers are exploring methods to tame jet hair, cancel divergences in observables, and develop frameworks for precise calculations at various perturbative orders. The vision includes mastering m
1 views • 19 slides
An Lp Theory for Outer Measures and Applications to Singular Integrals II
This content delves into the theory of outer measures, focusing on its application to singular integrals. It covers topics such as Carleson boxes, outer measures on the upper half-plane, sizes of functions on tents, outer essential supremum spaces, Lp spaces, embedding theorems, and weak L1-Sinfty e
0 views • 34 slides
Asymptotic Evaluation Techniques in Integral Calculus
Learn about asymptotic evaluation of integrals through techniques like integration by parts and the stationary-phase method. Understand how to handle integrals involving real functions, and grasp the significance of concepts like the Riemann-Lebesgue lemma and small o notation. Delve into the physic
0 views • 21 slides
Innovative Approach in Mathematical Education for Maritime Students
Explore the innovative approach in mathematical education for maritime students as presented during the MareMathics Teachers Training and Meeting in Tallinn. The sessions covered topics such as mathematical applications in thermodynamics, including partial derivatives, derivations, and integrals wit
0 views • 11 slides
Advanced Quantum Chemistry: Solving Secular Determinants and Integrals
Lecture 3 delves into secular determinants, overlap, resonance, and Coulomb integrals, introducing the Hückel approximation method for molecular orbital calculations in inorganic and solid-state chemistry. The lecture explains the approximations made, simplifying the calculation of integrals and en
0 views • 20 slides
Integration in the Complex Plane: Definitions and Equivalences
Learn about line integrals in the complex plane, equivalence between complex and real line integrals, and review of line integral evaluation. Understand how to evaluate line integrals using parameterization and examples.
0 views • 53 slides
Monte Carlo Integration in Computer Graphics: Overview and Applications
Explore Monte Carlo Integration in computer graphics, from motivation to algorithms, advantages, and disadvantages. Learn about its application in rendering complex shading effects and how it provides robust solutions for irregular domains and high-dimensional integrals. Understand the basics of pro
0 views • 51 slides
Matrix Models and Superintegrability: Deformations and Integrals
Explore the concepts of superintegrability, W-operators, and matrix integrals in mathematical physics through deformations and generalizations. Learn about matrix models, Hermitian Gaussian model, W-representations, and more. Discover the significance of deformation of integration space, combining W
0 views • 11 slides
Tutorial for Multiloop Calculations: Master Integrals & Automated Solutions
Learn about multiloop calculations in theoretical physics with a tutorial by Marvin Schnubel. Discover the step-by-step process of reducing to master integrals, creating diagrams, IBP reduction, and solving integrals with automated tools. Find out how to generate expressions and handle complex compu
0 views • 9 slides
Study of Scalar Box Diagram via Loop-Tree Duality Theorem
Explore the analysis of the scalar box diagram using the Loop-Tree Duality theorem to understand the origins of singularities. The work delves into N-particle scalar one-loop integrals, massless scalar box integrals, dual representations, and parametrization of momenta to compute integrals efficient
0 views • 14 slides
Reducing Feynman Integrals Using Blade in Collider Physics Workshop
Exploring the reduction of Feynman integrals using advanced techniques like Blade in the context of collider physics. This presentation covers perturbative calculations, IBP reduction, algorithms, examples, and the significance of reducing integrals for precise predictions in high-energy physics.
0 views • 29 slides