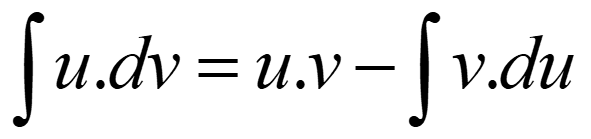Methods of Integration and Trigonometric Substitution
Explore methods of integration including integration by parts and trigonometric substitution. Learn how to apply these techniques to solve integrals involving logarithmic, trigonometric, and rational functions. Discover step-by-step processes and identities to simplify and evaluate various types of integrals effectively.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author.If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
You are allowed to download the files provided on this website for personal or commercial use, subject to the condition that they are used lawfully. All files are the property of their respective owners.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author.
E N D
Presentation Transcript
Methods Methods of Integration of Integration
Steps of integration by parts: 1. Split the integration into two parts, one of which we denoted it u and the other is dv. 2. Differentiate u and integrate dv. Apply the formula: . Steps of integration by parts: 3 = dv . v . du . u u v Note once on the same question. Note: This method can be repeated more than
We use integration by parts method in the next four cases: A1. Integration of logarithmic functions when the derivative not available A2. Integration of Inverse Trigonometric functions when the derivative not available A3. Integration of two multiplied functions, one of them not derivative to the other A4. Integration of some trigonometric functions raised to a certain power
In mathematics, trigonometric substitution is replacing term or mathematical expression with a trigonometric function by using trigonometric identities, usually to simplify integrals containing radical expressions.
B1. If the integral contains the expression a a2 2 x x2 2, suppose x= cos B2. If the integral contains the expression a a2 2+ suppose x= sec B3. If the integral contains the expression x x2 suppose x= tan x= a sin z x=1 1- - sin a sin z , then use the identity sin2 2x x cos2 2x= + x x2 2, x= a tan z x =tan a tan z , then use the identity tan2 2x + sec2 2x = x +1 1 2 - - a a2 2, x= a sec z x= sec a sec z , then use the identity sec2 2x tan2 2x= x - -1 1
( ) p x = If rational function and p(x) polynomial with degree less than degree of the polynomial q(x), we factorized the denominator q(x) to linear factors or quadratic factors. ( ) f x ( ) q x
( ) p x A + B + = + + + ( )( ) ( ) ( ) x a x b x a x b A + ( ) p x B + C + = + + + 3 2 3 ( ) ( ) ( ) ( ) x a x a x a x a + A x + B + C ( ) p + x = + + + + 2 2 ( ( ) c ) ( ) ( ) ax bx g x h ax bx c g x h + + 2 where must not be factorized further, and A, B, C are constants must be evaluated. ax bx c