Trigonometric Integrals: Strategies and Identities
Learn useful trigonometric identities and strategies for integrating powers of sine and cosine. Understand when to use Pythagorean, Half or Double Angle Identities, and how to handle odd or even powers efficiently. Examples provided for clarity.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author.If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
You are allowed to download the files provided on this website for personal or commercial use, subject to the condition that they are used lawfully. All files are the property of their respective owners.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author.
E N D
Presentation Transcript
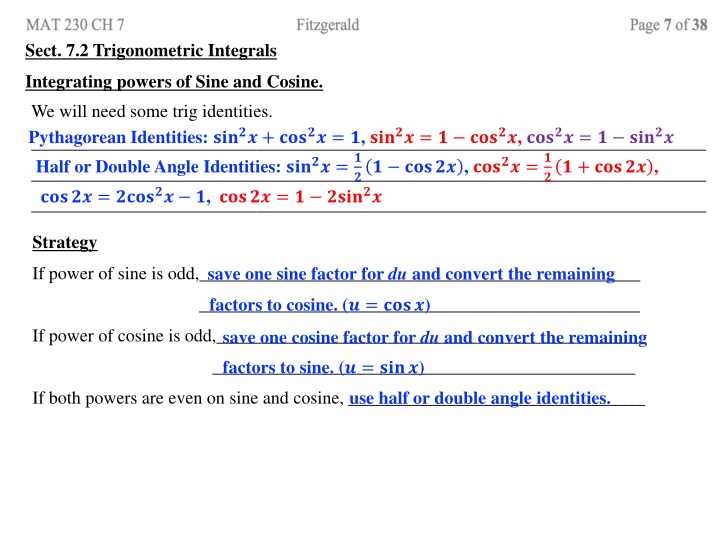
Sect. 7.2 Trigonometric Integrals Integrating powers of Sine and Cosine. We will need some trig identities. Pythagorean Identities: ????? + ????? = ?, ????? = ? ?????, ????? = ? ????? Half or Double Angle Identities: ????? =? ????? = ?????? ?, ????? = ? ?????? ___________________________________________________________________________ ?? ????? , ????? =? ?? + ????? , ___________________________________________________________________________ ___________________________________________________________________________ Strategy If power of sine is odd,_________________________________________________ save one sine factor for du and convert the remaining _________________________________________________ factors to cosine. (? = ????) If power of cosine is odd,_______________________________________________ save one cosine factor for du and convert the remaining factors to sine. (? = ????) _______________________________________________ use half or double angle identities. If both powers are even on sine and cosine, _________________________________
Sine has odd power, factor one out for du & u = cosx. Example 1: sin3? cos4? ?? u = _________ ???? = sin2? cos4? sin ? ?? Convert to cosine. = 1 cos2? du = ___________ ?????? ?? = ?????? cos4? sin ? ?? Now substitute = ? ??????= ?? ???? ?? ? ?? = ????? +????? = + ? + ? ? ? ?
Both sine & cosine have odd powers. Look back at the previous example to work smarter not harder! Better to break down the smallest power! Example 2: cos5? sin3? ?? u = _________ ???? du = ___________ ?????? = cos5? sin2? sin??? Convert to cosine. = cos5? 1 cos2? ?? = ?????? sin??? Now substitute = ??? ????= ?? ???? ?? ? ?? + ? = ????? +????? = + ? ? ? ?
Cosine has odd power, factor one out for du & u = sinx. The rest rewrite as cos2x. cos5? sin ??? Example 3: u = _________ ???? = sin 1 cos2? Convert to sine. 1 sin2? 2cos ? ?? 2 ? du = ___________ ?????? = sin 1 2cos ? ?? Now substitute 2 ? ? ? ??? ??= ? ? = ? ? ? ? ???+ ???? = ? ? ? ?+ ? ? ? ?? ? ?? ? ? ? ? ? ?+? ? ?+ ? = ?? ?? ??
Both sine & cosine have even powers...use half angle identities. ????? =? ?? + ????? Example 4: sin2? cos2? ?? ????? =? ?? ????? ? ?? ????? ? = ?? + ????? ?? ? ? ?????? ?? =? ? ?????? ?? =? ? ?? ??? ?? =? ?? ? + ? =? ? ????? ?? + ? =? ?? ? ?? ???? ??
Sine has an even power... use half angle identity. ????? =? Example 5: ?2sin2? ?? ?? ????? = ??? ?? ????? ?? = ? ? ??? ??????? ?? = ? ? ???? ????????? dx ?? ? ????????? Integration by Parts twice or Tabular Integration cos 2x ( ) 1 2sin 2x -1 4cos 2x -1 8sin 2x = ? u = + x2 = dv ? ( ) ( ) ( ) - 2x ?? ? ?? ???? ?? +? = ? ???? ?? ? ???? ?? + ? ? + 2 = ?? ? ?? ???? ?? ? ???? ?? +? ???? ?? + ? - 0
Integrating powers of Secant and Tangent. 1. If power of secant is even and positive, ________________________________________ save a sec2x factor for du convert remaining factors to tangent u = tan x ________________________________________ ________________________________________ save a sec x tan x factor for du convert remaining factors to secant u = sec x convert tan2x factor to a sec2x factor by Pythagorean Theorem use Integration by Parts. 2. If power of tangent is odd and positive, ________________________________________ ________________________________________ ________________________________________ 3. If no secant factors, power of tangent is even and positive, ________________________ __________________________________________ 4. sec????, m is odd and positive, ___________________________________________ try converting to sine and cosine. 5. None of the above, ____________________________________________ sec x tan x sec2x Review: ? ? ??sec? = ___________________ ln| sec x + tan x | + C ??tan? = __________ tan? ?? = ___________________ ln| sec x | + C tan2x + 1 =sec2x tan2x =sec2x 1 sec? ?? = _____________________________ Pythagorean Identity: ______________________________________
Example 6: tan3? sec2??? Secant has an even power, so... u = _________ ???? =? = ????=? ?????x + C ???+ C du = ___________ ??????? Tangent has an odd power, so save a secx tanx for du Example 7: tan3? sec2??? u = _________ sec x = ????????? ?????????? Convert to secant. = ????? ? ???? ?????????? = ?? ? ? ??= ?? ? ??=? du = _____________ sec x tan xdx Now substitute ??? ? ???+ C =? ? ??????+ C ??????
Both powers fit our rules and either method is the same amount of work. Example 8: sec43? tan33? ?? u = _________ ??? ?? Let s use secant to an even power so u = tan(3x) du = ___________ ??????? ?? = ?????? ?????? ?????? ?? Convert to tangent. ? ??? = ???? ?? ?? = ?????? + ? ?????? ?????? ?? Now substitute ? ??+ ? ?? ??= ? = ? ? ??+ ????= ? ? ???+? ???+ C ? = ? ? ???????? + C ???????? +
tan2? sec5??? Convert to sines and cosines. ????? ????? ????? Example 9: = Cancel ?? u = _________ ???? Cosine has an odd power, so save a cosine for du. = ????? ????? ?? du = ___________ ?????? = ????? ????? ??? ? ?? Convert to sine = ????? ? ????? ??? ? ??Substitute = ??? ????= ?? ???? = ? ??? ? ???+ ? = ? ?????? ? ?????? + ?
Example 10: tan3? ?? = ???? ??????? u = _________ ???? Convert tan2x to sec2x 1. = ???? ????? ? ?? du = ___________ ????? ?? = ????????? ???? ?? = ????????? ?? ?????? Substitute = ? ?? ?????? =? ??? ?? ???? + C =? ?????? ?? ???? + C
? ?????? ? ?? ? 3tan2? ?? Example 11: 0 = ? Convert tan2x to sec2x 1. ? ? = ???? ?? = ???? ? ? ? ???? ? ? ? = ? ? ? = ? ? ? ?.????
Product to Sum Identities = ? Example 12: cos 2? cos 6? ?? ???? ?? ?? + ??? ?? + ?? ?? ???? ?? +??? ?? ?? = ? Cosine is an even function cos(-x) = cos(x) = ? ???? ?? + ??? ?? ?? = ? ? ???? ?? +? ???? ??+ C ? = ? ? ????? ?? + C ???? ?? +