Understanding Related Rates in Calculus
Related rates problems in calculus involve finding the rate of change of one quantity with respect to another, utilizing concepts of implicit differentiation, chain rule, and relevant formulas. This includes scenarios such as ripples in a pond, inflating balloons, tracking airplanes, and filling tanks, where rates of change are interconnected. By assigning symbols, writing equations, differentiating, and solving, one can determine the desired rates of change.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
Chapter 2.6: Chapter 2.6: Related Rates Related Rates HONORS CALCULUS/CALCULUS HONORS CALCULUS/CALCULUS
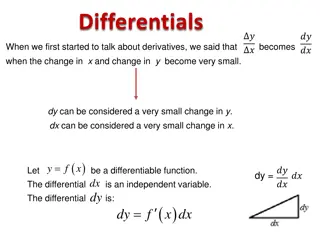
Related rate problems are differentiated with respect to time. This means every variable, except t is a differentiated implicitly. Ex.) Given ? = ??+ ?, find ?? ?? when ? = ?, given that ?? ??= ?.
Related Rate Problems 1. Assign symbols to all given quantities and quantities to be determined. Make a sketch and label the quantities if possible. 2. Write an equation involving the variables whose rates of change are give or are to be determined. 3. Using the Chain Rule, implicitly differentiate both sides of the equation with respect to ?. 4. Substitute into the resulting equation all known values for the variables and their rates of change. 5. Solve for the required rate of change.
Frequently Used Formulas Pythagorean Theorem: ??+ ??= ?? Volume of a Cone: ? =? ????? Area of a Circle: ? = ??? Volume of a Sphere: ? =? ????
Ex.) A pebble is dropped into a calm pond, causing ripples in the form of concentric circles. The radius r of the outer ripple is increasing at a constant rate of 1 foot per second. When the radius is 4ft., what rate is the total area A of the disturbed water increasing?
Ex.) Air if being pumped into a spherical balloon at the rate of ?.? ??? ??? ??????. Find the rate of change of the radius when the radius is 2 inches.
The Velocity of an Airplane Tracked by Radar An airplane is flying at an elevation of 6 miles on a flight path that will take it directly over a radar tracking station. Let s represent the distance (in miles) between the radar station and the plane. If s is decreasing at a rate of 400 miles per hour when s is 10 miles, what is the velocity of the plane?
Ex.) A conical tank (with vertex down) is 10 ft. across the top and 12 feet deep. If water is flowing into the tank at a rate of 10 cubic feet per minute, find the rate of change of the depth of the water when the water is 8 feet deep.
?? ?????? ?? ??= ?? ?? ??=? ? = ? = ?