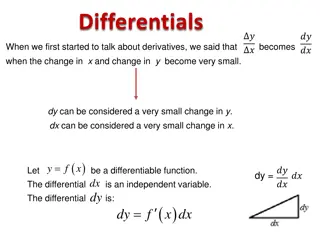
Understanding Points of Inflection in Calculus
Points of inflection in calculus refer to points where the curve changes from convex to concave or vice versa. These points are identified by observing changes in the curve's concavity, and they are not always stationary points. A stationary point can be a point of inflection, but not all points of inflection are stationary. By analyzing the gradient before and after a point, we can determine the type of stationary point, whether it is a local maximum, minimum, or a point of inflection.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
Points of Inflection Maximum and minimum stationary points are often called turning points because the curve turns as its gradient changes from positive to negative or from negative to positive. A stationary point can also be a point of inflection.
A point of inflection is where the curve changes from convex to concave (or vice versa). The line curves in one direction before the point of inflection, then curves in the other direction after. concave convex ? ? = 0 (the same terms used in optics!) Technically we could label these either way round depending on where we view the curve from. What s important is that the concavity changes.
A stationary point can be a point of inflection but a point of inflection is not always a stationary point. When a point of inflection is stationary it is called a stationary (or horizontal) point of inflection or saddle point. A point of inflection is not a turning point because the gradient doesn t change sign as we move from one side of the point to the other. Not all points of inflection are stationary points, as can be seen in the example on the right. A point of inflection which is a stationary point is known as a saddle point.
Stationary point of inflection ( saddle point ) Non-stationary point of inflection A point of inflection is a point where the curve changes from concave to convex (or vice versa). We can see that when ?? but a point of inflection instead. At Level 2, you won t see non-stationary points of inflection. ??= 0, we might not have a maximum or minimum,
What type of stationary point? Method 1: Look at gradient just before and just after point. Local Maximum Gradient just before Gradient at maximum Gradient just after ? ? ? +ve 0 +ve Point of Inflection Local Minimum Gradient just before Gradient at p.o.i Gradient just after Gradient just before Gradient at minimum Gradient just after ? ? ? ? ? ? +ve 0 +ve -ve 0 +ve
What type of stationary point? Method 1: Look at gradient just before and just after point. Find the stationary point on the curve with equation ? = ?4 32?, and determine whether it is a local maximum, a local minimum or a point of inflection. ?? ??= 4?3 32 = 0 ? = 2 ? = 48 Stationary point is 2, 48 ? Turning Point ? Strategy: Find the gradient for values just before and after ? = 2. Let s try ? = 1.9 and ? = 2.1. ? = ?.? ? = ? ? = ?.? 4.56 0 5.04 Gradient Shape ? Determine point type Looking at the shape, we can see that 2, 48 is a minimum.
Using the second derivative The method of substituting values of ? just before and after is a bit cumbersome. It also has the potential for problems: what if two different types of stationary points are really close together? 0 Recall the gradient gives a measure of the rate of change of ?, i.e. how much the ? value changes as ? changes. gradient -ve +ve gradient gradient Thus by differentiating the gradient function, the second derivative tells us the rate at what the gradient is changing. At a maximum point, we can see that as ? increases (left to right), the gradient starts out as a high positive value, gradually decreasing to a low positive value, then zero, before becoming a negative value. As such, the gradient function is decreasing and thus has a negative value ?2? ??2< 0 Thus if the second derivative is positive, the gradient is increasing. If the second derivative is negative, the gradient is decreasing.
Using the second derivative At a stationary point ? = ?: If ? ? > 0 the point is a local minimum. If ? ? < 0 the point is a local maximum. If ? ? = 0 it could be any type of point, so resort to Method 1. The stationary point of ? = ?4 32? is 2, 48 . Use the second derivative to classify this stationary point. ?? ??= 4?3 32 Find ?2? stationary point. ??2 for the ? at the ?2? ??2= 12?2 ? When ? = 2,?2? ??2= 12 22> 0 Therefore the stationary point is a minimum point.