Understanding Rates of Change and Calculus Concepts
Exploring the concept of rates of change through examples like finding the equation of a line passing through given points and understanding differentiation to calculate gradients and speeds. The relationship between gradients, curves, and tangents is highlighted to illustrate how calculus helps in analyzing changing variables over time.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
Starter: Past paper question Find the equation of the line through the points (1,5) (6, 15)
The Gradient of a Curve To understand the meaning of calculus To be able to differentiate using standard results To find the gradient of a function
Rates of Change This graph shows the distance that a car travels over a period of 5 seconds. The gradient of the graph tells us the rate at which the distance changes with respect to time. distance (m) 40 In other words, the gradient tells us the speed of the car. 0 time (s) 5 change in distance change in time 40= 5 gradient = = 8 m/s The car in this example is travelling at a constant speed since the gradient is the same at every point on the graph.
Rates of Change In most situations, however, the speed will not be constant and the distance time graph will be curved. For example, this graph shows the distance time graph as the car moves off from rest. The speed of the car, and therefore the gradient, changes as you move along the curve. To find the rate of change in speed we need to find the gradient of the curve. The process of finding the rate at which one variable changes with respect to another is called differentiation. In most situations this involves finding the gradient of a curve. distance (m) 0 time (s)
Gradient of a curve The gradient of a curve at a point is given by the gradient of the tangent at that point. Look at how the gradient changes as we move along a curve:
Gradient of a curve at a point The gradient of a curve at a point is given by the gradient of the tangent at that point. e.g. 2 y = = x 12 (2, 4)x 3 ( 4 2 , ) Tangent at 12= = The gradient of the tangent at (2, 4) is So, the gradient of the curve at (2, 4) is 4 = = m 4 3
Gradient of a curve at a point The gradient of a curve at a point is given by the gradient of the tangent at that point. e.g. 2 y = = 6 x = = m The gradient changes as we move along a curve
Gradient of a curve at a point The gradient of a curve at a point is given by the gradient of the tangent at that point. e.g. 2 y = = x = = m 2 The gradient changes as we move along a curve
Gradient of a curve at a point The gradient of a curve at a point is given by the gradient of the tangent at that point. e.g. 2 y = = x = = m 0 The gradient changes as we move along a curve
Gradient of a curve at a point The gradient of a curve at a point is given by the gradient of the tangent at that point. e.g. 2 y = = x = = m 2 The gradient changes as we move along a curve
Gradient of a curve at a point The gradient of a curve at a point is given by the gradient of the tangent at that point. e.g. 2 y = = x = = m 4 The gradient changes as we move along a curve
Gradient of a curve at a point The gradient of a curve at a point is given by the gradient of the tangent at that point. e.g. 2 y = = x = = m 6 The gradient changes as we move along a curve
Gradient of a curve at a point 2 For the curve we have the following gradients: x y = = Point on the curve 3 Gradient ( , ) 9 6 4 2 0 2 4 6 2 1 ( ( ( ( ( ( , ) 4 ) 1 , ) 0 ) 1 ) 4 ) 9 At every point, the gradient is twice the x-value , 0 1 2 3 , , ,
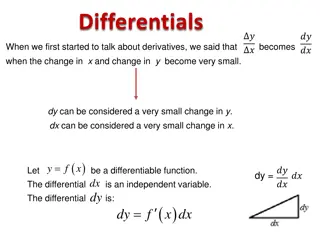
Gradient of a curve at a point 2 At every point on the gradient is twice the x-value y = = x dy = = x 2 This rule can be written as dx The notation comes from the idea of the gradient of a line being the ifference in the -values the ifference in the - values d d d d y y x x dy dx represents the derivative of y with respect to x. The function giving the gradient of a curve is called the gradient function
Using the notation This notation can be adapted for other variables so, for example: ds dt If s is distance and t is time then we can interpret this as the rate of change in distance with respect to time. In other words, the speed. represents the derivative of s with respect to t. Also, if we want to differentiate 2x4 with respect to x, for example, we can write: d dx 4 (2 ) x We could work this out by differentiating from first principles, but in practice this is unusual.
The General Rule If we differentiate from first principles we would obtain the following results: x2 x3 x4 x5 x6 y x dy dx 2x 3x2 4x3 5x4 6x5 1 What pattern do you notice?
The General Rule Here s some different notation and different examples. Can you write down a general rule? ? ? = 3?2+ 2? ? ? = 5?3 7 ? ? = 15?2 ? ? = 6? + 2 ? ? = ???+ ?, ? ? = ???? 1 ? ? = ?12 7?3 ? ? = 2? 4 ? ? = 12?11 21?2 ? ? = 8? 5
Notation NOTE: If a function is y = Then the gradient function is dy/dx= If the function is f(x) = Then the gradient function is f (x) = You must be consistent with what the question uses.