Understanding Reciprocal Lattices in Crystal Structures
Explore the concept of reciprocal lattices in crystal structures, including non-rectangular lattices, Fourier space in 2D, Wigner-Seitz cells, Brillouin Zones, and the relationship between BCC and FCC structures. Learn about primitive lattice vectors, construction of the Brillouin Zone, and the significance of reciprocal space in crystallography.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
Group: What happens if the lattice is not rectangular? Determine the reciprocal lattice for: a2 b2 a1 b1 Real space Fourier (reciprocal) space In 2D, reciprocal vectors are perpendicular to opposite axis.
The reciprocal lattice in one dimension a x Real lattice - /a /a What is the range of unique environments? Reciprocal lattice k 2 /a 4 /a -6 /a -4 /a -2 /a 0 Does this look familiar to anything? Weigner Seitz Cell: Smallest space enclosed when intersecting the midpoint to the neighboring lattice points. Why don t we include second neighbors here (do in 2D/3D)?
The Brillouin Zone (BZ) - /a /a Reciprocal lattice k 2 /a 4 /a -6 /a -4 /a -2 /a 0 Is defined as the Wigner-Seitz primitive cell in the reciprocal lattice (smallest unique set of distance/area/volume in reciprocal space) Its construction exhibits all the wavevectors k which can be Bragg-reflected by the crystal Also critical for understanding energy diagrams
Group: Find the reciprocal lattice vectors of BCC A B C D E F B F E C D C A F A E B C a a The primitive lattice vectors for BCC are: b b = 2 a 3 a 2 ( ) 1 a 1 2 3 The volume of the primitive cell is a3(2 pts./unit cell) So, the primitive translation vectors in reciprocal space are: Look familiar? Good websites: http://newton.umsl.edu/run//nano/reltutor2.html http://matter.org.uk/diffraction/geometry/plane_reciprocal_lattices.htm
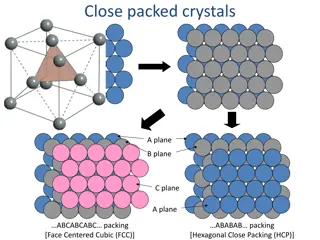
Reciprocal Lattices to SC, FCC and BCC Primitive Direct lattice = y a a 3 Reciprocal lattice ( ( ( = b / 2 3 Volume of BZ ( / 2 ) ) ) )3 = a x b x 2 / a a a 1 1 SC = = b y 2 / a a 2 2 Direct Reciprocal = z a z a Simple cubic Simple cubic bcc fcc ( ( ( ) ) ) ( ( ( ) a 2 2 1 = + = + x y b y z a x y z a fcc bcc 1 1 BCC 2 ) a 2 ( )3 1 = x + y + z = + a z b x z a 2 2 / a 2 2 2 ) a 1 = + = + a b x y a 3 3 2 ( ( ( ) ( ( ( ) ) ) a 2 2 1 = x + y z = + b x y z a x y a 1 1 2 FCC ) ) a 2 1 = + = + b a y z a 2 2 2 ( )3 a 1 = + = + a z x b x y z a 4 2 / a 3 3 2
Wigner-Seitz cells and Brillouin Zones What type of crystal is the BZ cutout? The reciprocal lattice of BCC if FCC, so the BZ of bcc looks like FCC and vice versa.
Nomenclature Usually, it is sufficient to know the energy En(k) curves - the dispersion relations - along the major directions. Directions are chosen that lead aong special symmetry points. These points are labeled according to the following rules: Points (and lines) inside the Brillouin zone are denoted with Greek letters. Points on the surface of the Brillouin zone with Roman letters. The center of the Wigner-Seitz cell is always denoted by a
Brillouin Zones in 3D fcc bcc Note: bcc lattice in reciprocal space is a fcc lattice hcp Note: fcc lattice in reciprocal space is a bcc lattice Construction leads to primitive unit cell in rec. space The BZ reflects lattice symmetry (some argue that most of solid state physics can be understood by understanding symmetry)
Brillouin Zone of Silicon Symbol Description Center of the Brillouin zone Simple Cubic Center of an edge Corner point Center of a face FCC Middle of an edge joining two hexagonal faces Center of a hexagonal face C6 Middle of an edge joining a hexagonal and a square face Corner point Center of a square face C4 BCC M R X K L U W X Points of symmetry on the BZ are important (e.g. determining bandstructure). Electrons in semiconductors are perturbed by the potential of the crystal, which varies across unit cell. H Corner point joining 4 edges N P Center of a face Corner point joining 3 edges
Now that we know how to construct reciprocal lattice vectors and BZs, let s get back to how that helps us understand diffraction.
XRD allows Crystal Structure Determination LATTICE TYPE What do we need to know in order to define the crystal structure? The lattice parameters and the lattice type What do we need to know in order to define the crystal structure? -The lattice parameters and the lattice type PbS
XRD allows Crystal Structure Determination POSITION OF PEAKS LATTICE TYPE What do we need to know in order to define the crystal structure? The lattice parameters and the lattice type (this defines the positions of diffraction spots) What do we need to know in order to define the crystal structure? -The lattice parameters and the lattice type (this defines the positions of diffraction spots) PbS
XRD allows Crystal Structure Determination POSITION OF PEAKS LATTICE TYPE WIDTH OF PEAK INTENSITY OF PEAKS What do we need to know in order to define the crystal structure? The lattice parameters and the lattice type (this defines the positions of diffraction spots) What do we need to know in order to define the crystal structure? -The lattice parameters and the lattice type (this defines the positions of diffraction spots) PbS
XRD allows Crystal Structure Determination POSITION OF PEAKS LATTICE TYPE PERFECTION OF LATTICE WIDTH OF PEAK INTENSITY OF PEAKS What do we need to know in order to define the crystal structure? The lattice parameters and the lattice type (this defines the positions of diffraction spots) What do we need to know in order to define the crystal structure? -The lattice parameters and the lattice type (this defines the positions of diffraction spots) PbS
XRD allows Crystal Structure Determination POSITION OF PEAKS LATTICE TYPE PERFECTION OF LATTICE WIDTH OF PEAK POSITION OF ATOMS IN BASIS INTENSITY OF PEAKS What do we need to know in order to define the crystal structure? The lattice parameters and the lattice type (this defines the positions of diffraction spots) The atom type at each point (these define the intensity of diffraction spots) What do we need to know in order to define the crystal structure? -The lattice parameters and the lattice type (this defines the positions of diffraction spots) -The atom type at each point (these define the intensity of diffraction spots) PbS
XRD allows Crystal Structure Determination POSITION OF PEAKS LATTICE TYPE PERFECTION OF LATTICE WIDTH OF PEAK POSITION OF ATOMS IN BASIS INTENSITY OF PEAKS What do we need to know in order to define the crystal structure? The lattice parameters and the lattice type (this defines the positions of diffraction spots) The atom type at each point (these define the intensity of diffraction spots) Conclusion: If we measure positions and intensities of many spots, then we should be able to determine the crystal structure. What do we need to know in order to define the crystal structure? -The lattice parameters and the lattice type (this defines the positions of diffraction spots) -The atom type at each point (these define the intensity of diffraction spots) Conclusion: If we measure positions and intensities of many spots, then we should be able to determine the crystal structure. PbS
To get a diffraction peak, K has to be a reciprocal lattice vector, but even if K is, f(r)e-ir K might still be zero! ' k K 2 o k
To get a diffraction peak, K has to be a reciprocal lattice vector, but even if K is, f(r)e-ir K might still be zero! The scattered x-ray amplitude is proportional to: atoms all ( r r K K ) i A f e i i ' k K 2 o k
To get a diffraction peak, K has to be a reciprocal lattice vector, but even if K is, f(r)e-ir K might still be zero! The scattered x-ray amplitude is proportional to: lattice basis atoms all ( K K r r ( r r K K = ) i ) i f e A f e i hkl i i i ' k K 2 o k Can break this sum into a sum over all lattices and a sum over all of the atoms within the basis.
To get a diffraction peak, K has to be a reciprocal lattice vector, but even if K is, f(r)e-ir K might still be zero! Structure Factor Shklgives intensity of peaks The scattered x-ray amplitude is proportional to: lattice basis atoms all ( K K r r ( r r K K = ) i ) i f e A f e i hkl i i i ' k S Structure factor hkl K 2 o k Can break this sum into a sum over all lattices and a sum over all of the atoms within the basis.
i ( r r = ) i K S f e i hkl r K hkl i r = n1a1 + n2a2 + n3a3 (real space) = + + K hb kb lb 1 2 3 b b a = 2 i j ij
i ( r r = ) i K S f e i hkl r K hkl i r = n1a1 + n2a2 + n3a3 (real space) = + + K hb kb lb 1 2 3 Different h,k,l b b a = 2 i j ij S Cubic form: hkl Works for any conventional cubic cell Where xi, yi and zi are the lattice positions of the atoms in the basis. h, k and l are the miller indices of different planes in the crystal.
Group: Simple Cubic Lattice S hkl Simplify the structure factor for the simple cubic lattice for a one atom basis. Just let f be a constant. fiexp 2 i hxi+kyi+lzi ( ) i F= hkl S ( ) One atom basis: r= 0,0,0 = f exp 2 i 0 ( ) = f exp 0 F= f exp 2 i 0 h+0 k+0 l ( hkl ) S = f Where xi, yi and zi are the lattice positions of the atoms in the basis.
Group: Simple Cubic Lattice S hkl Simplify the structure factor for the simple cubic lattice for a one atom basis. Just let f be a constant. fiexp 2 i hxi+kyi+lzi ( ) i F= hkl S ( ) One atom basis: r= 0,0,0 = f exp 2 i 0 ( ) = f exp 0 F= f exp 2 i 0 h+0 k+0 l ( hkl ) S = f Where xi, yi and zi are the lattice positions of the atoms in the basis.
Group: Simple Cubic Lattice S hkl Simplify the structure factor for the simple cubic lattice for a one atom basis. Just let f be a constant. fiexp 2 i hxi+kyi+lzi ( ) i F= hkl S ( ) One atom basis: r= 0,0,0 = f exp 2 i 0 ( ) = f exp 0 F= f exp 2 i 0 h+0 k+0 l ( hkl ) S = f Where xi, yi and zi are the lattice positions of the atoms in the basis.
Group: Simple Cubic Lattice S hkl Simplify the structure factor for the simple cubic lattice for a one atom basis. Just let f be a constant. fiexp 2 i hxi+kyi+lzi ( ) i F= hkl S ( ) One atom basis: r= 0,0,0 = f exp 2 i 0 ( ) = f exp 0 F= f exp 2 i 0 h+0 k+0 l ( hkl ) S = f Where xi, yi and zi are the lattice positions of the atoms in the basis.
How Do We Determine The Lattice Constant? 2 = = = ) 0 ( i 2 For the simple cubic lattice with a one atom basis: S f e f I S f hkl hkl hkl So the x-ray intensity is nonzero for all values of (hkl), subject to the Bragg condition, which can be expressed . = 2 sin d hkl
How Do We Determine The Lattice Constant? 2 = = = ) 0 ( i 2 For the simple cubic lattice with a one atom basis: S f e f I S f hkl hkl hkl So the x-ray intensity is nonzero for all values of (hkl), subject to the Bragg condition, which can be expressed . = 2 sin d hkl a = dhkl We know for cubic lattices (a=b=c): ( ) / 1 2 + + 2 2 2 h k l
How Do We Determine The Lattice Constant? 2 = = = ) 0 ( i 2 For the simple cubic lattice with a one atom basis: S f e f I S f hkl hkl hkl So the x-ray intensity is nonzero for all values of (hkl), subject to the Bragg condition, which can be expressed . = 2 sin d hkl a = dhkl We know for cubic lattices (a=b=c): ( ) / 1 2 + + 2 2 2 h k l = ( ) 2 + + 2 2 2 2 sin h k l Substituting and squaring both sides: 2 4 a
How Do We Determine The Lattice Constant? 2 = = = ) 0 ( i 2 For the simple cubic lattice with a one atom basis: S f e f I S f hkl hkl hkl So the x-ray intensity is nonzero for all values of (hkl), subject to the Bragg condition, which can be expressed . = 2 sin d hkl a = dhkl We know for cubic lattices (a=b=c): ( ) / 1 2 + + 2 2 2 h k l = ( ) 2 + + 2 2 2 2 sin h k l Substituting and squaring both sides: 2 4 a Thus, if we know the x-ray wavelength and are given (or can measure) the angles at which each diffraction peak occurs, we can determine a for the lattice! How?
How Do We Determine The Lattice Constant? 2 = = = ) 0 ( i 2 For the simple cubic lattice with a one atom basis: S f e f I S f hkl hkl hkl So the x-ray intensity is nonzero for all values of (hkl), subject to the Bragg condition, which can be expressed . = 2 sin d hkl a = dhkl We know for cubic lattices (a=b=c): ( ) / 1 2 + + 2 2 2 h k l = ( ) 2 + + 2 2 2 2 sin h k l Substituting and squaring both sides: 2 4 a Thus, if we know the x-ray wavelength and are given (or can measure) the angles at which each diffraction peak occurs, we can determine a for the lattice! How? 2 sin + + 2 2 2 h k l
Missing Spots in the Diffraction Pattern In some lattices, the arrangement and spacing of planes produces diffractions from planes that are always exactly 180 out of phase causing a phenomenon called extinction. Can you think of an example? = /2
Missing Spots in the Diffraction Pattern In some lattices, the arrangement and spacing of planes produces diffractions from planes that are always exactly 180 out of phase causing a phenomenon called extinction. For the BCC lattice the (100) planes are interweaved with an equivalent set at the halfway position, giving a reflection exactly out of phase, which exactly signal. Can you think of an example? cancel the
Extinction in BCC (001)
Extinction in BCC (-101) (001) What about the 101 family of planes?
Extinction in BCC Let s see how this shows up in the structure factor (-101) (001) What about the 101 family of planes?
The Structure Factor of BCC What values of hkl do not have diffraction peaks? i r = iK S f e hkl hkl i ( ( ( ) ) ) ( ( ( ) a 2 2 1 = + = + x y b y z a x y z a 1 1 2 ) a 2 1 = + = x + y + z b x z a z a 2 2 2 ) a 1 = + = + a b x y a 3 3 2
Group: The Structure Factor of BCC What values of hkl do not have diffraction peaks? Analysis of more than one lattice point per conventional unit cell E.g: bcc and fcc lattices i hkl S r = iK S f e hkl hkl i ( ( ( ) ) ) ( ( ( ) a 2 2 1 = + = + x y b y z a x y z a 1 1 2 ) a 2 1 = + = x + y + z b x z a z a 2 2 2 ) a 1 = + = + a b x y a 3 3 2
Group: The Structure Factor of BCC What values of hkl do not have diffraction peaks? Analysis of more than one lattice point per conventional unit cell E.g: bcc and fcc lattices i hkl S r = iK S f e hkl hkl i bcc conventional lattice has two atoms per unit cell located at r1 = (0,0,0) and r2 = (1/2,1/2,1/2)
Group: Find the structure factor for BCC. Under what h,k,l is it non-zero? S hkl 1 2,1 2,1 ( ) & r= Two atom basis: r= 0,0,0 2 2 h+1 +exp 2 i1 2 k+1 F= f exp 2 i 0 h+0 k+0 l ( = f 1+exp i h+k+l h, k & l are integers, so h+k+l=N (where N is an integer) The exponential can then take one of two values: exp i h+k+l ( ) =+1 if N=even exp i h+k+l ( ) = 1 if N=odd So: F =2f if N=even hkl S ) S 2 l hkl ( ) F =0 if N=odd hkl S
Group: Find the structure factor for BCC. Under what h,k,l is it non-zero? S hkl 1 2,1 2,1 ( ) & r= Two atom basis: r= 0,0,0 2 2 h+1 +exp 2 i1 2 k+1 F= f exp 2 i 0 h+0 k+0 l ( = f 1+exp i h+k+l h, k & l are integers, so h+k+l=N (where N is an integer) The exponential can then take one of two values: exp i h+k+l ( ) =+1 if N=even exp i h+k+l ( ) = 1 if N=odd So: F =2f if N=even hkl S ) S 2 l hkl ( ) F =0 if N=odd hkl S
Group: Find the structure factor for BCC. Under what h,k,l is it non-zero? S hkl 1 2,1 2,1 ( ) & r= Two atom basis: r= 0,0,0 2 2 h+1 +exp 2 i1 2 k+1 F= f exp 2 i 0 h+0 k+0 l ( = f 1+exp i h+k+l h, k & l are integers, so h+k+l=N (where N is an integer) The exponential can then take one of two values: exp i h+k+l ( ) =+1 if N=even exp i h+k+l ( ) = 1 if N=odd So: F =2f if N=even hkl S ) S 2 l hkl ( ) F =0 if N=odd hkl S
Group: Find the structure factor for BCC. Under what h,k,l is it non-zero? S hkl 1 2,1 2,1 ( ) & r= Two atom basis: r= 0,0,0 2 2 h+1 +exp 2 i1 2 k+1 F= f exp 2 i 0 h+0 k+0 l ( = f 1+exp i h+k+l h, k & l are integers, so h+k+l=N (where N is an integer) The exponential can then take one of two values: exp i h+k+l ( ) =+1 if N=even exp i h+k+l ( ) = 1 if N=odd So: F =2f if N=even hkl S ) S 2 l hkl ( ) F =0 if N=odd hkl S
Group: Find the structure factor for BCC. Under what h,k,l is it non-zero? S hkl 1 2,1 2,1 ( ) & r= Two atom basis: r= 0,0,0 2 2 h+1 +exp 2 i1 2 k+1 F= f exp 2 i 0 h+0 k+0 l ( = f 1+exp i h+k+l h, k & l are integers, so h+k+l=N (where N is an integer) The exponential can then take one of two values: exp i h+k+l ( ) =+1 if N=even exp i h+k+l ( ) = 1 if N=odd So: F =2f if N=even hkl S ) S 2 l hkl ( ) F =0 if N=odd hkl S
Visualizing the structure factor for BCC Allowed low order reflections are: 110, 200, 112, 220, 310, 222, 321, 400, 330, 411, 420 Draw lowest on this cube -> Forbidden reflections are: 100, 111, 210 Due to identical plane of atoms halfway between causes destructive interference Real bcc lattice has an fcc reciprocal lattice (this is a good trick for remembering the rule) This kind of argument leads to rules for identifying the lattice symmetry from "missing" reflections. 022 002 112 011 202 121 000 101 020 211 110 220 200
How to determine lattice parameter this time? For a bcc lattice with a one atom basis, the x-ray intensity is nonzero for all planes (hkl), subject to the Bragg condition, except for the planes where h+k+l is odd. Thus, diffraction peaks will be observed for the following planes: (100) (110) (111) (200) (210) (211) (220) (221) (300)
How to determine lattice parameter this time? For a bcc lattice with a one atom basis, the x-ray intensity is nonzero for all planes (hkl), subject to the Bragg condition, except for the planes where h+k+l is odd. Thus, diffraction peaks will be observed for the following planes: (100) (110) (111) (200) (210) (211) (220) (221) (300) Just as before, if we are given or can measure the angles at which each diffraction peak occurs, we can graphically determine a for the lattice! 2 sin + + 2 2 2 h k l
How to determine lattice parameter this time? For a bcc lattice with a one atom basis, the x-ray intensity is nonzero for all planes (hkl), subject to the Bragg condition, except for the planes where h+k+l is odd. Thus, diffraction peaks will be observed for the following planes: (100) (110) (111) (200) (210) (211) (220) (221) (300) Just as before, if we are given or can measure the angles at which each diffraction peak occurs, we can graphically determine a for the lattice! 2 sin + + 2 2 2 h k l A similar analysis can be done for a crystal with the fcc lattice with a one atom basis. For materials with more than one of the same atom type per basis in a cubic lattice, the rules for the structure factor can be modified.
How to determine lattice parameter this time? For a bcc lattice with a one atom basis, the x-ray intensity is nonzero for all planes (hkl), subject to the Bragg condition, except for the planes where h+k+l is odd. Thus, diffraction peaks will be observed for the following planes: (100) (110) (111) (200) (210) (211) (220) (221) (300) Just as before, if we are given or can measure the angles at which each diffraction peak occurs, we can graphically determine a for the lattice! 2 sin What if you don t know the h k l values? + + 2 2 2 h k l A similar analysis can be done for a crystal with the fcc lattice with a one atom basis. For materials with more than one of the same atom type per basis in a cubic lattice, the rules for the structure factor can be modified.