Understanding Rational Exponents and Nth Roots
Learn about rational exponents, nth roots, even and odd exponents, evaluating expressions, rules for rational exponents, simplifying radicals, and more in this informative content with visual examples.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
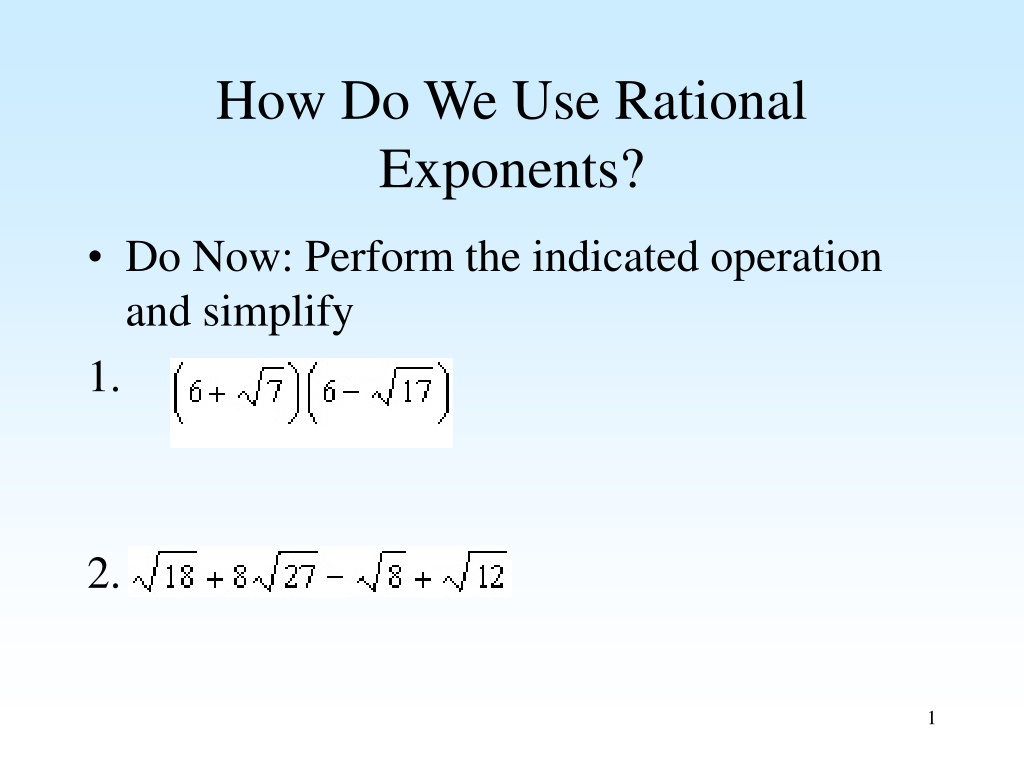
How Do We Use Rational Exponents? Do Now: Perform the indicated operation and simplify 1. 2. 1
nth Roots nth Roots An nth root of number a is a number whose nth power is a. a number whose nth power is a = na If the index n is even, then the radicand a must be nonnegative. is not a real number 5 32 2 = = 4 4 16 2, 16 but 2
Square Root of x2 x = 2 x 3 Page 393
Radicals 4
When n Is Even 1 = = 100 100 10 2 1 = = 4 625 625 5 4 1 = = 6 64 64 2 6 1 ( ) = 4 4 is not yet defined 2 7
Exponent 1/n When n Is Odd 1 = = 3 27 27 3 3 1 ( ) = = 3 27 27 3 3 1 1 1 1 5 = = 5 32 32 2 9
nth Root of Zero 0 = n 0 10
Evaluating in Either Order ( ) 8 2 ( ) 8 or ( ) 2 2 2 = = = 3 4 3 2 ( ) 8 = = = 2 3 3 8 64 4 3 12
Evaluating a-m/n 1 1 1 1 2 ( ) 8 = = = = ( ) 8 3 ( ) 2 2 2 2 4 ( ) 8 3 3 14
Rules for Rational Exponents 15 7-6
Simplifying ( ) a 1 = = 6 6 y y y 6 6 1 1 ( )= ab b 3 2 16
Simplifying ( ) y 1 = = 6 6 y y 6 6 1 1 1 1 ( ) ( ) = 1 1 a b ab a b a b 3 3 2 2 1 1 + + 1 1 = a b 3 2 2 3 = a b 3 2 17
Simplifying ( ) a 1 = = 6 6 y y y 6 6 1 2 1 3 ( ) = b ab a b 3 3 2 2 ( ) = 1 8 10 12 9 x y z 2 18
Multiplying Radicals Different Indices 1 1 1 1 3 + = = = = = 3 4 4 4 2 2 2 2 2 2 2 8 4 2 4 2 4 = 3 2 3 19
Multiplying Radicals Different Indices 1 1 1 1 3 + = = = = = 3 4 4 4 2 2 2 2 2 2 2 8 4 2 4 2 4 1 1 = = 3 2 3 2 3 3 2 20
Different Indices 1 1 1 1 3 + = = = = = 3 4 4 4 2 2 2 2 2 2 2 8 4 2 4 2 4 1 2 3 1 = = = 3 2 3 2 3 2 3 3 6 6 2 21
Different Indices 1 1 1 1 3 + = = = = = 3 4 4 4 2 2 2 2 2 2 2 8 4 2 4 2 4 1 2 3 1 = = = = 6 2 3 6 3 2 3 2 3 2 3 2 3 3 6 6 2 22
Different Indices 1 1 1 1 3 + = = = = = 3 4 4 4 2 2 2 2 2 2 2 8 4 2 4 2 4 1 2 3 1 = = = = 6 2 3 6 3 6 2 3 2 3 2 3 2 3 108 3 6 6 2 23
Rational Exponents Eliminate the root, then the power 2 = 2 a 3 24
Eliminate the Root, Then the Power 2 = 2 a 3 3 2 = 3 2 a 3 = 2 8 a = 2 8 a = 2 2 a CHECK 25
Negative Exponents 2 ( ) = 1 1 r 3 26
Negative Exponents Eliminate the root, then the power ( ) r 1 = 2 1 3 3 2 ( ) = 3 1 1 r 3 ( ) 2 = 1 1 r ( ) 2 = 1 1 r = 1 1 r = = 2 0 r r 27 CHECK
Negative Exponents Eliminate the root, then the power 2 ( ) = 2 3 1 t 3 28
No Solution Eliminate the root, then the power 2 ( ) = 2 3 1 t 3 3 2 ( ) ( ) 3 = 2 3 1 t 3 ( ) 2 = 2 3 1 t ( ) 2 = 2 3 1 t 29
No Solution Eliminate the root, then the power 2 ( ) = 2 3 1 t 3 3 2 ( ) ( ) 3 = 2 3 1 t 3 ( ) 2 = 2 3 1 t ( ) 2 = 2 3 1 t No real solution 30
Strategy for Solving Equations with Exponents and Radicals 31
This powerpoint was kindly donated to www.worldofteaching.com http://www.worldofteaching.com is home to over a thousand powerpoints submitted by teachers. This is a completely free site and requires no registration. Please visit and I hope it will help in your teaching. 32