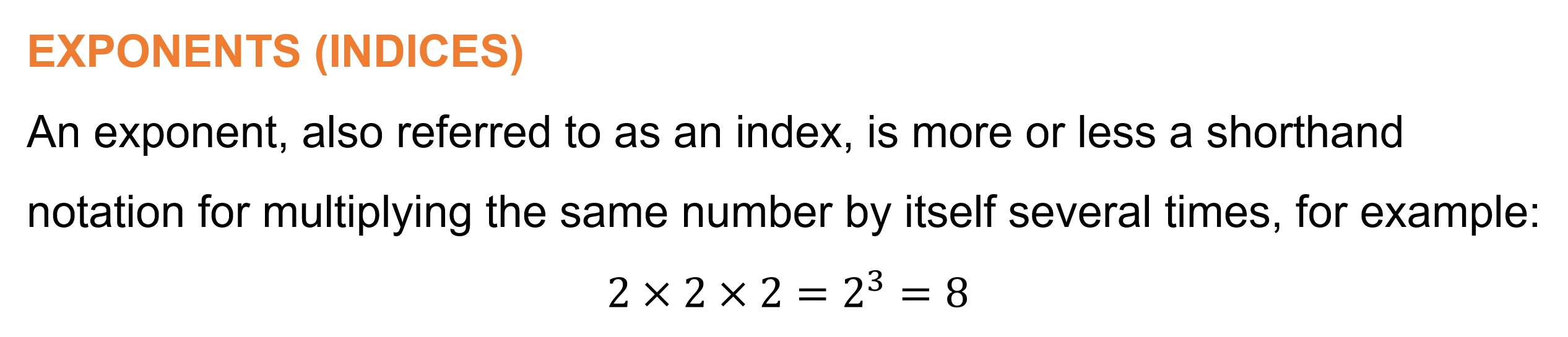Mathematics Modules Overview: Exponents, Logarithms, Factorisation, and Equations
Understanding key mathematical concepts such as exponents, logarithms, factorisation, and equations is crucial for building a strong foundation in mathematics. This overview covers the basics of exponents and logarithms, factorisation and algebraic fractions, highest common factor and lowest common multiple, and linear equations with practical examples and illustrations.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
Mathematics N2
Module 1: Exponents and logarithms EXPONENTS (INDICES) An exponent, also referred to as an index, is more or less a shorthand notation for multiplying the same number by itself several times, for example: 2 2 2 = 23= 8 www.futuremanagers.com
Module 1: Exponents and logarithms (continued) LOGARITHMS Logarithms are the opposite of exponentials. The logarithm of a number is the exponent by which another fixed value, the base, must be raised to produce that number. Therefore a logarithm is an unknown exponent that will be determined. For example: The logarithm of 100 to base 10 is 2, written as ???10100 = 2 www.futuremanagers.com
Module 2: Factorisation, HCF, LCM and algebraic fractions FACTORISATION Factorisation is he process of writing a mathematical statement as a product of its factors. For example: ?? + ?? + ?? = ?(? + ? + ?) ? and (? + ? + ?) are factors of the three terms ?? + ?? + ?? By expanding ?(? + ? + ?) you get the following: ?(? + ? + ?) = ?? + ?? + ?? www.futuremanagers.com
Module 2: Highest common factor and lowest common multiple (continued) HIGHEST COMMON FACTOR AND LOWEST COMMON MULTIPLE The highest common factor (HCF) of two or more numbers is the common factor of all those numbers with the greatest value. The lowest common multiple (LCM) of two or more numbers is the smallest possible number into which all the numbers can be divided exactly. www.futuremanagers.com
Module 2: Highest common factor and lowest common multiple (continued) ALGEBRAIC FRACTIONS An algebraic fraction is a fraction where the numerator and denominator are algebraic expressions. For example: 3?? ? = 3? In this example, the ? in the numerator cancels with the ? in the denominator, which leaves the equation with 3?. www.futuremanagers.com
Module 3: Equations, word problems and manipulation of technical formulae LINEAR EQUATIONS A linear equation is an algebraic equation in which each term is either a constant or the product of a constant and (the first power of) a single variable. The power of the highest variable is 1. Linear equations could have one or more variables. www.futuremanagers.com
Module 3: Equations, word problems and manipulation of technical formulae (continued) QUADRATIC EQUATIONS A quadratic equation is any equation in the form ??2+ ?? + ? = 0, where ? represents an unknown, and ?,?, and ? are constants which are not equal to 0. www.futuremanagers.com
Module 3: Equations, word problems and manipulation of technical formulae (continued) SIMULTANEOUS LINEAR EQUATIONS Sometimes there are two unknowns in one equation. For example: In 2? + 3? = 8. If you substitute values of (? = 1) and (? = 2), they would satisfy the equation. However, (? = 2) and (? =4 3), would also satisfy the equation, as would (? = 1) and (? = 3). There are an infinite number of solutions. To avoid this, a second equation containing the same variables is required, so that there is only one solution for ? and one solution for ?. www.futuremanagers.com
Module 3: Equations, word problems and manipulation of technical formulae (continued) WORD PROBLEMS When an answer is needed to a mathematical problem that is described in words as well as numbers, it is called a word problem. You find the solution by setting up an equation that represents the problem, and then solve it. www.futuremanagers.com
Module 3: Equations, word problems and manipulation of technical formulae (continued) MANIPULATION OF TECHNICAL FORMULAE Manipulation of technical formula can also be described as changing the subject of the formula. An equation can be manipulated in such a way that the required variable is isolated on the left-hand-side, and everything else is written on the right- hand-side. This manipulation is described as making the wanted variable the subject of the formula. www.futuremanagers.com
Module 4: Algebraic graphs LINEAR GRAPHS (STRAIGHT LINE GRAPHS) A linear equation can be represented graphically by a straight line, for example: ? = 3? + 2, or ? = ?? + ?. A linear graph is a graph in which all the points representing the relationship between 2 quantities lie on a straight line. www.futuremanagers.com
Module 4: Algebraic graphs (continued) PARABOLAS A parabola can be described by a quadratic equation of the following form: ? = ??2+ ?? + ? www.futuremanagers.com
Module 4: Algebraic graphs (continued) GRAPHICAL SOLUTIONS OF SIMULTANEOUS EQUATIONS Simultaneous equations can be solved exactly using an algebraic method or approximately using the graphical method. The first step is to draw the graphs and then read the coordinates of the points where the two graphs cut each other. These points are the solutions and are called the points of intersection of the graphs. www.futuremanagers.com
Module 5: Measuring of angles, angular and peripheral velocity and sectors of circles MEASURING OF ANGLES There are several ways to measure the size of an angle. One way is to use the unit degrees. A 360 angle is a full rotation or a full circle. This is called one revolution. www.futuremanagers.com
Module 5: Measuring of angles, angular and peripheral velocity and sectors of circles (continued) RADIANS Radians are used as an alternative way of measuring angles, or the amount of turn. A radian is defined as the angle made at the centre of a circle between two radii when the arc length on the circumference between the two radii (radiuses) is equal to the length of one radius. www.futuremanagers.com
Module 5: Measuring of angles, angular and peripheral velocity and sectors of circles (continued) ANGULAR AND PERIPHERAL VELOCITY Angular velocity, also called rotational velocity, is a quantitative expression of the amount of rotation that a spinning object undergoes per unit time. The angular velocity is the speed at the centre of a moving wheel or disc, with which the revolutions are completed and is measured in radians per time unit. The Angular Velocity Formula is as follows: ? =? ?=? ? ???? ?? ? ? ????? ?? ??????? ?? ?? ???? ???? ?? ?? ????? ?? ??????? www.futuremanagers.com
Module 5: Measuring of angles, angular and peripheral velocity and sectors of circles (continued) CIRCLE SECTORS A circular sector or circle sector is the portion of a disk enclosed by two radii and an arc, where the smaller area is the minor sector and the larger area is the major sector. In the diagram, ? is the central angle in radians, ? the radius of the circle, and ? is the arc length of the minor sector. www.futuremanagers.com
Module 5: Measuring of angles, angular and peripheral velocity and sectors of circles (continued) CHORDS IN A CIRCLE A chord is a straight line drawn from one side of the circumference of a circle to the other side, dividing the circle into two parts. www.futuremanagers.com
Module 6: Trigonometry TRIGONOMETRIC RATIOS For any angle ?: ???????? ????????? or ? ? sin? = ???????? ?????????or ? cos? = ? ???????? ???????? or ? tan? = ? Remember: SOH CAH TOA www.futuremanagers.com
Module 7: Mensuration MENSURATION When calculating equations which have different units of measurement, we need to remember: Perimeter: The total distance around a shape; Circumference: The total distance around a circle; Area: The total amount of surface space occupied by a shape; Surface area: The total outside area of a shape; and Volume: The total amount of space a shape occupies. www.futuremanagers.com