Understanding Exponents and Scientific Notation
Exponents are powers to which bases are raised, allowing for efficient representation and calculation of numbers. This review covers the basics of exponents, rules, laws, and practical applications. Scientific notation offers a method to express very large or small numbers using powers of 10. Learn how to convert between decimal form and scientific notation effortlessly.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
Exponents & Scientific Notation Review and Applications Presented by the Quantitative Success Center
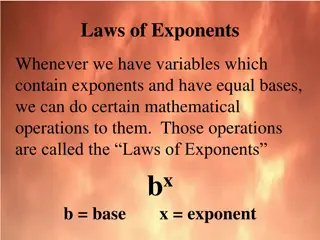
Lets remind ourselves What is an exponent? An exponent is the power which a base is raised to. Whatever your exponent is, you multiply your base by itself that many times ??= ? ? ? ? ? gets multiplied ? many times Examples: ?2= ? ? 34= 3 3 3 3 = 81 53= 5 5 5 = 125 12= 1 1 = 1 We can also use a carrot ^ to write exponents, rather than writing it in the form of base and a superscript of power. Example: ?^2 is ? ?. Can ? and ? take on any value? Yes! so what if we have negative exponents or rational (a fraction in the) exponents?
Rules of Exponents Rule Example(s) ?0= 1 80= 1 ?1= ? 7.891= 7.89 1 ?? 1 ? ?= 101 4= 1014 ? ? = 2 5= ? 2 ???= ?? 562= 56 ? 6 *a combination of rules of exponents can be used
Laws of Exponents Law Example(s) ????= ??+? 3234= 32+4= 36 ?? ??= ?? ? 32 34= 32 4= 3 2= 1 32 ?? ?= ??? 32 4= 32 4= 38 ???= ???? 3 54= 3454 =?? ?? 4 ? ? ? =34 3 5 54 *a combination of laws of exponents can be used
Lets Practice! 3.141590 201 15 3 2 3 0.7918 0.7911 0.7918 0.7911 81 10 25 251005 1 2 35 1005
Lets Practice Solving Equations! 1. Solve 2?= 16 2. Solve 3?= 27 3. Solve 2?=1 8 4. Solve 42? 3 = 13
Comparing Values, Without Evaluating 50220 vs 50217 50220 vs 50320 16 vs 4 6782vs 6782.5 301/3vs 271/3 16 vs 17
Scientific Notation Scientific notation is a way to write very small or very large numbers. ? 10? Where ? is a number between 1 and 10 and ? is any negative or positive integer in the exponent. I.e. the form for scientific notation is a number between 1 and 10 multiplied by a power of 10. Examples: 5400 can be written as 5.4 x 103 0.00072 can be written as 7.2 x 10-4 293,000,000 can be written as 2.93 x 108 0.005186 can be written as 5.186 x 10-3 How do we convert between a decimal and scientific notation, and vice versa?
Converting to Scientific Notation How do we convert between a decimal and scientific notation, and vice versa? If value is larger than 1, start with a decimal between 1 and 10. Move the decimal to the right until it gets to it s original place. The number of times you move the decimal is the power of the 10. If value is smaller than 1, start with a decimal between 1 and 10 based on the value. Move the decimal to the left until it gets to it s original place. The number of times you move the decimal is the negative power of the 10. We can use our previous examples to see: 5400 can be written as 5.4 x 103 0.00072 can be written as 7.2 x 10-4 293,000,000 can be written as 2.93 x 108 0.005186 can be written as 5.186 x 10-3
Examples Using Scientific Notation Examples: 6.02 x 1023is Avogadro s number, one of the fundamental constants of chemistry 9.461 x 1015 meters is one light-year 1 x 10-10 meters is the typical size of an atom Wavelength of UV rays typically span from 1 x 10- 8 to 4 x 10-7 meters, or 10 to 400 nanometers.
Lets Practice Converting! 6.4 x 106 meters (earth s average radius) 1.5 x 109 km (distance from earth to sun) 7 x 109 (population of earth) 418 4180 0.0000418
Comparing Values, Without Evaluating One reason we care about scientific notation is that it allows us to compare incredibly large or incredibly small values more easily. We can also perform arithmetic on scientific notation (using rules/laws of exponents). 6.8 x 1017 vs 7.2 x 1017 1 x 10-8 vs 1 x 10-7 1 x 10-8 vs 4 x 10-7 2.36 x 109 vs 2.35 x 109 3.15 x 10-19 vs 3.15 x 1018 6.02 x 1023 vs 9.461 x 1015 2.36 x 10-9 vs 2.35 x 10-9 (2.0 104) (3.5 10 2) (6.0 106)/(2.0 103 )
Thanks for Coming! 1. Workshop Survey: https://forms.gle/D6MqNV48SnSWPuNQ8 2. More QSC Workshops and Events: