Triangles Inequalities and The Hinge Theorem
5-7
Inequalities in Two
Triangles
The Hinge Theorem (SAS Inequality Thm.)
If two sides of one triangle are congruent to two sides
of another triangle, and the included angles are not
congruent, then the longer third side is opposite the
larger included angle
Problem 1: Using the Hinge Theorem
Problem 2: Applying the Hinge Theorem
Converse of the Hinge Theorem (SSS
Inequality)
If two sides of one triangle are congruent to two sides
of another triangle, and the third sides are not
congruent, then the larger included angle is opposite
the longer third side
Problem 3: Using the Converse of the
Hinge Theorem
Understanding the inequalities in triangles using the Hinge Theorem. The theorem explains how the length of the third side of a triangle relates to the included angles and congruent sides in different triangle configurations. Explore problems that involve applying the Hinge Theorem and its converse in determining relationships between sides and angles in triangles.
Download Presentation
Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author.If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
You are allowed to download the files provided on this website for personal or commercial use, subject to the condition that they are used lawfully. All files are the property of their respective owners.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author.
E N D
Presentation Transcript
5-7 Inequalities in Two Triangles
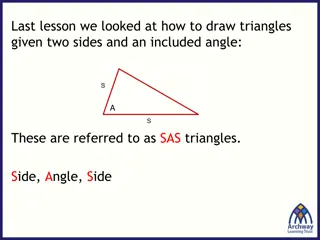
The Hinge Theorem (SAS Inequality Thm.) If two sides of one triangle are congruent to two sides of another triangle, and the included angles are not congruent, then the longer third side is opposite the larger included angle
Converse of the Hinge Theorem (SSS Inequality) If two sides of one triangle are congruent to two sides of another triangle, and the third sides are not congruent, then the larger included angle is opposite the longer third side
Problem 3: Using the Converse of the Hinge Theorem
