Introduction to MATLAB: Overview, Programming, and Applications

MATLAB, short for MATrix LABoratory, is a powerful tool that simplifies matrix computations with integrated visualization and programming features. Developed by Cleve Moler in the 1970s, MATLAB is widely used for mathematical operations, programming structures like conditions and loops, graphical user interfaces, plotting, symbolic math, control systems, file operations, and more. It finds applications in math, computation, modeling, simulation, data analysis, visualization, and scientific/engineering graphs.

• MATLAB programming
• Mathematical computations
• Data analysis
• Control systems
• Scientific visualization

oemm Follow

Uploaded on Oct 07, 2024 | 1 Views

Download Presentation

Please find below an Image/Link to download the presentation.

The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author.If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.

You are allowed to download the files provided on this website for personal or commercial use, subject to the condition that they are used lawfully. All files are the property of their respective owners.

The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author.

Presentation Transcript

1. MATLAB outline MATLAB outline MATLAB overview. Programming: conditions and switch case. Programming: for and while loops. Programming: function, try catch and structure. Graphical user interface (GUI). Plotting. Polynomials, block diagram, symbolic math toolbox and control. File: Read, write and append. SIMULINK.

2. Today's outline Today's outline Introduction Simple math operations Variables who and whos clc and clear help, lookfor and doc Rounding

3. WHY MATLAB

4. Introduction: some applications (MATLAB) Introduction: some applications (MATLAB) Math and computation Programming Modeling and simulation Plotting and data representation Data analysis, exploration and visualization Systems and control Scientific and engineering graphs

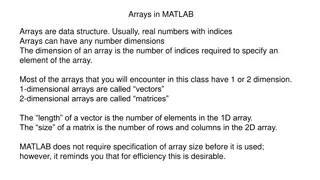
5. Introduction Introduction MATLAB stands for MATrix LABoratory. MATLAB was designed to make matrix computations particularly easy. Cleve Moler wrote the first version of MATLAB in 1970s. MATLAB integrates computation + visualization + programming in a very easy way to use.

6. fix (a) % round a toward zero floor (a) % round a toward -& ceil (a) % round a toward +& round (a) % round a toward to the nearest integer