Capacitors: Examples and Applications
Explore examples of parallel-plate capacitors with varying dimensions and configurations, calculating capacitance, maximum charge, and energy storage. Understand how capacitors behave in circuits and how to determine equivalent capacitance in complex setups.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author.If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
You are allowed to download the files provided on this website for personal or commercial use, subject to the condition that they are used lawfully. All files are the property of their respective owners.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author.
E N D
Presentation Transcript
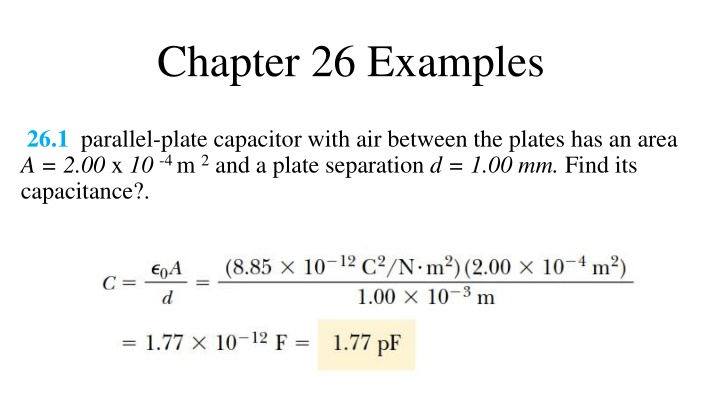
Chapter 26 Examples 26.1 parallel-plate capacitor with air between the plates has an area A = 2.00 x 10 -4 m 2and a plate separation d = 1.00 mm. Find its capacitance?.
4. Find the equivalent capacitance between a and b for the combination of capacitors shown in Figure. All capacitances are in microfarads.
We reduce the combination step by step as indicated in the figure. The 1.0- m F and 3.0- mF capacitors are in parallel and combine according to the expression C eq = C 1 + C 2 = 4.0 mF. The 2.0-mF and 6.0-mF capacitors also are in parallel and have an equivalent capacitance of 8.0 mF. Thus, the upper branch in Figure consists of two 4.0-mF capacitors in series, which combine as follows: The lower branch in Figure consists of two 8.0- F capacitors in series, which combine to yield an equivalent capacitance of 4.0 F. Finally, the 2.0- F and 4.0- F capacitors in Figure are in parallel and thus have an equivalent capacitance of 6.0 F.
6. A parallel-plate capacitor has plates of dimensions 2.0 cm by 3.0 cm separated by a 1.0-mm thickness of paper. (A) Find its capacitance.
(B) What is the maximum charge that can be placed on the capacitor?
7. A parallel-plate capacitor is charged with a battery to a charge Q0, as shown in Figure a. The battery is then removed, and a slab of material that has a dielectric constant 1 is inserted between the plates, as shown in Figure b. Find the energy stored in the capacitor before and after the dielectric is inserted.