Advanced Higher Algebraic Long Division Unit 1

This content covers advanced higher algebraic long division for Unit 1, including outcomes, answers, remainders, factors, and solutions. It also delves into partial fractions with distinct linear factors, providing examples and solutions to various algebraic problems.

• Algebraic Division
• Advanced Higher
• Unit 1
• Partial Fractions
• Factors

tbalt Follow

Uploaded on Oct 10, 2024 | 0 Views

Download Presentation

Please find below an Image/Link to download the presentation.

The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.

Presentation Transcript

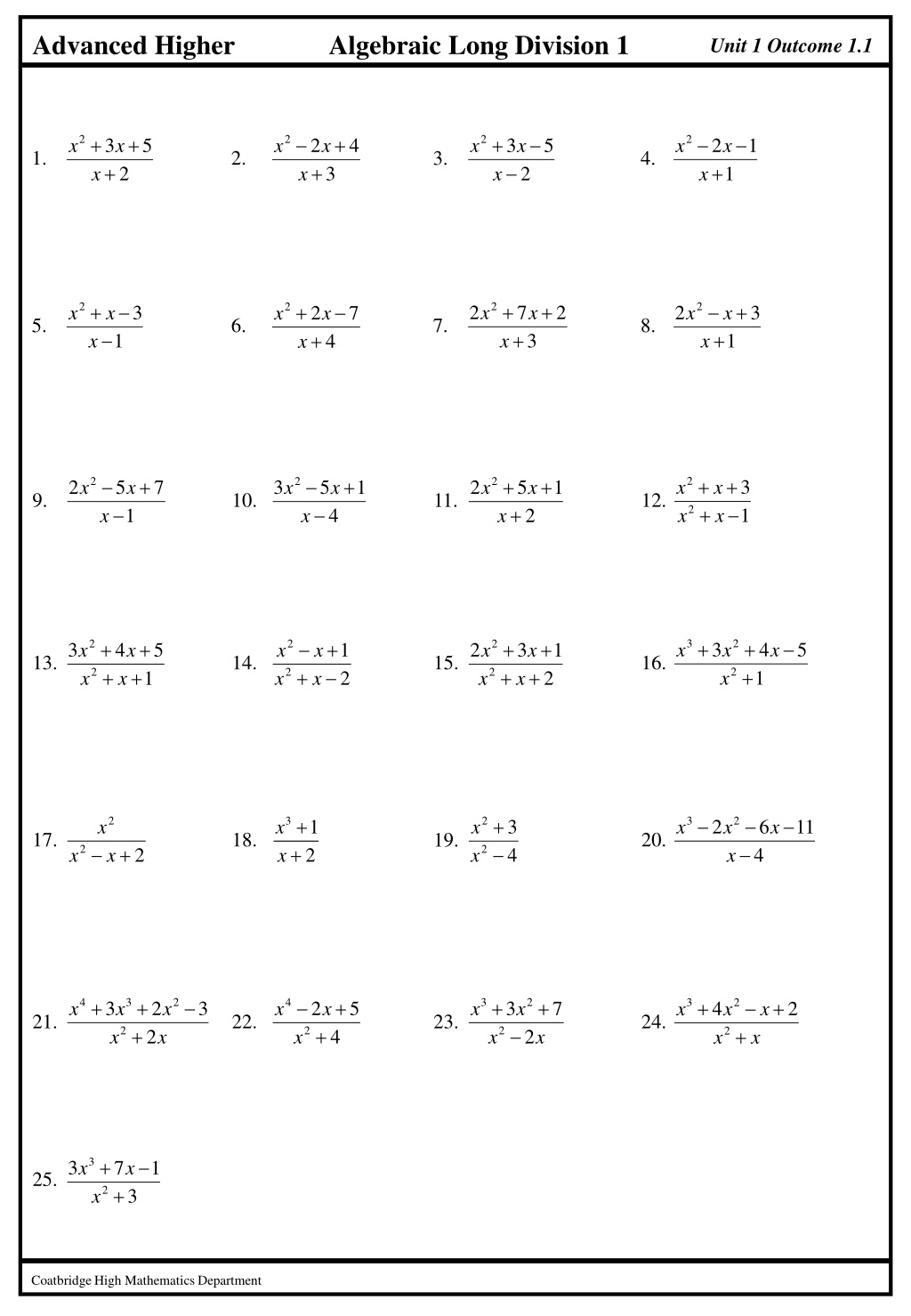
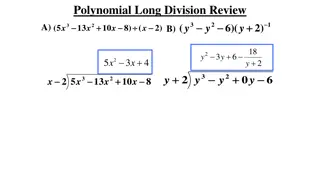
1. Advanced Higher Algebraic Long Division 1 Unit 1 Outcome 1.1 + x x + x + x + 2 2 2 2 3 5 2 + 1 3 + 5 2 + 4 x x x x x x x x 1. 2. 3. 4. 2 1 2 3 + x + + x + + x 2 2 2 2 2 7 + 2 3 2 3 x x 2 + 7 x x x x x x 5. 6. 7. 8. + 3 1 1 4 x + + x + x + + x + 2 2 2 2 3 2 5 7 3 5 1 2 5 1 x x x x x x x x 9. 10. 11. 12. + + 2 1 1 4 2 x x + + + + + + + 3 2 2 2 2 3 4 5 3 4 x 5 1 x x x 2 3 x 1 x x x x x x 13. 14. 15. 16. + + + + + + 2 2 2 2 1 1 2 x 2 x x x x + + 2 3 2 2 3 2 6 11 1 2 3 x x x x x x x 17. 18. 20. 19. x + + 2 2 2 4 4 x x x + + x + + + + + 4 3 2 4 3 2 3 2 3 2 3 2 + 5 3 7 x x 2 x 4 2 x x x x x x 2 x 21. 22. 23. 24. + + 2 2 2 4 2 x x x x x x + 3 3 7 + 1 x x 25. 2 3 x Coatbridge High Mathematics Department

2. Advanced Higher Algebraic Long Division 1 Unit 1 Outcome 1.1 ANSWERS 3 + 19 + x 5 2 + + + + + + + 1 5 5 3 x x x x 1. 2. 3. 4. 2 3 2 1 x x x 1 6 + 1 + 1 + + + 2 2 3 x x + + 5. 6. 7. 8. 2 2 1 x x 1 1 x x 4 3 x x 4 29 x 1 + 4 + + + + + 2 3 3 7 2 1 1 x x x 9. 10. 11. 12. + 2 1 4 2 1 x x x x + + x 2 2 + 3 3 + 3 x 8 x x x x + + + + + 3 1 2 3 x 13. 14. 15. 16. + + + 2+ 2 2 2 1 2 2 1 x x x x x 2 + 7 + 7 3 x + + +x + + 2 2 1 2 4 1 2 2 x x x x 17. 18. 19. 20. 2 2 2 2 4 4 x x x x + + + 3 + 2 x 21 10 7 4 2 x x x + + + + + + + 2 2 4 5 3 x x x x x 21. 22. 23. 24. + 2 2 2 2 2 4 2 x x x x x x 2 2+ x 1 x + 3 x 25. 3 Coatbridge High Mathematics Department

3. Advanced Higher Algebraic Long Division 2 Unit 1 Outcome 1.1 1. Find the remainder on dividing the following: + 3 2 2 3 4 x x x a) by 3 x + + 3 2 7 5 x x x + 2 b) by x 2 + x 3 c) by + 3 2 2 3 8 5 x x x 2 + x 1 d) 2 by + 3 2 3 3 2 x x x e) + by 3 x 3 2 6 11 6 x x x 2. Find the factors for the following: a) + 3 2 2 5 6 x x x x b) 3 7 6 x + 3 2 c) 2 3 3 2 x x x + + + 3 2 d) 2 9 13 6 x x x e) 3 2 6 13 14 3 x x x + + 4 3 2 11 9 18 x x x x f) g) + + 4 2 4 31 21 18 x x x 3. Solve the following equations a) + = 3 2 2 7 3 18 0 x x x + = b) 3 2 4 4 5 3 0 x x x + = 3 2 c) 6 17 5 6 0 x x x + = 4 2 d) 15 10 24 0 x x x e) + + = 4 3 2 6 23 23 2 8 0 x x x x Coatbridge High Mathematics Department

4. Advanced Higher Algebraic Long Division 2 Unit 1 Outcome 1.1 ANSWERS 1. Remainders a) 4 b) 15 c) 3.5 d) 0 e) 0 2. Factors + + ) 2 ( 1 )( 3 )( x x x a) + + ) 3 ( 1 )( 2 )( x x x b) + ) 1 ( 1 )( 2 )( 2 x x x c) + + ) 3 + ( 1 )( 2 )( 2 x x x d) + ) 1 + e) ( 3 )( 3 1 )( 2 x x x + ) 3 + ( 1 )( 2 )( 3 )( x x x x f) + ) 1 + ( 3 )( 2 )( 2 3 )( 2 x x x x g) 3. Solutions 3 = , 2 3 , 2 , 2 1 x a) 1 3 = , 1 x b) 2 2 = , 3 c) , x 2 4 , 3 3 = , 2 d) , 1 x 4 1 = , 1 , 2 e) , x 3 2 Coatbridge High Mathematics Department

5. Unit 1 Outcome 1.1 Advanced Higher Partial Fractions 1 Type 1 Distinct Linear Factors 2 x 10 4 20 x x 1. 2. 3. 4. ) 1 + ( 1 )( x x + + + + ( 1 )( ) 5 ( 2 )( ) 3 ( 3 )( ) 2 x x x x x x 3 2 x 3 5 14 x x 5 5. 8. 6. 7. + + ( 2 )( ) 2 x x ) 3 ( 1 )( ) 2 x x ( 2 )( x + + ( 2 )( ) 3 x x 6 x 15 + 15 x 2 x x x + 7 5 + x 7 x x 12. 9. 10. 11. + ( ) 3 2 ( 1 )( ) 3 x ) 1 + ( 1 )( ) 3 ( 3 )( x x x x 7 11 x 4 6 + x x x + 3 12 3 4 x x 16. 15. 14. 13. ) 1 + 5 ( )( x ( ) 4 x + + ( ) 6 x ( ) 2 x x + + x 2 7 11 + 6 x x x 5 + 3 5 2 11 x x x 17. 18. 20. 19. ) 1 + 2 ( 3 )( 4 3 ( 1 )( 2 ) 3 + x x x 2 30 4 3 x x + + x 4 10 6 1 4 2 1 x 4 + 5 + x 2 x x x 21. 22. 24. 23. 2 + + 2 3 4 1 2 3 1 x x x x 3 2 x x 2 2 2 6 x x x 25. + + ) 1 ( 1 )( 2 )( x Coatbridge High Mathematics Department

6. Unit 1 Outcome 1.1 Advanced Higher Partial Fractions 1 ANSWERS 4 4 + 1 1 + 4 6 + 1 5 + + + + 4. 1. 2. 3. + 3 2 x x 1 1 2 3 1 5 x x x x x x 2 + 1 1 1 + 5 + 5 + 4 1 + + 8. 5. 6. 7. 2 2 x x 1 2 2 3 2 3 x x x x x x x 5 3 11 + 5 4 3 + 3 4 + + + + 12. 9. 10. 11. ) 3 ) 1 + ( 2 ( 2 x 3 x x 2 1 3 1 3 x x x x 4 3 + 2 x 1 + 2 x 1 + 1 x 7 + +x x 16. 14. 15. 13. 5 1 x x 2 6 4 x 3 2 1 3 2 3 2 3 + + + + 20. 17. 18. 19. + 1 3 x x 3 1 2 3 2 3 4 1 5 6 x x x x x x 10 2 3 + 2 1 + 3 + 1 2 + + + 24. 21. 22. 23. ) 3 + 3 ( 3 + x x 1 2 1 x x 1 2 2 1 2 1 x x x x 1 + 2 + 1 + 25. 1 2 1 x x x Coatbridge High Mathematics Department

7. Unit 1 Outcome 1.1 Advanced Higher Partial Fractions 2 Type 2 Repeated Linear Factor + + x 2 2 2 9 2 3 x 11 5 4 9 + x x x x x 1. 2. 3. + ) 1 ) 1 2 2 2 ( 1 )( ( 2 )( ( 2 )( ) 3 x x x x x + x 2 7 + x 2 2 6 3 6 7 x x x x 4. 5. 6. + 2) 2 ( 1 )( x ) 1 + 2 2 ( ( 3 )( ) 2 x x x + + x 16 5 1 2 x 5 x x 7. 8. 9. + 2) 3 2) 1 + 2) 2 + ( 1 )( 2 ( x 1 ( )( x x x + + x + 2 2 2 4 x 8 15 2 7 3 x x x x 10. 11. 12. + 2) 1 + ) 2 ( 1 )( ) 1 + x x 2 2 ( 1 )( ( x x + 2 2 3 1 7 2 1 x x x 13. 14. ) 1 + ) 1 2 ( 2 )( ( x x x x Coatbridge High Mathematics Department

8. Advanced Higher Partial Fractions 2 Unit 1 Outcome 1.1 ANSWERS 2 + 1 5 5 8 3 1 3 + 9 + + + 1. 2. 3. 2) 1 2) 1 2) 3 + 1 1 ( 2 1 ( 2 3 ( x x x x x x x x x 1 + 1 1 2 4 + 3 3 x 4 4 + + + 4. 5. 6. 2) 2 2) 2 + 2) 1 1 2 ( 3 2 ( 1 ( x x x x x x x x 1 + 1 4 1 x 2 3 7 7 + 1 + + + + 7. 8. 9. 2) 3 2) 2 + + 2) 1 + 1 3 ( 1 ( 9 ) ( 9 ) 2 ( 3 2 1 2 ( x x x x x x x x 3 + 1 5 3 x 1 + 2 1 1 1 + + + + 10. 11. 12. 2) 2 2) 1 + ) 1 + ) 1 2) 1 1 2 ( 1 ( ( 2 ( 2 ( x x x x x x x x 1 2 + 1 8 1 x 1 x + 13. 14. 2) 1 + 2 1 ( x x x 2 1 x Coatbridge High Mathematics Department

9. Unit 1 Outcome 1.1 Advanced Higher Partial Fractions 3 Type 3 Irreducible Quadratic Factor 1.a) Show that the quadratic is irreducible. + x + 2 2 2 x + + x 2 3 2 1 x x b) Hence express in partial fractions. )( 1 ( + + x x + 2 2 ) 2 + x + 2 2 5 2.a) Show that the quadratic is irreducible. x 8 2 b) Hence express in partial fractions. )( 1 ( x x + ) 5 + 2 x 3. Express in partial fractions (you must show first that the quadratic factor is irreducible). x + x 3 2 x 2 2 4 3 2 2 10 2 x 8 x x x x x a) b) c) 2+ ( ) 2 + ) 1 + + + x x ( 1 )( ( 2 )( 2 ) 4 x x + + 2 4 + 5 2 13 x x x + x + x + + 2 2 2 3 2 1 2 9 x x x x d) e) f) + ) 3 + ( 1 )( x x + ) 1 + ) 3 + 2 ( 1 )( ( 1 )( x x x The following question is an actual examination question. 4.a) Factorise the cubic polynomial as the product of a linear factor l(x) and a quadratic factor q(x). 3 2 2 x x x b) Show that the quadratic factor q(x) is irreducible. + 5 4 x x c) Hence express in partial fractions. 2 3 x x 2 Coatbridge High Mathematics Department

10. Advanced Higher Partial Fractions 3 Unit 1 Outcome 1.1 ANSWERS 1.a) Proof 2 + 3 x x b) + + + 2 1 2 2 x x 2.a) Proof + 1 3 x x + b) + + 2 1 2 5 x x x 1 3 1 + x + 2 3 1 x 3 x x + 3.a) 2 b) c) + + + 1 1 x x + + 2+ 2 2 2 4 2 x x x x x 4 + 1 2 1 3 2 x + + + d) 2 e) f) + + + + 2+ 2 1 3 1 1 1 3 x x x x x x x = + ) 1 + 3 2 2 2 ( 2 )( x x x x x x 4.a) b) Proof x 2 2 + 1 x + c) + 2 2 1 x x Coatbridge High Mathematics Department

11. Unit 1 Outcome 1.1 Advanced Higher Partial Fractions 4 Mixed Partial Fractions 1. Express in partial fractions (remember to ensure that the denominator of each fraction is factorised fully. 8 x 3 3 4 4 9 x x x a) b) c) d) ) 1 ) 1 + + 2) 1 ) 3 ( ( 2 )( ( 1 )( ( 2 )( x x x x x x x + + + + 5 1 + x 2 3 4 10 x 3 1 x 2+ x x e) f) g) h) ) 1 + 2 ( 1 )( ) 1 + x x x 2 2 ( 4 12 x x x x x + x + + 8 2 1 x 2 2 10 16 x x x x i) j) k) l) + ) 5 + ) 1 2 1 ( )( 2 ( + ) 1 + x x x x 2 2 ( 2 )( 2 6 x x x 2 + 2 + 6 1 2 9 2 x x x 2 7 3 x 3 x 2 m) n) o) 2 ) 1 2 + + 4 1 ( 1 )( x x x x x x 9 5 x 2. Express in partial fractions. )( 2 )( 1 ( x x ) 3 x 2+ x 3.a) Prove that does not factorise into the product of two linear factors with real coefficients. 2 x 2 3 2+ x x b) Express in partial fractions. ) 2 ( + + + 3 2 2 4 3 x x x 4.a) Factorise into the product of a linear factor and a quadratic factor, and prove that the quadratic factor is irreducible. + + 2 4 2 7 + x x b) Express in partial fractions. 4 2 + + x x 3 2 3 x + 3 2 3 3 2 x x x 5.a) Factorise into the product of a linear factor and a quadratic factor and prove that the quadratic factor is irreducible. + x x x 2 4 4 1 x x b) Express in partial fractions. 3 3 + 3 2 2 Coatbridge High Mathematics Department

12. Advanced Higher Partial Fractions 4 Unit 1 Outcome 1.1 ANSWERS 1 + 1 2 3 x 5 2 1 + 1 3 + +x + + 1. a) b) c) d) 2) 1 1 1 ( x x x 1 2 1 2 3 x x x x 1 x 2 + 4 x + 2 2 + 1 1 x 2 + 2 1 + x + + +x e) f) g) h) 2) 1 + 1 ( x x + 2 1 1 4 4 3 x x x x + 2 + 1 3 1 3 x 2 2 x 1 x 5 2 + x + + i) j) k) l) 2) 1 2 1 ( + + x x x 2 2 1 2 5 1 2 3 2 x x x x x 3 x 1 + 2 2 + 1 5 1 2 + + m) n) o) 2) 1 + 2) 1 1 ( + 1 1 ( x x x x x 2 1 2 1 x x 2 1 3 2. + 1 2 3 x x x 2+ 2+ 3.a) The discriminant of is , which is less than zero, hence does not factorise. 2 x 8 2 x + 1 x 3 x + b) 2+ 2 x + + + = + + ) 3 + 3 2 2 2 4 3 ( 1 )( x x x x x x 4.a) The discriminant of is , which is less than zero, hence does not factorise. 3 + +x x 11 +x + 2 2 3 x 3 + 2 + x + b) + 2 1 3 x x x + = ) 1 + 3 2 2 3 3 2 ( 2 )( x x x x x x 5.a) The discriminant of is , which is less than zero, hence does not factorise. 1 + x x 3 x + 2 2 1 x + 3 1 x + b) + 2 2 1 x x x Coatbridge High Mathematics Department

13. Unit 1 Outcome 1.1 Advanced Higher Partial Fractions 5 Partial Fractions for Improper Rational Functions Using algebraic long division first, express each of the improper rational functions below as the sum of a polynomial function and partial fractions. + x + + 2 3 2 3 2 6 5 x 1 5 x 11 x 12 x x x x x x x x a) b) c) + + 2 2 2 2 2 3 5 6 x x + + + 3 2 2 2 4 2 3 x x 2 x x x d) e) f) + 2 2 1 4 x x x x + ) 1 ( 3 )( x x + x x 3 2 2 2 5 + 6 x x x g) h) + ) 1 + ( 2 )( x x 4 3 Coatbridge High Mathematics Department

14. Advanced Higher Partial Fractions 5 Unit 1 Outcome 1.1 ANSWERS 4 + 2 1 1 + 3 2 + + + + + 1 1 x x a) b) c) 2 1 3 1 3 2 x x x x x x 1 1 + 2 x 6 + 2 2 + + + + + 1 3 1 x d) e) f) 2 2 1 1 1 x x x x x 3 + 4 + 12 x 4 + + + + 1 6 x g) h) 2 1 3 1 x x x Coatbridge High Mathematics Department

15. Unit 1 Outcome 1.1 Advanced Higher Partial Fractions 6 Mixed Examples + + 2 + 5 x 3 13 x x x x 1. 2. 3. + + ) 1 5 ( 1 )( 7 ) 2 2 ( 1 )( ) 3 x x ( 2 )( x x 8 x + 2 x 3 2 x 2 + 3 x x 4. 5. 6. ) 1 + 2 + ( ) 2 ( x x ( 1 )( 2 )( ) 3 x x 3 2 3 x x + x 7 5 2+ x 1 2 3 x x 7. 8. 9. ( 3 )( ) 4 4 x + ) 1 + 1 x 2 ( 2 )( 2 x + + 2 + + 9 3 5 3 2 x x 2 2 5 2 1 15 + x x 3 x x 10. 11. 12. ) 1 + ) 2 ) 5 + ( ( x x 2 x x ( 1 )( 2 x x x + 4 4 5 x x 3 2 17 + x 13. 14. ) 3 + 2 2 + + ( 1 )( x x 2 ( 1 )( 2 ) 2 x x x Coatbridge High Mathematics Department

16. Advanced Higher Partial Fractions 6 Unit 1 Outcome 1.1 ANSWERS 2 1 4 5 + x 1 8 x + 1. 2. 3. ) 1 ( ) 2 ( x x 5 1 7 2 x + ) 1 + ( 5 ) 3 2 ( 5 x 1 1 9 x 1 + 1 2 3 x 11 x 34 x + + 4. 5. 6. + ) 1 + 2) 2 ( 4 ( 5 ) 2 20 ( ) 3 1 2 ( x x x x x + 2 3 1 1 + ( 7 ) 2 1 1 1 2+ 2 2 x 1 x x + 7. 8. 9. 2+ ) 1 ) 1 + 2+ ( 9 ) 2 2 ( 9 ) 1 ( 4 ( 4 ( 2 ) 1 x x x x x 3 4 x + 1 1 + 2 1 2 5 x 1 x 2 2 + x 10. 11. + + + + 12. 2 ) 1 + ) 1 + 2 3 + + 2 2 1 ( ( x x x x 1 2 5 1 1 x x x x + + x + x 1 3 8 + 3 20 + 19 + x 13 2 x 17 + x x x x + + 2 13. 14. ) 1 2 2 2 + ( 3 ( ) 3 x x x 1 2 2 Coatbridge High Mathematics Department