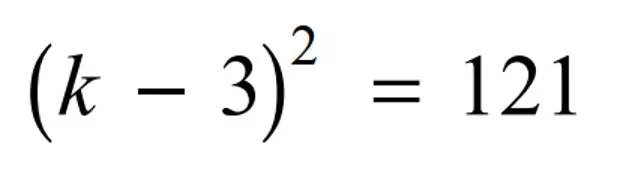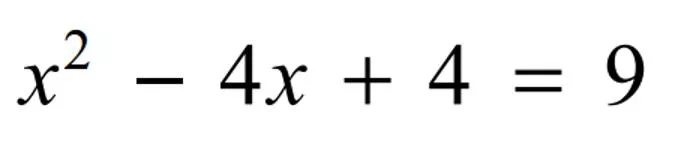Algebraic Equations and Quadratic Functions Exploration
Explore the world of algebraic equations and quadratic functions through visual aids and interactive activities. Learn to solve equations using square roots, complete the square, write functions in vertex form, and work with algebra tiles to visualize mathematical concepts. Discover the relationship between coefficients in perfect squares and solve problems to enhance your understanding of algebraic expressions.
Uploaded on Sep 12, 2024 | 0 Views
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
Bellwork Solve the equation Using square root: 1. 2.
Solving quadratic function by Solving quadratic function by completing a square completing a square Section 3.3
What You Will Learn Solve quadratic equations using square roots. Solve quadratic equations by completing the square. Write quadratic functions in vertex form.
Lets work with algebra tiles ?2tile x tile 1 tile
Use algebra tiles to multiply ( Use algebra tiles to multiply (x x + 2)( + 2)(x x + 3) + 3) You Try: multiply (x + 2)(x + 5)
Use algebra tiles to complete the square for the expression ?2+ 6x.
Use algebra tiles to complete the square for the expression ?2+ 4x+ ? What is the length of each side of the square? What is the area of the bigger square?
??? ????? ?2+ 6x + c What is the length of each side of the square? What is the area of the bigger square?
Work with your partner and fill up the table Work with your partner and fill up the table using you Algebra tiles. using you Algebra tiles.
In a perfect square, there is a relationship between the In a perfect square, there is a relationship between the coefficient of the middle term and the constant term. coefficient of the middle term and the constant term. 2 ( 7) x+ = 7 =1(14) 2 7 + + 2 14 49 x x 7 = 49 2
Can you come up with a pattern to find the Can you come up with a pattern to find the value of C? value of C? Solve problem # 5 -7 in practice number 3.3 A side
Solving Quadratic Equations by Completing the Square Solve the following equation by completing the square: + = 2 x x 8 20 0 Step 1: Keep quadratic term, and linear term to left side of the equation and move the constant term to the right side of the equation + = 2 x x 8 20
Solving Quadratic Equations by Completing the Square =20 + Step 2: Find the term that completes the square on the left side of the equation. Add that term to both sides. 2 x + + 2 x x 8 1 2 ( ) 8 = = 2 4 then square it, 4 16 16 16 + + = + x 8 20
Solving Quadratic Equations by Completing the Square Step 3: Factor the perfect square trinomial on the left side of the equation. Simplify the right side of the equation. 16 16 + + = + 2 x x 8 20 = = x x ( ( 4)( 4) 36 36 2 x 4)
Solving Quadratic Equations by Completing the Square Step 4: Take the square root of each side x + = 2 ( 4) 36 x + = ( 4) 6
Solving Quadratic Equations by Completing the Square Step 5: Set up the two possibilities and solve = x x x = 4 6 d x = = + 4 6 an 10 and 4 6 x= 2
You Try Practice packet 3.3 A side Problem # 9 - 12