Algebraic Fractions Simplification Techniques
Starter
Factorise the following:
8x² - 32x
x² + 5x – 14
3x² + 17x + 10
= 8x(x – 4)
= (x + 7)(x – 2)
= (3x + 2)(x + 5)
Simplifying Algebraic Fractions
To simplify algebraic fractions:
1)
factorise the top and/or the bottom
2)
cancel common factors
=
3(x + 2)
3x²
3x + 6
3x²
=
x + 2
x²
=
4a(b + 2a)
12a
4ab + 8a²
12a
=
b + 2a
3
3
Why can’t we
cancel this
further?
7x – 35
21
3x² + 15x
27x
x² + 7x + 10
3x + 15
6x²y + 18xy
21xy
Checkpoint
x – 5
3
x + 5
9
2(x + 3)
7
x + 2
3
Simplifying Algebraic Fractions
To simplify algebraic fractions:
1)
factorise the top and/or the bottom
2)
cancel common factors
=
4(x - 5)
(x – 5)(x – 8)
4x - 20
x² - 13x + 40
= _
4_
(x – 8)
=
(x + 4)(x – 4)
(x + 4)(x – 5)
x² - 16
x² - x - 20
=
(x – 4)
(x - 5)
Simplifying Algebraic Fractions
5x – 25
12x + 30
20x² - 40x
10x²
9x²
15x²
8ab – 16b
9cd² - 24d²
14efg² + 7ef²
24ab
15d
21efg
x² + 9x + 20
x² - 3x - 88
x² - 49
7x + 28
x² - 21x + 110
x² - 5x - 14
2x² + 7x + 3
5x² - 3x - 14
6x² - 13x - 5
x² - 9
x² - 11x + 18
10² - 19x - 15
Answers
x – 5
2x + 5
4x - 8
2x²
3x²
3x
a - 2
3cd – 8d
2g² + 7f
3a
5
3g
x + 5
x + 8
x + 7
7
x - 10
x + 2
2x + 1
5x + 7
3x + 1
x - 3
x - 9
5x + 3
Multiplying Algebraic Fractions
To multiply algebraic fractions:
1)
cancel common factors
2)
multiply the numerators, multiply the denominators
= a x
3b
4
4a
x
15b
5 16
=
3ab
4
n + 4
x
3n – 9
n – 3 5n + 20
4
3
=
n + 4
x
3(n – 3)
n – 3 5(n + 4)
=
3
5
Dividing Algebraic Fractions
To divide algebraic fractions:
1)
flip the second fraction and change ÷ to x
2)
cancel common factors
3)
multiply the numerators, multiply the denominators
_
11_
÷
77y
9x²y 15x
x² - 4
÷
x² - 5x + 6
x² + 4x – 32 5x + 40
= _
11_
x
15x
9x²y 77y
7
3x
5
= _
1_
x _
5_
3xy 7y
= _
5_
21xy²
=
x² - 4
x
5x + 40
x² + 4x – 32 x² - 5x + 6
=
(x + 2)(x – 2)
x
5(x + 8)
(x + 8)(x – 4) (x – 2)(x – 3)
=
5(x + 2)
(x - 4)(x – 3)
Adding and Subtracting Fractions
2
+
1
3
4
1) Find a common denominator:
3
x
4
= 12
2
=
8
3
12
1
=
3
4
12
2) Find equivalent fractions with that denominator:
3) Add (or subtract!) the numerators and put the answer over the
same denominator:
8
+
3
=
11
12 12 12
4) Simplify if possible.
Adding and Subtracting Algebraic Fractions
To simplify algebraic fractions:
1)
Find a common denominator
2)
Find equivalent fractions with that denominator
3)
Add (or subtract!) the numerators and put the answer over
the same denominator
4)
Simplify if possible.
2x
+
x
3 4
=
8x
+
3x
12 12
=
11x
12
4
-
5
x 2x
=
8
-
5
2x 2x
=
3
2x
Adding and Subtracting Algebraic Fractions
To simplify algebraic fractions:
1)
Find a common denominator
2)
Find equivalent fractions with that denominator
3)
Add (or subtract!) the numerators and put the answer over
the same denominator
4)
Simplify if possible.
x + 1
+
x – 3
4 7
=
7(x + 1)
+
4(x – 3)
28 28
=
7x + 7 + 4x + 12
28
=
11x - 5
28
Adding and Subtracting Algebraic Fractions
To simplify algebraic fractions:
1)
Find a common denominator
2)
Find equivalent fractions with that denominator
3)
Add (or subtract!) the numerators and put the answer over
the same denominator
4)
Simplify if possible.
_
9
_ - _
8
_
x – 2 x + 4
=
9(x + 4)
-
8(x – 2)
(x – 2)(x + 4) (x + 4)(x – 2)
=
9x + 36 – 8x + 16
x² + 2x - 8
=
x + 52
x² + 2x - 8
Explore how to factorize and simplify algebraic fractions using common techniques such as factorization, canceling common factors, and multiplying/dividing fractions. The process involves identifying factors, canceling where possible, and performing operations to simplify expressions. Checkpoints and answers are provided to reinforce understanding and practice. Mastering these methods will aid in solving complex algebraic problems efficiently and accurately.
Download Presentation
Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author.If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
You are allowed to download the files provided on this website for personal or commercial use, subject to the condition that they are used lawfully. All files are the property of their respective owners.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author.
E N D
Presentation Transcript
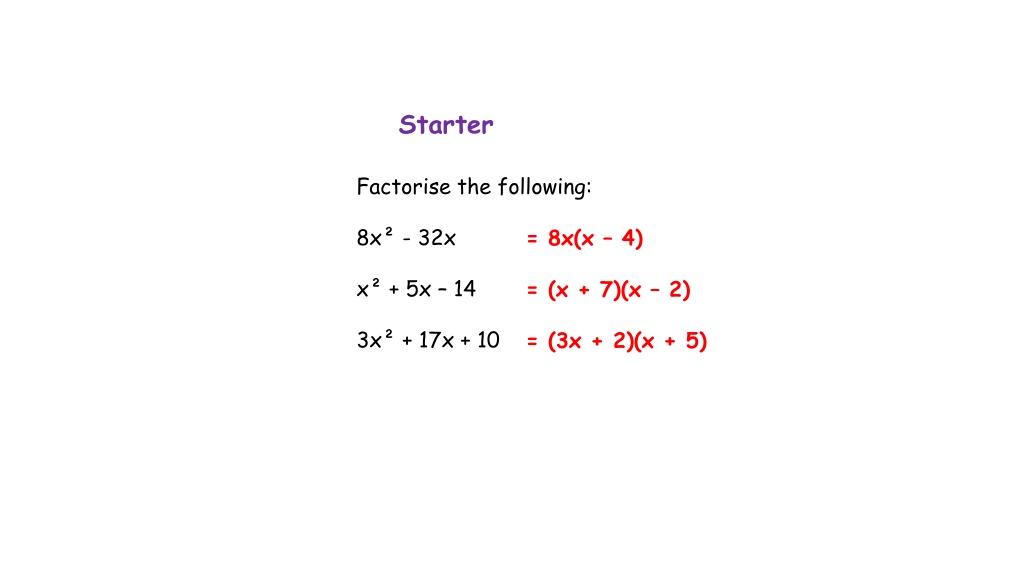
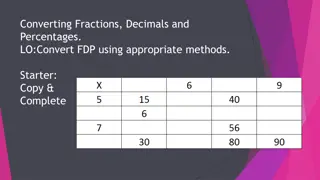
Starter Factorise the following: 8x - 32x = 8x(x 4) x + 5x 14 = (x + 7)(x 2) 3x + 17x + 10 = (3x + 2)(x + 5)
Simplifying Algebraic Fractions To simplify algebraic fractions: 1) factorise the top and/or the bottom 2) cancel common factors Why can t we cancel this further? 3x + 6 3x = 3(x + 2) 3x = x + 2 x = b + 2a 3 4ab + 8a 12a = 4a(b + 2a) 12a 3
Checkpoint 7x 35 21 x 5 3 3x + 15x 27x x + 5 9 6x y + 18xy 21xy 2(x + 3) 7 x + 7x + 10 3x + 15 x + 2 3
Simplifying Algebraic Fractions To simplify algebraic fractions: 1) factorise the top and/or the bottom 2) cancel common factors 4x - 20 x - 13x + 40 = 4(x - 5) (x 5)(x 8) = _4_ (x 8) = (x + 4)(x 4) (x + 4)(x 5) = (x 4) (x - 5) x - 16 x - x - 20
Simplifying Algebraic Fractions 5x 25 10x 12x + 30 9x 20x - 40x 15x 8ab 16b 24ab 9cd - 24d 15d 14efg + 7ef 21efg x + 9x + 20 7x + 28 x - 3x - 88 x - 21x + 110 x - 49 x - 5x - 14 2x + 7x + 3 x - 9 5x - 3x - 14 x - 11x + 18 6x - 13x - 5 10 - 19x - 15
Answers x 5 2x 2x + 5 3x 4x - 8 3x a - 2 3a 3cd 8d 5 2g + 7f 3g x + 5 7 x + 8 x - 10 x + 7 x + 2 2x + 1 x - 3 5x + 7 x - 9 3x + 1 5x + 3
Multiplying Algebraic Fractions To multiply algebraic fractions: 1) cancel common factors 2) multiply the numerators, multiply the denominators 3 4a x 15b 5 16 = a x 3b 4 = 3ab 4 4 = n + 4 x 3(n 3) n 3 5(n + 4) = 3 5 n + 4 x 3n 9 n 3 5n + 20
Dividing Algebraic Fractions To divide algebraic fractions: 1) flip the second fraction and change to x 2) cancel common factors 3) multiply the numerators, multiply the denominators 5= _1_ x _5_ 3xy 7y _11_ 77y 9x y 15x = _11_ x 15x 9x y 77y 3x = _5_ 21xy 7 x - 4 x - 5x + 6 x + 4x 32 5x + 40 = x - 4 x 5x + 40 x + 4x 32 x - 5x + 6 = 5(x + 2) (x - 4)(x 3) = (x + 2)(x 2) x 5(x + 8) (x + 8)(x 4) (x 2)(x 3)
Adding and Subtracting Fractions 2 + 1 3 4 1) Find a common denominator: 3 x 4 = 12 2) Find equivalent fractions with that denominator: 2 = 8 3 12 1 = 3 4 12 3) Add (or subtract!) the numerators and put the answer over the same denominator: 8 + 3 = 11 12 12 12 4) Simplify if possible.
Adding and Subtracting Algebraic Fractions To simplify algebraic fractions: 1) Find a common denominator 2) Find equivalent fractions with that denominator 3) Add (or subtract!) the numerators and put the answer over the same denominator 4) Simplify if possible. 2x + x 3 4 = 8x + 3x 12 12 = 11x 12 4 - 5 x 2x = 8 - 5 2x 2x = 3 2x
Adding and Subtracting Algebraic Fractions To simplify algebraic fractions: 1) Find a common denominator 2) Find equivalent fractions with that denominator 3) Add (or subtract!) the numerators and put the answer over the same denominator 4) Simplify if possible. x + 1 + x 3 4 7 = 7(x + 1) + 4(x 3) 28 28 = 7x + 7 + 4x + 12 28 = 11x - 5 28
Adding and Subtracting Algebraic Fractions To simplify algebraic fractions: 1) Find a common denominator 2) Find equivalent fractions with that denominator 3) Add (or subtract!) the numerators and put the answer over the same denominator 4) Simplify if possible. _9_ - _8_ x 2 x + 4 = 9(x + 4) - (x 2)(x + 4) (x + 4)(x 2) 8(x 2) = 9x + 36 8x + 16 x + 2x - 8 = x + 52 x + 2x - 8
Algebraic Fractions Adding and Subtracting 1) x x 7 + 5 2) 3 3 8x + 4x 3) 2 2 5x + 1 - 3x 4) 3 4 7x + 3x 5) 3 5 7x - 8x 6) 5x x 12 + 3 7) 6 4 x + 3 + x - 2 8) 3 7 2x 5 - 4x + 1 9) x + 4 x - 2 _7_ - _9_ 10) x 1 x + 1 _3_ + _2_
Algebraic Fractions Multiply and Divide 1) 8v 5v 7 x 2 2) 5 4t 6t 3 3) 7j 7 6j _6_ x 5j 5 4) 8x 4 6x + 3 10x + 5 5) 8w 24w - 16w 21w 14 x ___4____ 6) 10b + 20 2b + 4b __3b__ __9__ 7) a + 6a + 5 a + a 12 a - a 6 x a + 3a 10 8) c - 5c + 4 c + c 2 c + 12c + 32 c - 3c 28 9) 3f + 23f 8 2f - f 3 2f - 11f + 12 x 5f + 42f + 16 10) 6g + 7g + 2 5g + 21g + 4 10g - 13g - 3 2g 17g + 8
