Stochastic Storm Transposition in HEC-HMS: Modern Techniques and Applications
Explore the innovative methods and practical applications of Stochastic Storm Transposition (SST) in the context of HEC-HMS. Delve into the history, fundamentals, simulation procedures, and benefits of using SST for watershed-averaged precipitation frequency analysis. Learn about the non-parametric
3 views • 41 slides
The Importance of Completing a Trainer Matrix
Completing a Trainer Matrix is essential for Registered Training Organizations (RTOs) to demonstrate compliance with Standards for RTOs 2015, specifically Clauses 1.13 to 1.16. This matrix outlines requirements for trainers, including holding relevant qualifications, industry skills, and maintaining
2 views • 38 slides
Panel Stochastic Frontier Models with Endogeneity in Stata
Introducing xtsfkk, a new Stata command for fitting panel stochastic frontier models with endogeneity, offering better control for endogenous variables in the frontier and/or the inefficiency term in longitudinal settings compared to standard estimators. Learn about the significance of stochastic fr
0 views • 13 slides
Generalization of Empirical Risk Minimization in Stochastic Convex Optimization by Vitaly Feldman
This study delves into the generalization of Empirical Risk Minimization (ERM) in stochastic convex optimization, focusing on minimizing true objective functions while considering generalization errors. It explores the application of ERM in machine learning and statistics, particularly in supervised
2 views • 11 slides
Singular Value Decomposition and the Conjugate Gradient Method
Singular Value Decomposition (SVD) is a powerful method that decomposes a matrix into orthogonal matrices and diagonal matrices. It helps in understanding the range, rank, nullity, and goal of matrix transformations. The method involves decomposing a matrix into basis vectors that span its range, id
1 views • 21 slides
Diagonalization in Linear Algebra
Discover the concept of diagonalization in linear algebra through eigenvectors, eigenvalues, and diagonal matrices. Learn the conditions for a matrix to be diagonalizable, the importance of eigenvectors in forming an invertible matrix, and the step-by-step process to diagonalize a matrix by finding
1 views • 26 slides
Stochastic Coastal Regional Uncertainty Modelling II (SCRUM2) Overview
SCRUM2 project aims to enhance CMEMS through regional/coastal ocean-biogeochemical uncertainty modelling, ensemble consistency verification, probabilistic forecasting, and data assimilation. The research team plans to contribute significant advancements in ensemble techniques and reliability assessm
0 views • 28 slides
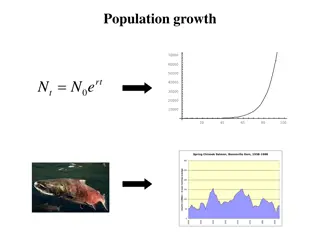
Population Growth Models and Stochastic Effects
Explore the simplest model of population growth and the assumptions it relies on. Delve into the challenges of real-world scenarios, such as stochastic effects caused by demographic and environmental variations in birth and death rates. Learn how these factors impact predictions and models.
0 views • 35 slides
Matrix Factorization for Latent Factor Recovery
Explore the concept of matrix factorization for recovering latent factors in a matrix, specifically focusing on user ratings of movies. This technique involves decomposing a matrix into multiple matrices to extract hidden patterns and relationships. The process is crucial for tasks like image denois
0 views • 50 slides
PageRank Algorithm: A Comprehensive Overview
The PageRank algorithm plays a crucial role in determining the importance of web pages based on link structures. Jeffrey D. Ullman from Stanford University explains the concept of PageRank using random surfer model and recursive equations, emphasizing the principal eigenvector of the transition matr
1 views • 55 slides
Linear Equations and Matrix Operations
Explore the concepts of linear equations, matrix forms, determinants, and finding solutions for variables like x1, x2, x3. Learn about Cramer's Rules, Adjoint Matrix, and calculating the inverse of a matrix through examples and formulas.
1 views • 24 slides
Multiserver Stochastic Scheduling Analysis
This presentation delves into the analysis and optimality of multiserver stochastic scheduling, focusing on the theory of large-scale computing systems, queueing theory, and prior work on single-server and multiserver scheduling. It explores optimizing response time and resource efficiency in modern
0 views • 38 slides
Approximation Algorithms for Stochastic Optimization: An Overview
This piece discusses approximation algorithms for stochastic optimization problems, focusing on modeling uncertainty in inputs, adapting to stochastic predictions, and exploring different optimization themes. It covers topics such as weakening the adversary in online stochastic optimization, two-sta
0 views • 33 slides
Optimal Sustainable Control of Forest Sector with Stochastic Dynamic Programming and Markov Chains
Stochastic dynamic programming with Markov chains is used for optimal control of the forest sector, focusing on continuous cover forestry. This approach optimizes forest industry production, harvest levels, and logistic solutions based on market conditions. The method involves solving quadratic prog
2 views • 27 slides
Integrating Stochastic Weather Generator with Climate Change Projections for Water Resource Analysis
Exploring the use of a stochastic weather generator combined with downscaled General Circulation Models for climate change analysis in the California Department of Water Resources. The presentation outlines the motivation, weather-regime based generator description, scenario generation, and a case s
1 views • 20 slides
Revolutionizing Hotel Communication with Matrix Hospitality Solution
Simplify hotel operations and enhance guest experiences with Matrix Hospitality Solution. From enhancing staff efficiency to boosting revenue generation opportunities, Matrix offers a comprehensive suite of features to meet the diverse needs of hotels. Its modular configuration, scalable platform, a
1 views • 36 slides
Composite Matrix Materials in Engineering
Composite materials are made of reinforcing fibers and matrix materials, with the matrix serving to protect and enhance the properties of the composite. There are three main types of composite matrix materials: metal matrix composites (MMC), ceramic matrix composites (CMC), and polymer matrix compos
0 views • 22 slides
Stochastic Differential Equations and Numerical Integration
Explore the concepts of Brownian motion, integration of stochastic differential equations, and derivations by Einstein and Langevin. Learn about the assumptions, forces, and numerical integration methods in the context of stochastic processes. Discover the key results and equations that characterize
0 views • 6 slides
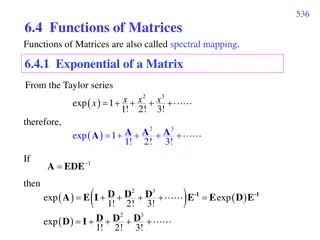
Matrix Functions and Taylor Series in Mathematics
A detailed exploration of functions of matrices, including exponential of a matrix, eigenvector sets, eigenvalues, Jordan-Canonical form, and applications of Taylor series to compute matrix functions like cosine. The content provides a deep dive into spectral mapping, eigenvalues, eigenvectors, and
0 views • 53 slides
Introduction to Generalized Stochastic Petri Nets (GSPN) in Manufacturing Systems
Explore Generalized Stochastic Petri Nets (GSPN) to model manufacturing systems and evaluate steady-state performances. Learn about stochastic Petri nets, inhibitors, priorities, and their applications through examples. Delve into models of unreliable machines, productions systems with priorities, a
0 views • 44 slides
Parallel Computation for Matrix Multiplication
Matrix multiplication is a fundamental operation with diverse applications across scientific research. Parallel computation for matrix multiplication involves distributing the computational workload over multiple processors, improving efficiency. Different algorithms have been developed for multiply
1 views • 36 slides
Stochastic Algorithms: Monte Carlo and Las Vegas Variations
Stochastic algorithms, including Monte Carlo and Las Vegas variations, leverage randomness to tackle complex tasks efficiently. While Monte Carlo algorithms prioritize speed with some margin of error, Las Vegas algorithms guarantee accuracy but with variable runtime. They play a vital role in primal
1 views • 13 slides
Optimal Early Drought Detection Using Stochastic Process
Explore an optimal stopping approach for early drought detection, focusing on setting trigger levels based on precipitation measures. The goal is to determine the best time to send humanitarian aid by maximizing expected rewards and minimizing expected costs through suitable gain/risk functions. Tas
0 views • 4 slides
Optimizing User Behavior in Viral Marketing Using Stochastic Control
Explore the world of viral marketing and user behavior optimization through stochastic optimal control in the realm of human-centered machine learning. Discover strategies to maximize user activity in social networks by steering behaviors and understanding endogenous and exogenous events. Dive into
0 views • 15 slides
Tradeoff between Sample and Space Complexity in Stochastic Streams
Explore the relationship between sample and space complexity in stochastic streams to estimate distribution properties and solve various problems. The research delves into the tradeoff between the number of samples required to solve a problem and the space needed for the algorithm, covering topics s
0 views • 23 slides
Efficient Training of Dense Linear Models on FPGA with Low-Precision Data
Training dense linear models on FPGA with low-precision data offers increased hardware efficiency while maintaining statistical efficiency. This approach leverages stochastic rounding and multivariate trade-offs to optimize performance in machine learning tasks, particularly using Stochastic Gradien
0 views • 26 slides
Evolution of Universes in Causal Set Cosmology Analysis
Causal sets propose a discrete and dynamical spacetime structure, where spacetime elements, called spacetime atoms, evolve through stochastic dynamics. This growth process governs the passage of time, manifesting as accretion or birth of new elements. Classical Sequential Growth Models offer a frame
0 views • 17 slides
ROBUST STOCHASTIC APPROXIMATION APPROACH TO STOCHASTIC PROGRAMMING
Discussed are stochastic optimization problems, including convex-concave saddle point problems. Solutions like stochastic approximation and sample average approximation are analyzed. Theoretical assumptions and notations are explained, along with classical SA algorithms. Further discussions delve in
0 views • 41 slides
Uncertainty Estimation in Hydrology: Incorporating Physical Knowledge in Stochastic Modeling
The presentation discusses the longstanding issue of uncertainty estimation in hydrology and the importance of incorporating physical knowledge in stochastic modeling of uncertain systems. It highlights the role of expert judgment and how uncertainty will always be present in hydrological assessment
0 views • 31 slides
Computer Simulation Models Classification
Computer simulation models are classified based on various characteristics such as static or dynamic, deterministic or stochastic, and discrete or continuous. Static models represent systems at a specific point in time, while dynamic models depict changes over time. Deterministic models involve no r
0 views • 8 slides
Learned Feedforward Visual Processing Overview
In this lecture, Antonio Torralba discusses learned feedforward visual processing, focusing on single layer networks, multiple layers, training a model, cost functions, and stochastic gradient descent. The content covers concepts such as forward-pass training, network outputs, cost comparison, and p
0 views • 32 slides
Matrices Multiplication: Understanding Matrix Dimensions and Operations
In matrix multiplication, understanding the dimensions of matrices is crucial for determining the feasibility of the operation. This involves multiplying corresponding elements of rows and columns to obtain the final matrix with specific dimensions. The order of multiplication matters, as changing i
1 views • 39 slides
Large Matrix-Matrix Multiply on PS3 Clusters - September 2010 Study
Matrix-matrix multiplication of large matrices over PS3 clusters, achieving high computational efficiency and GFLOPS performance. Challenges, approach, and results of the study are discussed in detail.
0 views • 5 slides
Optimizing Response Time Through Stochastic Scheduling
This article explores stochastic scheduling with predictions, aiming to minimize mean response time. It discusses the use of uniform bounds for scheduling with job size estimates and the significance of stochastic analysis in overcoming worst-case barriers. The study delves into two approaches - wor
0 views • 20 slides
Stochastic Programming in ATO Inventory Systems: Evolution and New Ideas
Marty Reiman's Markov lecture discussed a stochastic programming-based approach to ATO inventory systems, highlighting new frontiers and emerging ideas in the field. Structural and optimization results from selected literature were also reviewed, emphasizing the need for innovative approaches to inv
0 views • 16 slides
Effect of Bit-Level Correlation in Stochastic Computing
Impact of bit-level correlation in stochastic computing and its implications on system efficiency and performance. This study delves into the theoretical and simulated results, highlighting the properties and applications of stochastic computing. The research also analyzes previous works and aims to
0 views • 24 slides
Advanced Stochastic Local Search Techniques
Explore advanced stochastic local search algorithms such as Tabu Search, Simulated Annealing, Genetic Algorithms, and Ant Colony Optimization for solving combinatorial optimization problems. Understand the basic concepts, principles, and origins of Tabu Search, and discover how it utilizes intellige
0 views • 44 slides
Analysis and Design of Wireless Networks with Stochastic Geometry
Explore the application of stochastic geometry and random graphs in the analysis and design of wireless networks, focusing on SNR, SINR, Poisson point processes, random graph models, and interference characterization.
0 views • 15 slides
Efficient Multiparty Computation for Matrix Rings against Malicious Adversaries
Explore the development of efficient multiparty computation protocols for non-commutative rings, specifically focusing on matrix rings, in the dishonest majority setting to combat malicious adversaries. This study, presented at ASIACRYPT 2024, delves into the significance of utilizing matrix rings i
0 views • 19 slides
Introduction to Stochastic Network Calculus in Electrical and Computer Engineering
Explore the world of Stochastic Network Calculus in the Department of Electrical and Computer Engineering at Xidian University. Learn about Network Calculus, Queueing Theory, and the foundations laid by R. Cruz. Discover how Deterministic and Stochastic Network Calculus provide different levels of s
0 views • 43 slides