Exploring Mathematics: Definitions, Principles, and Applications
Mathematics is the science of number, space, quantity, and measurement, providing a logical study of shape, arrangement, and quantity. This lesson delves into the meaning and definitions of mathematics, emphasizing its systematic and exact nature. It covers quantitative facts, spatial relations, and
1 views • 32 slides
Empower Your Future Ace the DSST Business Mathematics Exam
Seize your opportunity for success with our comprehensive study resources for the DSST Business Mathematics Exam. Access practice tests, study guides, and expert guidance to excel in your test. Start your journey towards academic and career advancement today!\nClick Here to Get Business-Mathematics
0 views • 8 slides
Maximize Success Dominate the IB Mathematics (SL) Examination
Achieve excellence in the IB Mathematics (SL) Examination with our comprehensive preparation resources. Get expert guidance, practice questions, and strategies to excel in your exam. Start your journey towards success now!\nClick Here to Get IB-Mathematics Dumps With 16 USD Discount Code: NB4XKTMZ\n
2 views • 8 slides
Polynomial-time Pseudodeterministic Construction of Primes and Motivational Challenges
Exploring the challenges and advancements in generating prime numbers, particularly focusing on a pseudodeterministic construction method within polynomial time. The discussion includes reviewing previous approaches, fundamental computational problems related to primes, motivational problem statemen
0 views • 40 slides
General Mathematics Study Design 2023-2027 Overview
The General Mathematics Study Design for 2023-2027 includes prescribed content for Units 1 and 2, covering areas such as data analysis, probability, algebra, functions, discrete mathematics, and more. The study design aims to provide a structured framework for students in understanding mathematical
1 views • 32 slides
A-Level Mathematics and Further Mathematics Overview
A-Level Mathematics covers core content along with Pure Mathematics, Statistics, and Mechanics components. Further Mathematics includes Pure Mathematics, Mechanics, Statistics, and Decision Mathematics. Emphasis is placed on correct notation, proof, and utilizing calculators like Casio 991-EX for co
0 views • 17 slides
Importance of Mathematics Education and Pedagogy
Mathematics plays a crucial role in quantifying ideas, fostering logical thinking, problem-solving, and enhancing critical thinking skills. Mathematics education helps develop competencies in learners through learner-friendly pedagogies, ensuring progress and achievement of learning outcomes. The na
0 views • 16 slides
Introduction to Arithmetic Operations on Polynomials
This unit focuses on developing an understanding of polynomials in mathematical expressions. You will learn about the parts of a polynomial, polynomial operations, and representing polynomials. The topics cover performing arithmetic operations on polynomials, identifying variables in expressions, le
1 views • 71 slides
Overview of VCE Specialist Mathematics 2023-2027 Study Design
The VCE Specialist Mathematics program for 2023-2027 study design aims to enhance students' mathematical skills through prescribed Units 1-4. This comprehensive curriculum covers topics such as algebra, discrete mathematics, space and measurement, functions, data analysis, and more. The course struc
0 views • 26 slides
Understanding Polynomial Functions and Operations
Polynomial functions are mathematical functions in the form of an expression involving variables and coefficients. They can be manipulated through operations like addition, subtraction, multiplication, and division. Learn about polynomial degrees, identifying polynomials, and performing various oper
0 views • 20 slides
Understanding Polynomial Degrees and Special Names
The degree of a polynomial is determined by its highest exponent, with specific names for each degree level. From the basic constant to the nth degree polynomial, this guide showcases the different degrees and their characteristics, helping you grasp the concept of polynomial functions easily.
0 views • 18 slides
Understanding Polynomials: Types, Degrees, and Zeroes
Polynomial expressions consist of terms with non-zero coefficients. They can have any number of terms and different degrees. Linear polynomials have a degree of one, quadratic polynomials have a degree of two, and cubic polynomials have a degree of three. Zeroes of a polynomial are the values of the
0 views • 17 slides
Understanding Polynomial Functions with Real Zeros
Learn how to identify and write polynomial functions that include real zeros, find zeros of given functions, explore the Fundamental Theorem of Algebra, and apply the Number of Zeros Theorem. Practice writing polynomial functions satisfying specific conditions.
0 views • 11 slides
Understanding Polynomials and Graphs through Real-World Analogies
Explore the relationship between mountain ranges and polynomials, and learn how to apply the Intermediate Value Theorem to find zeros of polynomial functions. This guide covers concepts like the Interval Value Theorem, sketching graphs of higher-degree polynomials, and constructing tables to analyze
3 views • 85 slides
Understanding PISA Mathematics Assessment
PISA (Programme for International Student Assessment) evaluates 15-year-old students' preparedness in reading, mathematics, and science literacy. The mathematics assessment focuses on mathematization as a five-stage process, starting from real-world problems to mathematical solutions. The assessment
0 views • 39 slides
Writing Polynomial Functions: A Comprehensive Guide
Understand how to write polynomial functions by identifying zeros, conjugate pairs, and factors from graphs. Learn how to translate zeroes into factors, consider leading coefficients, and determine function forms from different types of graph interactions. Examples provided for practical application
0 views • 4 slides
René Descartes: Philosopher and Mathematician Extraordinaire
René Descartes, known as the Father of Modern Philosophy, made significant contributions to both philosophy and mathematics. Born in 1596 in France, Descartes received a Jesuit education and later worked as a Corps of Engineers applying mathematics to practical applications. His philosophical works
0 views • 15 slides
The Vital Role of Mathematics in Engineering Education
Engineers require a solid foundation in mathematics to tackle complex problems effectively. Mathematics is a fundamental tool that is intertwined with all engineering disciplines, emphasizing abstract thinking and problem-solving skills. At DTU, mathematics courses for B.Eng. students cover topics l
2 views • 21 slides
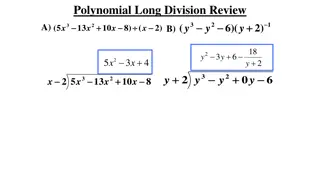
Polynomial Long Division Review and Practice
This content provides a detailed review on polynomial long division including step-by-step instructions, examples, and synthetic division practice problems. It covers topics such as descending polynomial order, solving binomial divisors, writing coefficients, determining remainders, and obtaining fi
0 views • 4 slides
Polynomial Division Methods and Examples
Dividing polynomials involves using methods like long division or equating coefficients. By applying these techniques, you can determine whether a polynomial divides exactly or leaves a remainder. The process is similar to long division of numbers, where the dividend is divided by the divisor to obt
0 views • 10 slides
Polynomial Division and Remainder Theorems Explained
Learn how to use long division to find quotients and remainders in polynomial problems. Understand when to use long division or synthetic division. Discover how the remainder theorem works by finding remainders when dividing specific polynomials by different factors. Explore the factor theorem and i
0 views • 6 slides
Polynomial and Synthetic Division Techniques
Learn how to perform polynomial division using long division and synthetic division methods. Understand how to divide polynomials by other polynomials or binomials, utilize the Remainder Theorem and Factor Theorem, and apply these concepts through detailed examples.
0 views • 41 slides
Strong List Coloring and the Polynomial Method in Graph Theory
Exploring the Polynomial Method in the context of Strong List Coloring, Group Connectivity, and Algebraic tools. This method involves proper coloring of graphs based on polynomial assignments, highlighting the significance of Strong Choosability and the Co-graphic case. The applications and proofs a
0 views • 34 slides
Understanding Polynomial Identity Testing in Algorithm Design
Explore the concept of polynomial identity testing as a powerful tool in algorithm design. Learn how to determine if a polynomial is identically zero by choosing random points and applying the Schwartz-Zippel Lemma. Discover the application of this technique in finding perfect matchings in bipartite
0 views • 26 slides
Approximating Knapsack Problem in Polynomial Time
In the recent discussion, we explored approximating the Knapsack problem in fully polynomial time. By utilizing a polynomial-time approximation scheme (PTAS), we aim to find a set of items within a weight capacity whose value is within a certain range of the optimal value. This approach involves lev
0 views • 22 slides
Understanding Signatures, Commitments, and Zero-Knowledge in Lattice Problems
Explore the intricacies of lattice problems such as Learning With Errors (LWE) and Short Integer Solution (SIS), and their relation to the Knapsack Problem. Delve into the hardness of these problems and their applications in building secure cryptographic schemes based on polynomial rings and lattice
0 views • 44 slides
Understanding Decision Problems in Polynomial Time Complexity
Decision problems play a crucial role in computational complexity theory, especially in the context of P and NP classes. These problems involve questions with yes or no answers, where the input describes specific instances. By focusing on polynomial-time algorithms, we explore the distinction betwee
0 views • 32 slides
Lower Bounds for Small Depth Arithmetic Circuits
This work explores lower bounds for small-depth arithmetic circuits, jointly conducted by researchers from MSRI, IITB, and experts in the field. They investigate the complexity of multivariate polynomials in arithmetic circuits, discussing circuit depth, size, and the quest for an explicit family of
0 views • 114 slides
Advanced Techniques in Secret Sharing Schemes
Explore the advancements in polynomial secret-sharing schemes and their applications in cryptography. Discover how polynomial schemes provide efficient solutions for sharing secrets among multiple parties while maintaining security. Learn about the construction of polynomial conditional disclosure p
0 views • 16 slides
Innovative Mathematics Education Initiatives at Brookhill Institute
Brookhill Institute of Mathematics in Waukesha, WI, is a hub for pioneering educational programs focused on higher education, teacher preparation, and professional development in mathematics. The institute collaborates with various educational institutions and experts to enhance mathematics teaching
0 views • 33 slides
Revisiting Adjustor Curves for Total Phosphorus Removal Rates
Based on a literature review, it was found that a 5th-order polynomial curve is a better fit than the originally used logarithmic trendline for anchor rates of percent Total Phosphorus removal related to runoff depth. The expert panel report reflects the old curves while trendline equations in FAQ d
0 views • 7 slides
Understanding Rational Functions Through Divided Differences and Newton Polynomial
Explore the mathematical approach of using divided differences and Newton Polynomial to determine an equation for a rational function passing through given points. The process involves creating a system of linear equations and utilizing Newton Polynomial to establish relationships between points. Va
0 views • 20 slides
Polynomials Operations: Adding, Subtracting, Multiplying - Unit 7 Days 1 and 2
Dive into the world of polynomial operations in this engaging unit covering adding, subtracting, and multiplying polynomials. Explore methods to combine like terms, distribute negative signs, and apply polynomial operations to solve problems. Practice sorting gumballs with like terms and creating nu
0 views • 23 slides
Understanding P, NP, NP-Hard, NP-Complete Problems and Amortized Analysis
This comprehensive study covers P, NP, NP-Hard, NP-Complete Problems, and Amortized Analysis, including examples and concepts like Reduction, Vertex Cover, Max-Clique, 3-SAT, and Hamiltonian Cycle. It delves into Polynomial versus Non-Polynomial problems, outlining the difficulties and unsolvability
0 views • 32 slides
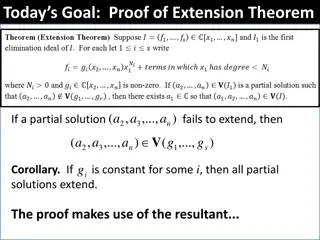
Understanding the Extension Theorem in Polynomial Mathematics
Explore the proof of the Extension Theorem, specializing in resultant calculations of polynomials and their extensions. Learn about Sylvester matrices, resultants, and how to make conjectures based on polynomial interactions. Take a deep dive into specializations and their implications in polynomial
0 views • 14 slides
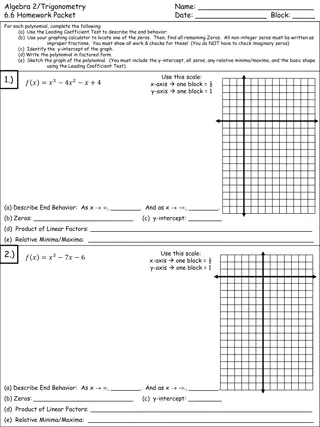
Polynomial Analysis Homework Packet
Complete polynomial analysis including end behavior description, locating zeros, finding y-intercepts, factoring, and sketching graphs for given polynomials in a homework packet. Utilize the leading coefficient test and graphing calculator to identify zeros and graph features accurately.
0 views • 4 slides
Understanding Zeroes of Polynomials - Grade 9 Chapter 2
Zeroes of a polynomial are the values of the variable that make the polynomial equal to zero. This concept is explored in Grade 9 Chapter 2, where students learn how to find the zeroes of a polynomial by equating it to zero. Through examples like p(x) = x - 4, students understand how to determine th
0 views • 10 slides
Understanding Multivariate Cryptography Schemes
Multivariate cryptography involves systems of polynomial equations, with public keys based on polynomial functions. GeMSS and Rainbow are discussed, highlighting their design features and vulnerabilities. The Butterfly Construction method in multivariate schemes constructs public keys using easily i
0 views • 33 slides
Classical Algorithms from Quantum and Arthur-Merlin Communication Protocols
Explore the Polynomial Method in classical algorithms, focusing on Orthogonal Vectors, All-Pair-Shortest-Path, and Approximate Closest Pair. Learn how the Polynomial Method works through batch evaluation for multi-variable polynomials and fast matrix multiplication. Discover insights on low-rank dec
0 views • 13 slides
Algebraic Complexity and Equational Proofs in Arithmetic Formulas
Explore the intricacies of polynomial identity testing (PIT), equational proofs, and arithmetic formulas in the context of algebraic complexity. Learn about the minimal number of operations needed to compute the zero polynomial and derive new identities using derivation rules and axioms in polynomia
0 views • 39 slides