Writing Polynomial Functions: A Comprehensive Guide
Understand how to write polynomial functions by identifying zeros, conjugate pairs, and factors from graphs. Learn how to translate zeroes into factors, consider leading coefficients, and determine function forms from different types of graph interactions. Examples provided for practical application.
Uploaded on Sep 07, 2024 | 0 Views
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author.If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
You are allowed to download the files provided on this website for personal or commercial use, subject to the condition that they are used lawfully. All files are the property of their respective owners.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author.
E N D
Presentation Transcript
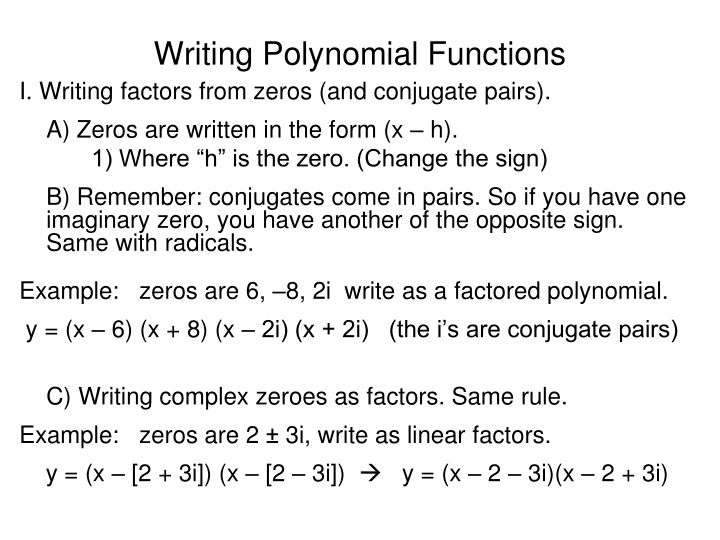
Writing Polynomial Functions I. Writing factors from zeros (and conjugate pairs). A) Zeros are written in the form (x h). 1) Where h is the zero. (Change the sign) B) Remember: conjugates come in pairs. So if you have one imaginary zero, you have another of the opposite sign. Same with radicals. Example: zeros are 6, 8, 2i write as a factored polynomial. y = (x 6) (x + 8) (x 2i) (x + 2i) (the i s are conjugate pairs) C) Writing complex zeroes as factors. Same rule. Example: zeros are 2 3i, write as linear factors. y = (x [2 + 3i]) (x [2 3i]) y = (x 2 3i)(x 2 + 3i)
Writing Polynomial Functions II. Writing factors given the degree and the leading coefficient. A) Remember: zeros are written in the form (x h). 1) Where h is the zero. (Change the sign) B) But we want the leading term to be the value a . 1) So write it as a(x h1)(x h2)(etc.) 2) Distribute the a term to any ONE of the (x h). 3) If you need it in standard form, FOIL all the (x h)s. Example: Leading coeff = 5; Degree 3; zeroes of 2, 3/5 & 5(x 2) (x 3/5) (x + ) distribute the 5 to (x 3/5) to get (x 2) (5x 3) (x + ) and then FOIL (x 2)(5x 3) to get (5x2 13x + 6)(x + ) and distribute for standard form of f(x) = 5x3 21/2x2 x + 3
Writing Polynomial Functions III. Writing factors from a graph. A) Find the zeroes of the graph (the x-intercepts). 1) Change the signs to get them into (x h) form. B) Look at the x-intercepts. Did it bounce or pass through? 1) Bounces mean (x h)2[or other even exponent]. 2) Pass through means simply (x h) if the graph went straight through the x-intercept. a) If the graph flattened out at the x-intercept before passing through then you have (x h)odd. C) To get the leading coefficient a , write the function in factored form with the a in front of the factors, then find any point on the graph and plug those values in for x and y. Solve for a to find your leading coefficient. D) If you don t know any x-intercepts, try to write the equation in shifted format. y = A f (B(x C)) + D.
Writing Polynomial Functions Example 1: A bounce at -3, a pass thru at 5 and the y-intercept is (0,-5). Write the equation in factored form. The bounce means an even exponent (2). y = a(x + 3)2(x 5) plug in (0,-5) -5 = a(3)2(-5) -5 = -45a solve for a and you get 1/9 so f(x) = 1/9(x + 3)2(x 5) Example 2: A bounce at 4, a flattened pass thru at -2 and the y-intecept is (0, 7). Write the equation in factored form. The bounce is (x-h)even, the flattened pass thru is (x-h)odd y = a(x 4)2(x+2)3plug in (0,-7) -7 = a(-4)2(2)3 -7 = 128a solve for a and you get = 1/16 so f(x) = 1/16(x 4)2(x+2)3 Example 3: The graph has no known x-intercepts, but you know the graph is cubic (opposite end directions) and there is a y-int at (0,19) and flattened value at (-2,-5). Shift rules y = a(x+2)3 5 plug in (0 , 19) 19 = a(2)3 5, solve for and you get 3 so f(x) = 3(x + 2)3 5