Understanding Reactor Start-up and Neutron Multiplication in Subcritical Reactors
Reactor start-up is a crucial operation that transitions a reactor from a subcritical state to a critical state by carefully adjusting reactivity levels. In a subcritical reactor, the neutron density should decrease over time but may not reach zero due to certain factors. Neutron multiplication factors play a key role in reactor operation, determining the neutron population in each generation. This process is essential for maintaining nuclear safety and operational efficiency.
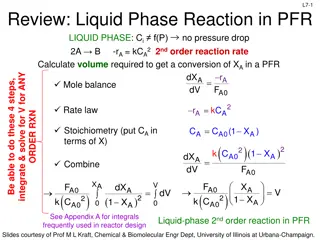
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
When a reactor does not operate, it is in a subcritical state (K <1). All control rods are submerged into the core. In the absence of external sources of neutrons, there are virtually no neutrons in the core. When shutdown time of the reactor ends, the reactor is put into operation again. 2
Reactor start up - an operation which brings a reactor out of the subcritical state to the critical by means of careful rise of compensation units of reactivity in a critical state or reduction of boric acid concentration in the water of the primary circuit to a critical value. 3
Reactor start-up - one of the most important operations of control and protection systems (CPS) of the reactor. Start-up is performed by operations with the reactor, in the sub-critical state, i.e. at < 0 or eff< 1. 4
Reactor start up is carried out from deep subcritical state by gradual extracting of regulating units and reactivity compensation until reaching criticality with the unconditional enforcement of nuclear safety rules in relation to the rate of the process. In a subcritical reactor after a sufficiently long time neutron density should fall off asymptotically to zero. But in reality this does not happen! WHY? 5
Neutron source in a subcritical reactor Effective neutron multiplication factor in the reactor is the ratio of the numbers of neutrons of the considered generation and its previous generation. 6
One can imagine a critical reactor in the core of which there are no neutrons. If so, then in the first, second, third and in all subsequent generations, neutrons should not appear in the reactor: n1= n2= n3= ... = ni= 0. 7
If at least one neutron is given to the core of such critical reactor from the outside, this neutron will be reproduced in any generation: n1= n2= n3= ... = ni= 1. 8
If 2, 3, 4 or in principle any number of neutrons ... are sequentially inserted to the reactor core, the core will properly reproduce the inserted number of neutrons from generation to generation. The density of neutrons in such a critical core will be stabilized in time to a level determined by the dimensions of the core and the number of neutrons inserted to the core. 9
If neutron groups are evenly inserted to the core, in the process of this even time insertion of neutrons to the core of the critical reactor, the value of neutron density in the core will increase linearly with intensity determined by insertion rate of neutrons to the core. 10
We can conclude that if there are no neutron sources in the reactor core, even critical by its intrinsic properties reactor will not operate. Moreover, subcritical reactor would not operate without the so-called start-up neutrons: there is nothing to multiply in the core. 11
Also, if the reactor is subcritical (K <1), after a sufficiently long time neutron density in the reactor should asymptotically decrease to zero, which does not happen actually. What is it - the error of kinetic equations or just some unaccounted factor in them? 12
It turned out - the last one. And this unaccounted factor is related to the presence of various neutrons sources in the reactor independent of the fission reaction in it. What kind of sources? 13
) Neutrons of cosmic radiation There is a neutron component in the composition of cosmic showers. Part of cosmic neutron radiation passes through Earth's atmosphere and reaches its surface. Some of these neutrons, due to their high penetrating ability, can fall into the reactor core and cause fission of fuel nuclei. 15
It is important for us that cosmic neutrons as a source for subcritical reactor have more fundamental than practical significance: in most favorable conditions, only a few neutrons may penetrate into the core during an hour. 16
b) Spontaneous fission neutrons Spontaneous fission was discovered in 1940. At first it was believed that neutrons emitted from pieces of uranium metal and uranium compounds are conventional fission neutrons, obtained in the fission of uranium nuclei under the influence of cosmic neutrons. However, the experiments showed that these fissions occur spontaneously. This type of fission was called spontaneous fission. 17
The rate of spontaneous fission is subject to the same law as radioactive decay that is proportional only to the total present amount of spontaneously fissile nuclei at a given time. In particular, for two of the most important for us (235U and 238U), this law is written as: dN5/ dt = - 5spN5(t) dN8/ dt = - 8spN8(t) 18
Studies have revealed a strange feature: the value of spontaneous fission constant for 235U ( 5sp 1.156.10- 25s-1) was approximately 24 times less than spontaneous fission constant of 238U ( 8sp 2.745.10-24s-1), although cross section of "conventional" (forced) fission of 235U is many times greater than that of 238U. 19
An elementary calculation shows that in every kilogram of natural uranium only 23 spontaneous fissions occur within one hour. In the reactor with loading of 80 tonnes of uranium into core about 1070 spontaneous neutrons are emitted every second. 20
According to these numbers we can conclude that spontaneous fission - a more powerful source of neutrons in subcritical reactor, than neutrons of the cosmic radiation, but for a large core - source is rather weak. 21
c) Neutrons, emitted in the reactor in reactions ( , n), ( , n) and (n,2n) In the core of a power reactor, after its relatively short operation significant amounts of - and -active fission fragments accumulate in the fuel elements, characterized by different half-lives. They continue to emit a-particles and -quanta, causing the above-mentioned types of nuclear reactions with fuel nuclei and some other fission fragments. 22
Therefore, in the subcritical reactor core after operation there are always neutron sources scattered by the volume of its fuel elements, which are much more powerful than spontaneous fission. This source is absent in the reactor at the beginning of a campaign. 23
Therefore, to ensure safe reactor start-up at the physical start-up, artificial neutron sources have to be lowered into a freshly loaded core. But to ensure a safe start-up at any time of the campaign, this power source is insufficient. 24
Conclusion: In the core of a subcritical reactor there are always natural neutron sources, operating independently from multiplying properties of the core. These sources are low-powered, but they make reactor start-up possible without the use of artificial neutron sources. 25
Neutron density in a subcritical reactor If external neutron source (S) is inserted to the core of a subcritical reactor, the total number of neutrons in the reactor will be more than the source emits. This is due to the fact that neutrons of source cause fission of nuclear fuel (235U, 239Pu). 26
Consider the case where in the medium there is external neutron source (S) of constant power, not associated with the fission reaction in the medium. Neutrons of this source are evenly distributed in space and generate S neutrons continuously in each unit volume (1 cm3) per second. 27
If a reactor has an independent neutrons source (for example, for simplicity - a source of constant specific power in S neutrons /cm3), it seems obvious that the value of the neutron density should not be reduced to zero, since this constantly functioning source will not give it to fall to zero. So what can be expected in this case? 28
When the rate of the neutron density decrease due to subcritical reactor equals the rate of neutron density increase from the source, neutron density decrease in the reactor should stop. And the value of the neutron density in the reactor should stabilize at a certain level. This value of the average neutron density in the subcritical reactor, which is established in it over time, will be called establishing subcritical density. 29
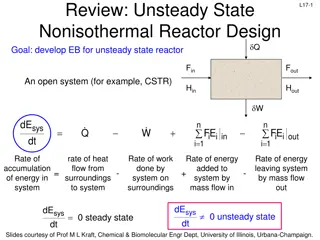
The number of neutrons in the point reactor can be determined based on the system of equations of the so-called point kinetics: K dN N i eff l = + + + ( * * ) N l C S eff i i dt l * * N K dC , eff i eff = i C i i dt l 30
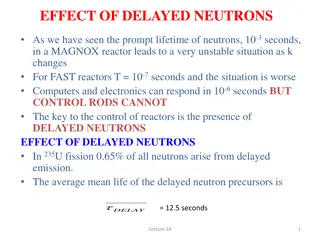
where: N average number of neutrons in the reactor; Keff -effective multiplication factor; l- average neutron lifetime in the reactor; Ci- emitter concentration of delayed neutrons of i group; i- decay constant of emitters of delayed neutrons of i- group; eff,i- fraction of delayed neutrons of i-group. 31
To describe all the critical and supercritical states, the equivalent system of equations is much more widespread, which describes the processes by the variable of reactivity =( ef-1)/ ef 32
This system can be written in the form which is almost universal: ( N dt i N dt ) dN eff = + + C S i i dC eff , i = i C i i Where: N - average number of neutrons in the reactor; - average time of neutron generation; 33
In stationary states (when the derivatives are equal to zero) the system of equations are reduced to the following simple relations: n + = ( ) * 0 Keff l S The same can be expressed in terms of reactivity: N = -S or = - S / N. l 34
In subcritical reactor neutron power level is associated with the value of the criticality K or reactivity by the source power, hen l Keff = S l N or: S l =1 N K eff 35
? ? ? ? = 1 ?= ? ? ? 1 where: ? = is called multiplication factor (subcritical multiplication factor). The meaning of this name can be illustrated by the dependence diagram of the value of establishing neutron density in the reactor on the value of subcriticality degree at different power values of a subcritical neutron source. 1 ? k = 1- 36
Dependence of establishing subcritical neutron density on the value of reactor degree subcriticality at three different power values of the independent neutron source n n /n =Subcritical multiplication factor at s3 s2 at s2 s1 n 3 n 21 n 1 at s1 0 k 0k 1 1 k 1 0 k 1 0 37
Dependence of establishing subcritical neutron density on the value of reactor degree subcriticality (in a dimensionless form). 38
Follows from the form this dependence, risk of the start-up procedure becomes clear: If we approach the critical state by the change steps of subcriticality degree which are equal in value, with each successive step of reduction k , difference of establishing values of neutron density increases still more and more, and value of the establishing neutron density tends to very large values. 39
Establishing neutron flux in the subcritical reactor Keffin a subcritical reactor - a value less than unity. This means that as soon as the reactor has become subcritical, neutron density in it is decreasing steadily from generation to generation, asymptotically approaching zero. 41
But if the reactor has an independent source of neutrons (S n/cm3), the neutron density value should not be reduced to zero because constant functioning source doesn t allow it to fall to zero. What happens in this case? 42
When the rate of neutron density decrease due to reactor subcriticality equals the rate of neutron density increase from the source, neutron density decrease in the reactor should be stopped, and the value of neutron density in the reactor should stabilize at a certain level. 43
The value of average neutron density in a subcritical reactor, which is established in it over time, is called establishing subcritical neutron flux density. 44
With regard to the subcritical reactor with a neutron source, elementary equation of reactor kinetics is: positive term is added to the right side - rate of neutron increase from the source S, representing specific power value of the neutron source. k l dn dt = + ( ) , n t s 45
In subcritical reactor, the value of excess multiplication factor k is negative. To make it clear that we are talking about a subcritical reactor, positive value of a lack of the effective factor value to the unit in a subcritical reactor ( k ) is called reactor subcriticality degree: k = 1 - k = - k 46
Then kinetics equation is: dn dt k l = + ( ) . n t s If to put the multiplier before n(t) on the right side of the brackets, then: 47
When the value of neutron density in subcritical reactor is established constant, the value of the derivative dn/dt = 0, Then we can obtain: This implies: 48
From this formula it follows that: the greater the value of establishing neutron density in a subcritical reactor: a) the greater the power value of independent neutron source. b) the greater the value of the average neutron generation lifetime in the reactor (l). c) the lower the degree of reactor subcriticality degree ( ). 49
In the numerator of the expression there is a value of the product sl with neutron density dimension (neutrons / cm3), which has the meaning of establishing neutron density at the value of subcriticality degree ks= 1. This value is called initial establishing neutron density in the reactor at a given power of subcritical source S, n 0= s l 50