Insights into Quasiparticles in Normal and Superfluid Fermi Liquids
This content discusses quasiparticles in normal and superfluid Fermi liquids, covering topics such as the Landau Fermi-liquid idea, BCS theory, Bogoliubov-de Gennes approach, Majorana fermions, conservation quantities in liquids, consequences of conservation for response functions, and applications to cuprates. It delves into the behavior of quasiparticles in fully interacting systems and the implications for understanding Fermi liquids, including incoherent backgrounds and contributions to correlation functions.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
QUASIPARTICLES IN NORMAL AND SUPERFLUID FERMI LIQUIDS Tony Leggett Department of Physics University of Illinois Urbana-Champaign partly joint work with Yiruo Lin 1. (Brief) review of Landau Fermi-liquid idea 2(a). Quasiparticles in the superfluid state: BCS theory Quasiparticles in the superfluid: Bogoliubov- de Gennes approach 3. Majarana fermions (orthodox approach) 4. Majarana fermions (doubts)
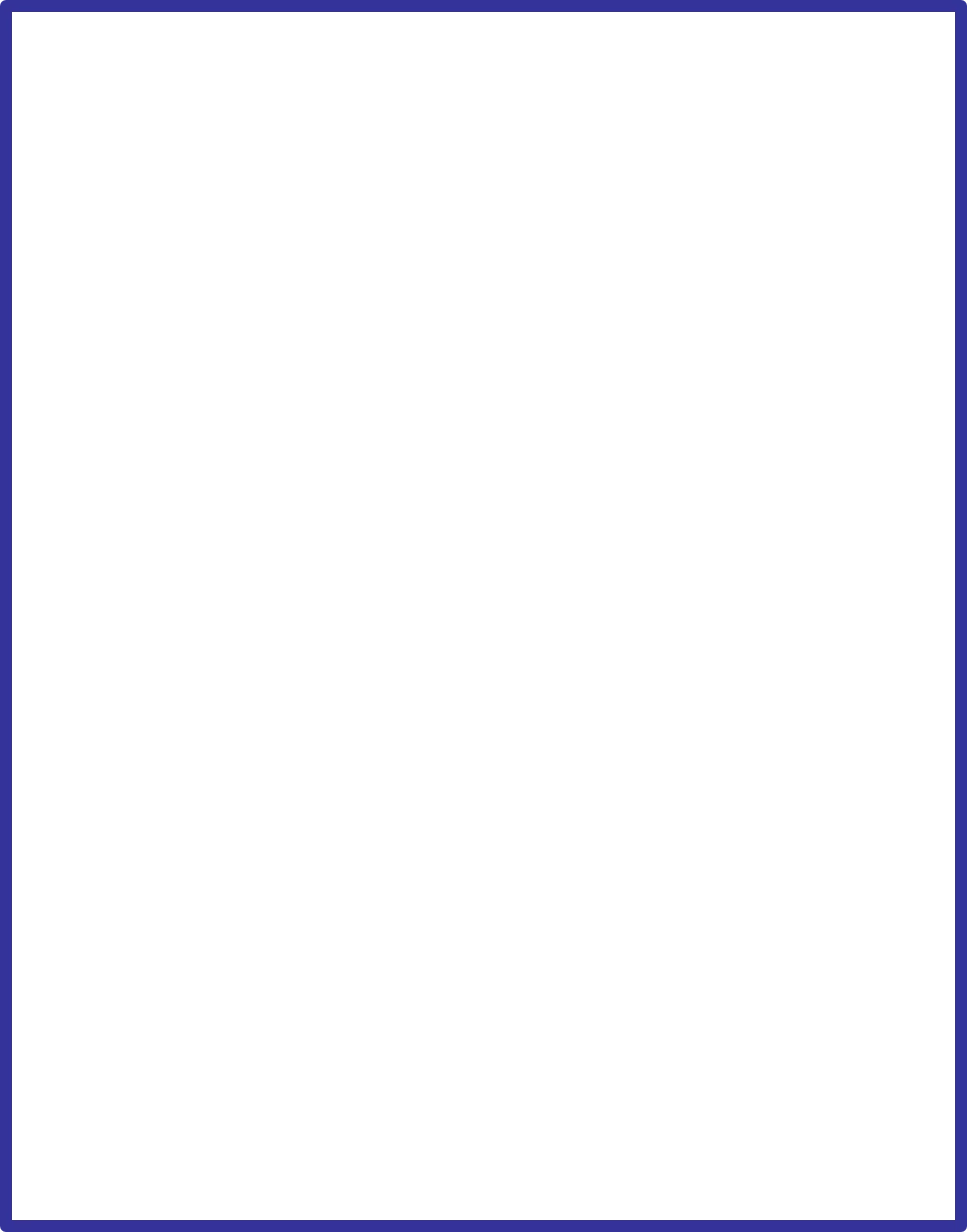
UCSC-1 QUASIPARTICLESIN NORMAL PHASE Landau (1956), Nozi res, Theory of Interacting Fermi Systems (1964): Start from noninteracting Fermi gas, then energy eigenstates specified by ? ?,? ,? ?,? = 0 or 1 groundstate has ? ?,? = ?? ? ,??= 3?2?1/3 switch on inter-particle interaction ? adiabatically: ? < 0,? 0 ? ? = 0 = ? ? ? = ?exp ?? provided perturbation theory converges, states of fully interacting system can be labelled by the noninteracting states ?0?,? from which they evolved. ( adiabatic evolution) then define no. of quasiparticles in state ?,? n ?,? as simply equal to n0?,? ( Luttinger theorem trivial) (Actually, adiabaticity is sufficient but not necessary (S. Shastry, Ann. Phys. 405, 155 (2019)) Suppose ? = +??,? ? ?? ??? ?? ? = then in original noninteracting system ? ?? ?0?,? . ?? ? = Is it true that in fully interacting system also ? ?? ? ?,? ? ?? Answer: yes, if and only if ?, ? ? = 0
UCSC-2 ?, ? ? What quantities are conserved = 0 ? (a) liquid 3??: ? = +??? total number ??? yes ?? ? +??? total spin ???? yes ?? ? = ? 1 +??? total current ???? yes ?? ?? ? 1 +??? total spin current ?? ??? no ?? in real liquid 3??, ? = ?? ?? (etc.) ?? ? ??? ?? ?? but ?? (b) metallic system (e.g. cuprates) ?,? conserved but ? not conserved (even after transformation to Bloch states, because of U-processes).
UCSC-3 Consequences of conservation for response functions Consider ????,? ?? of ? 0,0 ? ?? If ? is conserved, then in limit ? 0, support of ??????? comes entirely from quasiparticle states and is limited to ? v?? Ex: ? = ? (density response function) of liquid 3?? ? = v?? ??????? zero-sound peak v?? ? If ? is not conserved, e.g. ? = ?? in 3??, quasiparticle states do not exhaust sum rule for ????? and even in limit k 0 get incoherent background. extends to v?? ??????? v?? ? In liquid 3??, can infer quasiparticle contribution to ????? from Landau parameter ??1 (measurable in spin-echo experiments). Conclusion: In 3??, incoherent background contributes >80% of sum rule!
UCSC-4 Moral: Even if system is a decent Fermi liquid, correlation function of non-conserved quantity can have large contribution from incoherent background. Application to cuprates (and maybe other SCES): ?,? conserved but ? not conserved (because of U-processes) sum rule for ??????? large contribtion from incoherent background (MIR peak). = ?? ? ? can have Are the optimally doped and underdoped cuprates simply bad Fermi liquids? (cf. e.g. Berthod et al., PR B 87, 115109 (2013)).
UCSC-5 QUASIPARTICLES IN SUPERFLUID STATE 1. BCS CASE: MEAN-FIELDTREATMENT breaks U(1) symmetry ???= ? ? = ? ? ? +?? + ? ? + +? ? + ? ? = ???? ? ? + ??? + ?.?. 2?2 2? ? to be determined self-consistently Hilbert space of ? is 4D: |1 1 |0 0 , |0 1 , |1 0 , Ground pair state: ??= ?? 1 +? ? + ? |0 0 + ?? 1 +?? ?? |1 1 ??+ ???? 1 1 ?? ?? |vac ?? ,?? , even no. parity 2 2 12 2+ ? 2 ?? ?? Excited-pair state: ??= ?? ? |0 0 ?? |1 1 ,??? ???= 2??
UCSC-6 Odd-no-parity states (Bogoliubov quasiparticles): Standard textbook maneuver: solve ???, = ? Bogoliubov quasiparticle creation operator +,? ? + with lin. combination of ?? ,?? ,? ? result: 2 solutions with E = +??, e.g. ?= ???? ?? ?? + + ??? ? ?? | ? ?? | ? |1 0 2 solutions with ? = ??, e.g. = ???? ?? ?? ++ ??? ? + ? | ? ?? | ? vector of zero norm However, 0, i.e. ?? + is pure annihilator ? + is H.C. of second Bogoliubov + . This is obvious since ?? quasiparticle creation operator ? ? (note: ?, | ??=0 for any ?!) | ?? = ? | ?? is compatible with
UCSC-7 SOME PROPERTIES OF BOGOLIUBOV QUASIPARTICLES 1. reflection by barrier 0 0 ?? ? ? e.g.: > ? ? ?? 1 energy relative to Fermi energy Incident particle, 0 < ??< 0 for normal reflection one needs ?~2?? so amplitude ~exp 2??? 1, and Fermi sea blocked:?? Quasiclassical discussion: ? = ? ?,? , 12 2+ ? 2 ? ?,? ?? ?? ??= ?? ?? ??=1 ?? ?? v? ?,?, ??? ?? 0= ? ,??= 0 ? falls through Fermi at point ?? surface particle reflected as hole (Andreev reflection) by conservation of energy, far from barrier ??= ?? hole energy is ??.
UCSC-8 What is momentum transfer in Andreev reflection? ??~ ??? ?? ? =2?? ?? ?? (normal incidence) Is there direct experimental evidence for this? Yes! Buchanan et al., (PRL 57, 341 (1986) measure terminal velocity of 3?? ? ? interface. frictional force due to reflection of qps ?????= ???/ If we assume reflection is normal , ????? 1mm/sec Experimentally, ?????~ 0.1 1m/sec Andreev reflection (S-K Yip and AJL, PRL 57, 345 (1986))
UCSC-9 2. The Zeeman-dimple problem Note: spatial variation of gap ? not a necessary condition for AR! Andreev reflection Can alternatively result from spatial variation of diagonal potential ? ? , provided this is the same for particle and hole (e.g. Zeeman potential ???? ? ) Ex*: neutral Fermi superfluid with Zeeman coupling to external field ? ?with dimple ?0 /?? ? ? ? ?0 Even (number) parity ground state 0 has ? = ????? to linear order in ?. What is nature of lowest-energy odd-parity state? Answer: Single Bogoliubov quasiparticle trapped in dimple . Extra spin localized in/close to dimple = 1. *Y.-R. Lin and AJL, JETP 119, 1034 (2014)
UCSC-10 What is extra charge? particle hole In quasiclassical approximation with only Andreev reflection: ? ???= ????????? ? ???= ????????? but in formula + ??? ? + ???= ???? | 0 1 1 ??= 1 + ??/??,??= 1 ??/?? 2 2 so?? ?? ?? ?. Also, velocityk= 1??? ??? ???/?? , so to the extent that N -state = 1??/?? spectrum is particle-hole symmetric, ???/?? = ??= ?????. , extra charge = 0 but this result is not robust .
UCSC-11 Further complication: in this approximation, ground state of odd-number-parity sector is doublet related by time reversal! hole particle T particle hole Normal (non-Andreev) reflection splits doublet into even and odd combinations with exponentially small splitting. However, this does not change situation with regard to C-symmetry. zero extra charge is not robust. (even in quasiclassical approximation) Another interesting question: if geometry is that of torus and the dimple is dragged once around it, what is resultant Berry phase (a) if superfluid is stationary (b) if it is moving with ??= 2??( Abrikosov vortex )?
UCSC-12 BEYOND BCS In original BCS case, simple relation between even- number-parity states (Cooper pairs) and odd-number- parity ones (Bogoliubov quasiparticles). In the more general case this is no longer so. Generic ansatz for (particle-conserving) completely paired GS of even-N system: ?/2 ? ?? ? ?= ? normalization ???? ??? ?,? ?? |vac ?? antisymmetric By standard theorem (Yang 1962) can always rewrite in form ?/2 + +? ? ?= ? ???? |vac ? with ? , ? nonintersecting complete half-sets. If we break U(1) symmetry as in BCS, then even-parity GS is of form even= ? ?? ?. ??= ??+ ???? + +? ? ? |vac (similar to BCS with ? ?, ? ?
UCSC-13 Also similarly to BCS, we can say + +? ? (a) Excited pair state is ?? ???? |vac ++ ??? ?, ??? ? + ???? are (b) Operators v??? pure annihilators However: the obvious guess at a Bogoliubov quasiparticle operator, namely += ???? + ???? ?? indeed generates a state state, but |1 0 which is an odd parity this state is in general not an eigenstate of the Hamiltonian! In fact, we need to write the Bogoliubov quasiparticle states in form odd,?= ??? |1 0?+ ??? |0 1? ? ?? + ++ ???? ? ????? | ? ? with ???,??? fixed by minimizing MF Hamiltonian.
UCSC-14 Bogoliubov-de Gennes In coordinate representation, mean-field (BdG) Hamiltonian is schematically of form ???= ? ??? +1 ? ?? ? + ?? 2 ???? ???,? ?? ?????? ?? ?? ? : bilinear in ??? ,?? (with a term ???? included in ???? to fix average particle number ? .) In our context, interesting problem is to find simplest fermionic (odd-parity) states ( Bogoliubov quasiparticles ). For this purpose write schematically ?= ?? + even, where (ignoring (real) spin degree of freedom) ? ? ? ? Nambu spinor = ??? ? ? + ??? ? ? ?? ?? and determine the coefficients ??? ,??? by solving the Bogoliubov-de Gennes equations = ?? ?? ???, ?? so that ??+ const. ???= ???? ?
UCSC-15 (All this is standard textbook stuff ) Note crucial point: In mean-field treatment, fermionic quasiparticles are quantum superpositions of particle and hole do not correspond to definite particle number (justified by appeal to SBU(1)S).* This particle-hole mixing is sometimes (misleadingly) regarded as analogous to the mixing of different bands in an insulator by spin-orbit coupling. (hence, analogy topological insulator topological superconductor.) Another note: just as in simple BCS case, negative- energy solutions of the BdG equations are fictitious (they simply correspond to operations which annihilate the GS). *spontaneously broken U(1) symmetry
UCSC-16 Majorana fermions operators Recap: fermionic (Bogoliubov) quasiparticles created by = ?? ??? ? ? + ??? ? ? ?? with the coefficients ??? ,??? given by solution of the BdG equations ???,?? = ???? Question: Do there exist solutions of the BdG equations such that ?? (and thus ??= 0)? =?? This requires (at least) 1. Spin structure of ? ? ,? ? the same pairing of parallel spins (spinless or spin triplet, not BCS s-wave) 2. ? ? = ? ? 3. interesting structure of ???,? , e.g. ? + ?? ?,? ?,? ~ ? ??+ ???
UCSC-17 Case of particular interest: half-quantum vortices (HQV s) in Sr2RuO4 (widely believed to be ? + ?? superconductor). In this case a M.F. predicted to occur in (say) component, (which sustains vortex), not in (which does not). Not that vortices always come in pairs (or second MF solution exists on boundary) computing? Why the special interest for topological quantum (1) Because MF is exactly equal superposition of particle and hole, it should be undetectable by any local probe. (2) MF s should behave under braiding as Ising anyons*: if 2 HQV s, each carrying a M.F., interchanged, phase of MBWF changed by /2 (note not as for real fermions!) So in principle : (1) create pairs of HQV s with and without MF s (2) braid adiabatically (3) recombine and measure result (partially) topologically protected quantum computer! * D. A. Ivanov, PRL 86, 268 (2001) Stone & Chung, Phys. Rev. B 73, 014505 (2006)
UCSC-18 Comments on Majarama fermions (within the standard mean-field approach) (1) What is a M.F. anyway? Recall: it has energy exactly zero, that is its creation operator ?? satisfies the equation = 0 ?,?? But this equation has two possible interpretations: creates a fermionic quasiparticle with exactly zero energy (i.e. the odd- and even-number-parity GS s are exactly degenerate) (a) ?? annihilates the (even-parity) groundstate ( pure annihilator ) (b) ?? However, it is easy to show that in neither case do we have ?? (a) and (b), i.e. = ??. To get this we must superpose the cases superposition of a real Bogoliubov quasiparticle and a pure annihilator. a Majarana fermion is simply a quantum
UCSC-19 But Majorana solutions always come in pairs by superposing two MF s we can make a real zero- energy fermionic quasiparticle HQV 1 HQV 2 ?1 ?2 + ??2 ? ?1 Bog. qp. The curious point: the extra fermion is split between two regions which may be arbitrarily far apart! (hence, usefulness for TQC) topological quantum computing Thus, e.g. interchange of 2 vortices each carrying an MF rotation of zero-energy fermion by . (note predicted behavior (phase change of /2) is average of usual symmetric (0) and antisymmetric ( ) states)
TQ-7.9 TQ-7.9 TQ-7.9 However, we are still missing one DB creation operator and one pureannihilator. Clearly thesehavetobeassociated with the miss- ing link (n 1)-0. In fact, consider 0 1 2 This may be verified explicitly to create an (N + 1)-particleenergy eigenstatewhichisde- generate with thegroundstate. Thecorresponding pureannihilator is 0 1 a However, we are still missing one DB creation operator and one pureannihilator. Clearly thesehavetobeassociated with the miss- ing link (n 1)-0. In fact, consider 0 1 2 This may be verified explicitly to create an (N + 1)-particleenergy eigenstatewhichisde- generate with thegroundstate. Thecorresponding pureannihilator is a 2 If now weconsider theoperators 1 2 Mn 2 thesegenerateM ajorana fermions localized on sitesn 1and 0 thesegenerateM ajorana fermions localized on sitesn 1and 0 However, we are still missing one DB creation operator and one pureannihilator. Clearly thesehavetobeassociated with the miss- ing link (n 1)-0. In fact, consider 0 1 2 This may be verified explicitly to create an (N + 1)-particleenergy eigenstatewhichisde- generate with thegroundstate. Thecorresponding pureannihilator is 0 1 a 2 If now weconsider theoperators 1 2 Mn 0 = 0 0 0 1 1 1 a a a a a a n 1 n 1 n 1 n 1+ ian 1 + 0 ia0 n 1+ ian 1 + 0 ia0 n 1+ ian 1 + 0 ia0 2 2 2 UCSC-20 An intuitive way of generating MF s in the KQW: Kitaev quantum wire 0 1 2 If now weconsider theoperators 1 2 Mn 2 thesegenerateM ajorana fermions localized on sitesn 1and 0 separately. separately. separately. a a a n 1+ ian 1 0 ia0 n 1+ ian 1 0 ia0 n 1+ ian 1 0 ia0 For this problem, fermionic excitations have form M0 0+ = 1 1 1 M0 M0 0+ = 0+ = = ?? 1 2 a a a n 1+ ian 1 n 1+ ian 1 + ??? 1 0 ia0 2 1 2 2 1 2 n 1+ ian 1 2 1 2 + ??? + ?? 1 ?? 1 1 0 = 0 = a a a 0 ia0 0 ia0 so localized on links not sites. Energy for link ?,? 1 is ?? K itaev quantum wire K itaev quantum wire K itaev quantum wire ?? 0 n 1 An intuitiveway of generating MF sin theKQW: An intuitiveway of generating MF sin theKQW: ? 1 ? An intuitiveway of generating MF sin theKQW: ? 1 ? n 1 n 1 0 0 0 Xj Xj Xj ?? X0 0 X0 0 X0 0 0 0 n 1 n 1 n 1 M F2 0 M F1 M F1 M F1 M F2 M F2 [ Variationson KQW T-junctionsetc. ] [ Variationson KQW T-junctionsetc. ] [ Variationson KQW T-junctionsetc. ]
UCSC-21 Majorana fermions: beyond the mean-field approach Problem: The whole apparatus of mean-field theory rests fundamentally on the notion of SBU(1)S spontaneously broken U(1) gauge symmetry: ??~ ?????? even~ ?? ? ?= even | odd~ ?? ? ? ? ? + ? ? ? ? | even ?? even But in real life condensed-matter physics, SB U(1)S IS A MYTH!! This doesn t matter for the even-parity GS, because of Anderson trick : 2N~ even? exp ????? But for odd-parity states equation ( * ) is fatal! Examples: (1) Galilean invariance (2) NMR of surface MF in 3He-B
UCSC-22 We must replace ( * ) by creates extra Cooper pairs = ?? ? ? ? ? + ? ? ?? ?? This doesn t matter, so long as Cooper pairs have no interesting properties (momentum, angular momentum, partial localization...) But to generate MF s, pairs must have interesting properties! doesn t change arguments about existence of MF s, but completely changes arguments about their braiding, undetectability etc. Need completely new approach!