Understanding Polygon Angle-Sum Theorems

Explore the Polygon Angle-Sum Theorems that determine the sum of interior angle measures in polygons. Learn about the Polygon Angle-Sum Theorem, number of sides in polygons, finding angle sums, and the corollary for regular polygons. Practice using the theorems to calculate interior angle measures in polygons like heptagons, 17-gons, and regular nonagons. Delve into real-world applications like determining angle measures in common housefly eyes with hexagonal facets. Enhance your knowledge of geometry with these theorem applications and problem-solving exercises.

• Polygon Angle-Sum Theorems
• Interior Angle Measures
• Polygons
• Geometry
• Problem-Solving

nash_ler Follow

Uploaded on Oct 01, 2024 | 0 Views

Download Presentation

Please find below an Image/Link to download the presentation.

The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.

Presentation Transcript

1. 6-1 The Polygon Angle-Sum Theorems

2. Polygon Angle-Sum Theorem The sum of the measures of the interior angles of a n-gon is ? 2 180

3. NUMBER OF SIDES NAME 3 Triangle 4 Quadrilateral 5 Pentagon 6 Hexagon 7 Heptagon 8 Octagon 9 Nonagon 10 Decagon

4. Problem 1: Finding a Polygon Angle Sum What is the sum of the interior angle measures of a heptagon?

5. What is the sum of the interior angle measures of a 17-gon?

6. The sum of the interior angle measures of a polygon is 1980. How can you find the number of sides in the polygon?

10. Corollary to the Polygon Angle-Sum Theorem The measure of each interior angle of a regular n-gon is ? 2 180 ?

11. Problem 2: Using the Polygon Angle-Sum Theorem The common housefly has eyes that consist of approximately 4000 facets. Each facet is a regular hexagon. What is the measure of each interior angle in on hexagonal facet?

12. What is the measure of each interior angle in a regular nonagon?

13. Problem 3: Using the Polygon Angle-Sum Theorem

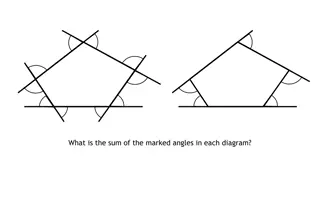
15. You can draw exterior angles at any vertex of a polygon. The figures below show that the sum of the measures of the exterior angles, one at each vertex is 360.

16. Polygon Exterior Angle-Sum Theorem The sum of the measures of the exterior angles of a polygon, one at each vertex, is 360. For the pentagon

17. Problem 4: Finding an Exterior Angle Measure

18. What is the measure of an exterior angle of a regular nonagon?